Chapter 3.2, Problem 22AYU

(a)

To determine

To check: The ordered pair $\left(A,S\right)$ represent a function.

Answer to Problem 22AYU

Not function

Explanation of Solution

Given: A marketing firm wishes to find a function that relates the sales S of a product and A , the amount spent on advertising the product. The data are obtained from past experience. Advertising and sales are measured in thousands of dollars.

  $\begin{array}{cc}\text{Advertising Expenditures,}A& \text{Sales, }S\\ 20& 335\\ 22& 339\\ 22.5& 338\\ 24& 343\\ 24& 341\\ 27& 350\\ 28.3& 351\end{array}$

The ordered pair $\left(A,S\right)$ represents the above table.

So, Independent variable is $A$ and dependent variable is $S$ .

From table, Two same input get two different output.

Therefore, above table doesn’t represent function.

(b)

To determine

To draw: The scatter diagram of the table.

Answer to Problem 22AYU

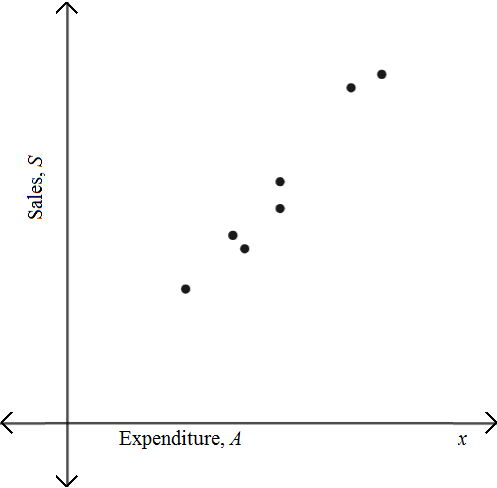
Explanation of Solution

Given: A marketing firm wishes to find a function that relates the sales S of a product and A , the amount spent on advertising the product. The data are obtained from past experience. Advertising and sales are measured in thousands of dollars.

  $\begin{array}{cc}\text{Advertising Expenditures,}A& \text{Sales, }S\\ 20& 335\\ 22& 339\\ 22.5& 338\\ 24& 343\\ 24& 341\\ 27& 350\\ 28.3& 351\end{array}$

Using graphing utility to draw scatter plot.

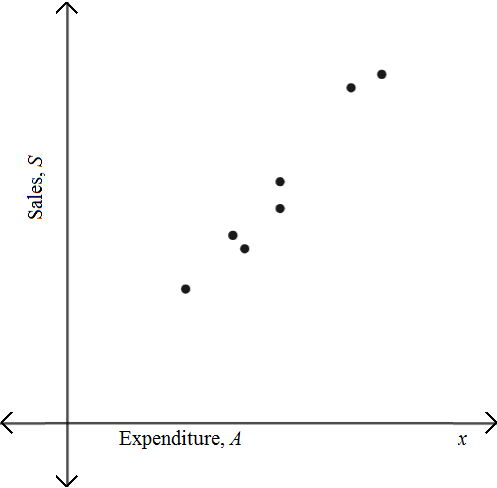
(c)

To determine

To find: The line of best fit that models the relation between expenditures and sales.

Answer to Problem 22AYU

  $y=2.067x+292.89$

Explanation of Solution

Given: A marketing firm wishes to find a function that relates the sales S of a product and A , the amount spent on advertising the product. The data are obtained from past experience. Advertising and sales are measured in thousands of dollars.

  $\begin{array}{cc}\text{Advertising Expenditures,}A& \text{Sales, }S\\ 20& 335\\ 22& 339\\ 22.5& 338\\ 24& 343\\ 24& 341\\ 27& 350\\ 28.3& 351\end{array}$

Using graphing utility, the equation of line of best fit.

  $y=2.067x+292.89$

(d)

To determine

To find: The slope of line of best fit.

Answer to Problem 22AYU

  $\text{Slope}=2.067$

Explanation of Solution

Given: A marketing firm wishes to find a function that relates the sales S of a product and A , the amount spent on advertising the product. The data are obtained from past experience. Advertising and sales are measured in thousands of dollars.

  $\begin{array}{cc}\text{Advertising Expenditures,}A& \text{Sales, }S\\ 20& 335\\ 22& 339\\ 22.5& 338\\ 24& 343\\ 24& 341\\ 27& 350\\ 28.3& 351\end{array}$

Using graphing utility, the equation of line of best fit.

  $y=2.067x+292.89$

Slope is coefficient of x.

Therefore, the slope is 2.067

(e)

To determine

To express: The relationship from part (c) using function notation.

Answer to Problem 22AYU

  $S=2.067A+292.89$

Explanation of Solution

Given: A marketing firm wishes to find a function that relates the sales S of a product and A , the amount spent on advertising the product. The data are obtained from past experience. Advertising and sales are measured in thousands of dollars.

  $\begin{array}{cc}\text{Advertising Expenditures,}A& \text{Sales, }S\\ 20& 335\\ 22& 339\\ 22.5& 338\\ 24& 343\\ 24& 341\\ 27& 350\\ 28.3& 351\end{array}$

Using graphing utility, the equation of line of best fit.

  $y=2.067x+292.89$

Now, write as function form.

Therefore, $S=2.067A+292.89$

Where, A represents expenditure and S represent sales.

(f)

To determine

To find: The domain of function.

Answer to Problem 22AYU

  $D\in \left(0,\infty \right)$

Explanation of Solution

Given: A marketing firm wishes to find a function that relates the sales S of a product and A , the amount spent on advertising the product. The data are obtained from past experience. Advertising and sales are measured in thousands of dollars.

  $\begin{array}{cc}\text{Advertising Expenditures,}A& \text{Sales, }S\\ 20& 335\\ 22& 339\\ 22.5& 338\\ 24& 343\\ 24& 341\\ 27& 350\\ 28.3& 351\end{array}$

Using graphing utility, the equation of line of best fit.

  $y=2.067x+292.89$

Now, write as function form.

Therefore, $S=2.067A+292.89$

Where, A represents expenditure and S represent sales.

The domain depends on the input value of A .

  $D\in \left(0,\infty \right)$

(g)

To determine

To predict: The sales for expenditures are $25,000.

Answer to Problem 22AYU

  $\$34457$

Explanation of Solution

Given: A marketing firm wishes to find a function that relates the sales S of a product and A , the amount spent on advertising the product. The data are obtained from past experience. Advertising and sales are measured in thousands of dollars.

  $\begin{array}{cc}\text{Advertising Expenditures,}A& \text{Sales, }S\\ 20& 335\\ 22& 339\\ 22.5& 338\\ 24& 343\\ 24& 341\\ 27& 350\\ 28.3& 351\end{array}$

Using graphing utility, the equation of line of best fit.

  $y=2.067x+292.89$

Now, write as function form.

Therefore, $S=2.067A+292.89$

Where, A represents expenditure and S represent sales.

Put $A=25$ into $S=2.067A+292.89$

  $\begin{array}{l}S=2.067\left(25\right)+292.89\\ S=\$344.57\end{array}$

Hence, the sales of $34457.