Chapter 3.4, Problem 11AYU

(a)

To determine

To find: the horizontal distance from the face of the cliff

Answer to Problem 11AYU

Therefore, the height of the projectile will be the maximum at a horizontal distance of $39\text{ft}$ approximately.

Explanation of Solution

Given:

  $h(x)=\frac{-32x^2}{{(50)}^2}+x+200$

Calculation:

Simplify the function for the height of the projectile.

  $h(x)=\frac{-32x^2}{{(50)}^2}+x+200=\frac{-32}{2500}{x}^2+x+200=\frac{-8}{625}{x}^2+x+200$

Compare $h(x)$ with the standard quadratic equation.

  $a=\frac{-8}{625},b=1$ and $c=200$

Since $a<0,$ the maximum value of $h$ will be at the vertex.

Thus,

  $\frac{-b}{2a}=\frac{-1}{2\left(\frac{-8}{625}\right)}=\frac{625}{16}=39\text{ft}$

Therefore, the height of the projectile will be the maximum at a horizontal distance of $39\text{ft}$ , approximately.

Conclusion:

Therefore, the height of the projectile will be the maximum at a horizontal distance of $39\text{ft}$ , approximately.

(b)

To determine

To find: the maximum height of the projectile.

Answer to Problem 11AYU

Therefore, the maximum height of the projectile is $219.5\text{ft},$

Explanation of Solution

Calculation:

Substitute $\frac{625}{16}$ for $x$ in $h(x)=\frac{-8}{625}{x}^2+x+200$ to find the maximum height of theprojectile,

  $h=\frac{-8}{625}{\left(\frac{625}{16}\right)}^2+\frac{625}{16}+200=\frac{-8}{1}\left(\frac{625}{256}\right)+\frac{625}{16}+200=\frac{-625}{32}+\frac{625}{16}+200$

Simplify by multiplying the numerator and the denominator by the least common multiple of the denominators, 32

  $h=\frac{-625}{32}+\frac{1250}{32}+\frac{6400}{32}=\frac{-625+1250+6400}{32}=\frac{7025}{32}=219.5\text{ft}$

Therefore, the maximum height of the projectile is $219.5\text{ft},$

Conclusion:

Therefore, the maximum height of the projectile is $219.5\text{ft},$

(c)

To determine

To find:horizontal distancethat the projectile will strike the water

Answer to Problem 11AYU

Therefore, the projectile will strike the water at a horizontal distance of $170\text{ft}$ from the face ofthe cliff.

Explanation of Solution

Calculation:

When the projectile strikes the water, the height will be 0 .

Thus,

  $\frac{-8}{625}{x}^2+x+200=0$

The equation is in the form of the standard quadratic equation.

Find $b^2-4ac$ first

  $b^2-4ac=1^2-4\left(\frac{-8}{625}\right)(200)=1+\frac{256}{25}=11.24$

Find the value of $x$ .

  $\begin{array}{l}x=\frac{-b\pm \sqrt{b^2-4ac}}{2a}\\ =\frac{-1\pm \sqrt{11.24}}{2\left(\frac{-8}{625}\right)}\\ =\frac{-1\pm 3.352}{-0.0256}\end{array}$

  $=\frac{-1-3.352}{-0.0256}$ or $\frac{-1+3.352}{-0.07}$

  $=170$ or -91.8

Since the distance cannot be negative, discard -91.8

Therefore, the projectile will strike the water at a horizontal distance of $170\text{ft}$ from the face ofthe cliff.

Conclusion:

Therefore, the projectile will strike the water at a horizontal distance of $170\text{ft}$ from the face ofthe cliff.

(d)

To determine

To graph: graph the function by using a graphing utility

Answer to Problem 11AYU

  

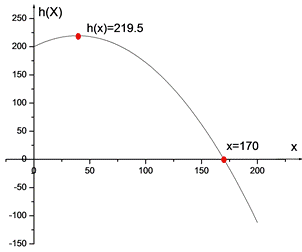
Explanation of Solution

Calculation:

Following is the graph of the function $\text{h},0\le x\le 200$

  

Conclusion:

Thus, the required graph is drawn.

(e)

To determine

To verify: the solutions found in parts (b) and (c).

Answer to Problem 11AYU

From the graph also the maximum height of the projectile is $219.5\text{ft}$ and the horizontal distancewhere the projectile strike the water is $170\text{ft}$ .

Explanation of Solution

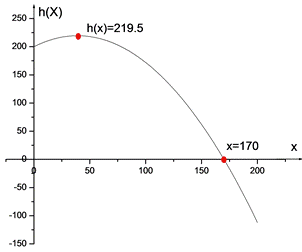
Calculation:

Following is the graph of the function $\text{h},0\le x\le 200$

  

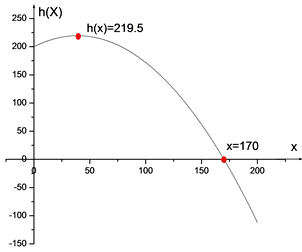
From the graph also the maximum height of the projectile is $219.5\text{ft}$ and the horizontal distancewhere the projectile strike the water is $170\text{ft}$

Conclusion:

From the graph also the maximum height of the projectile is $219.5\text{ft}$ and the horizontal distancewhere the projectile strike the water is $170\text{ft}$

(f)

To determine

To find:the distance of the projectile from the cliff

Answer to Problem 11AYU

Therefore, when the height of the projectile is $100\text{ft},$ the projectile is at a distance of $135.7\text{ft}$ from the cliff.

Explanation of Solution

Calculation:

Substitute $100\text{ft}$ for $h$ in $h(x)=\frac{-8}{625}{x}^2+x+200$

  $100=\frac{-8}{625}{x}^2+x+200$

Swap the sides of the equation.

  $\frac{-8}{625}{x}^2+x+200=100$

Subtract 100 from both the sides.

  $\begin{array}{l}\frac{-8}{625}{x}^2+x+200-100=100-100\hfill \\ \frac{-8}{625}{x}^2+x+100=0\hfill \end{array}$

Compare the equation with the standard form of the quadratic equation.

  $a=\frac{-8}{625},b=1$ and $c=100$

Find $b^2-4ac$ first.

  $b^2-4ac=1^2-4\left(\frac{-8}{625}\right)(100)=1+\frac{128}{25}=6.12$

Find the value of $x$

  $\begin{array}{l}x=\frac{-b\pm \sqrt{b^2-4ac}}{2a}\\ =\frac{-1\pm \sqrt{6.12}}{2\left(\frac{-8}{625}\right)}\\ =\frac{-1\pm 2.4739}{-0.0256}\end{array}$

  $=\frac{-1-2.4739}{-0.0256}$ or $\frac{-1+2.4739}{-0.07}$

  $=135.7$

or -57.57

Since the distance cannot be negative, discard -57.57

Therefore, when the height of the projectile is $100\text{ft},$ the projectile is at a distance of $135.7\text{ft}$ from the cliff

Conclusion:

Therefore, when the height of the projectile is $100\text{ft},$ the projectile is at a distance of $135.7\text{ft}$ from the cliff