Fundamentals of Heat and Mass Transfer
7th Edition
ISBN: 9780470917855
Author: Bergman, Theodore L./
Publisher: John Wiley & Sons Inc
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 4, Problem 4.56P
Functionally graded materials are intentionally fabricated to establish a spatial distribution of properties in the final product. Consider an
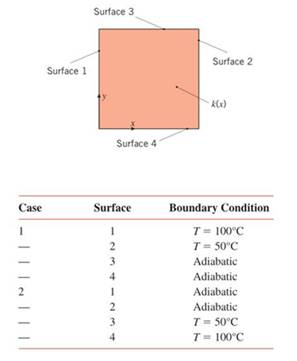
- Determine the spatially averaged value of the thermal conductivity k. Use this value to estimate the heat rate per unit length for cases I and 2.
- Using a grid spacing of 2 mm. determine the heat rate per unit depth for case 1. Compare your result to the estimated value calculated in part (a).
- Using a grid spacing of 2 mm, determine the heat rate per unit depth for case 2. Compare your result to the estimated value calculated in part (a).
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
Solve using the methodology : Known, Find, Schematic Diagram, Assumptions, Properties, Analysis and Comments.
please provide answers with step-by-step calculations and explanation
question A B and C
Chapter 4 Solutions
Fundamentals of Heat and Mass Transfer
Ch. 4 - In the method of separation of variables (Section...Ch. 4 - A two-dimensional rectangular plate is subjected...Ch. 4 - Consider the two-dimensional rectangular plate of...Ch. 4 - A two-dimensional rectangular plate is subjected...Ch. 4 - A two-dimensional rectangular plate is subjected...Ch. 4 - Using the thermal resistance relations developed...Ch. 4 - Free convection heat transfer is sometimes...Ch. 4 - Consider Problem 4.5 for the case where the plate...Ch. 4 - Prob. 4.9PCh. 4 - Based on the dimensionless conduction heat rates...
Ch. 4 - Determine the heat transfer rate between two...Ch. 4 - A two-dimensional object is subjected to...Ch. 4 - An electrical heater 100 mm long and 5 mm in...Ch. 4 - Two parallel pipelines spaced 0.5 m apart are...Ch. 4 - A small water droplet of diameter D=100m and...Ch. 4 - A tube of diameter 50 mm having a surface...Ch. 4 - Pressurized steam at 450K flows through a long,...Ch. 4 - The temperature distribution in laser-irradiated...Ch. 4 - Hot water at 85°C flows through a thin-walled...Ch. 4 - A furnace of cubical shape, with external...Ch. 4 - Laser beams are used to thermally process...Ch. 4 - A double-glazed window consists of two sheets of...Ch. 4 - A pipeline, used for the transport of crude oil,...Ch. 4 - A long power transmission cable is buried at a...Ch. 4 - A small device is used to measure the surface...Ch. 4 - A cubical glass melting furnace has exterior...Ch. 4 - An aluminum heat sink (k=240W/mK), used to cool an...Ch. 4 - Hot water is transported from a cogeneration power...Ch. 4 - A long constantan wire of 1-mm diameter is butt...Ch. 4 - A hole of diameter D=0.25m is drilled through the...Ch. 4 - In Chapter 3 we that, whenever fins are attached...Ch. 4 - An igloo is built in the shape of a hemisphere,...Ch. 4 - Prob. 4.34PCh. 4 - An electronic device, in the form of a disk 20 mm...Ch. 4 - The elemental unit of an air heater consists of a...Ch. 4 - Prob. 4.37PCh. 4 - Prob. 4.38PCh. 4 - Prob. 4.39PCh. 4 - Prob. 4.40PCh. 4 - One of the strengths of numerical methods is their...Ch. 4 - Determine expressionsfor...Ch. 4 - Consider heat transfer in a one-dimensional...Ch. 4 - In a two-dimensional cylindrical configuration,...Ch. 4 - Upper and lower surfaces of a bus bar are...Ch. 4 - Derive the nodal finite-difference equations for...Ch. 4 - Consider the nodal point 0 located on the boundary...Ch. 4 - Prob. 4.48PCh. 4 - Prob. 4.49PCh. 4 - Consider the network for a two-dimensional system...Ch. 4 - An ancient myth describes how a wooden ship was...Ch. 4 - Consider the square channel shown in the sketch...Ch. 4 - A long conducting rod of rectangular cross section...Ch. 4 - A flue passing hot exhaust gases has a square...Ch. 4 - Steady-state temperatures (K) at three nodal...Ch. 4 - Functionally graded materials are intentionally...Ch. 4 - Steady-state temperatures at selected nodal points...Ch. 4 - Consider an aluminum heat sink (k=240W/mK), such...Ch. 4 - Conduction within relatively complex geometries...Ch. 4 - Prob. 4.60PCh. 4 - The steady-state temperatures (°C) associated with...Ch. 4 - A steady-state, finite-difference analysis has...Ch. 4 - Prob. 4.63PCh. 4 - Prob. 4.64PCh. 4 - Consider a two-dimensional. straight triangular...Ch. 4 - A common arrangement for heating a large surface...Ch. 4 - A long, solid cylinder of diameter D=25mm is...Ch. 4 - Consider Problem 4.69. An engineer desires to...Ch. 4 - Prob. 4.71PCh. 4 - Prob. 4.72PCh. 4 - Prob. 4.73PCh. 4 - Refer to the two-dimensional rectangular plate of...Ch. 4 - The shape factor for conduction through the edge...Ch. 4 - Prob. 4.77PCh. 4 - A simplified representation for cooling in very...Ch. 4 - Prob. 4.84PCh. 4 - A long trapezoidal bar is subjected to uniform...Ch. 4 - Consider the system of Problem 4.54. The interior...Ch. 4 - A long furnace. constructed from refractory brick...Ch. 4 - A hot pipe is embedded eccentrically as shown in a...Ch. 4 - A hot liquid flows along a V-groove in a solid...Ch. 4 - Prob. 4S.5PCh. 4 - Hollow prismatic bars fabricated from plain carbon...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- The first part of the question has been worked on, where the heat transfer was calculated to be 6.770 kilowatt, but the problem also asks to calculate the temperature of the outer most surface. What is the temperature of the outer most surface?arrow_forwardA team of students tests a material for its thermal conductivity (k). After 20 minutes in a heat box, the temperature is 48° C inside the box and 28° C on top of the material. The following data is true about this test: Area of material = .0225 m2 Thickness of material = .0127 m Light bulb = 25 W What is the thermal conductivity constant for the material? Calculate the amount of energy transferred through the material. Determine the R-value of the material. Based on your calculations, would the material be a reasonable choice for home insulation? Yes of Noarrow_forwardX Your answer is incorrect. A dormitory at a large university, built 50 years ago, has exterior walls constructed of L, = 25-mm-thick sheathing with a thermal conductivity of k, = 0.1 W/m-K. To reduce heat losses in the winter, the university decides to encapsulate the entire dormitory by applying an L; = 25-mm-thick layer of extruded insulation characterized by k; = 0.029 W/m-K to the exterior of the original sheathing. The extruded insulation is, in turn, covered with an Lg = 5-mm-thick architectural glass with kg = 1.4 W/m-K. Determine the heat flux through the original and retrofitted walls when the interior and exterior air temperatures are T = 22°C and To = O°C, respectively. The inner and outer convection heat transfer coefficients are h; = 5 W/m?-Kand h, 00, 25 W/m2-K, respectively. The heat flux through the original walls is 1.46 W/m?. The heat flux through the retrofitted walls is 0.92 W/m?.arrow_forward
- i need the answer quicklyarrow_forwardBoth ends of a 32 cm long rod are maintained a constant temperature of 100 °C. Dimensions and thermal parameters of the rod are as follows: Diameter D = 2 cm Convection coefficient h = 10 W/m2K Ambient temperature T¥ = 20 °C Thermal conductivity k = 10 W/mK What is the midpoint temperature of the rod?arrow_forwardThe composite wall of an oven consists of three materials, two of which are ofknown thermal conductivity, kA = 25 W/m ⋅ K and kC = 60 W/m ⋅ K, and knownthickness, LA = 0.40 m and LC = 0.20 m. The third material, B, which is sandwichedbetween materials A and C, is of known thickness, LB = 0.20 m, but unknownthermal conductivity kB. Under steady-state operating conditions, measurementsreveal an outer surface temperature of Ts,o = 20°C, an inner surface temperature ofTs,i = 600°C, and an oven air temperature of T∞ = 800°C. The inside convection coefficient h is known to be 25 W/m2 ⋅K. Neglecting convection transfer effect,what is the value of kB?arrow_forward
- 1. A beverage cooler is in the shape of a cube, 42 cm on each inside edge. Its 3.0-cm thick wall are made up of plastic (kr = 0.050 W/mK). When the outside temperature is 20°C, how much ice will melt each hour? Tice is 0°C. 2. One of the possible mechanisms of heat transfer in human body is conduction through body fat. Suppose that heat travels through 0.03 m of fat in reaching the skin, which has a total surface area of 1.7 m² and a temperature of 34°C. Find the amount of heat that reaches the skin in half an hour, if the temperature at the body, interior is maintained at the normal value 37°C ? Thermal conductivity of body fat is k = 0.2 J/sm°C. 3. The air in a room is at 25°C and outside temperature is 0°C. The window of the room has an area of 2m² and thickness 2mm. Calculate the rate of loss of heat by conduction through window ? Thermal conductivity for glass is 1 Wm¯'degree!.arrow_forward= Consider a large plane wall of thickness L=0.3 m, thermal conductivity k = 2.5 W/m.K, and surface area A = 12 m². The left side of the wall at x=0 is subjected to a net heat flux of ɖo = 700 W/m² while the temperature at that surface is measured to be T₁ = 80°C. Assuming constant thermal conductivity and no heat generation in the wall, (a) express the differential equation and the boundary equations for steady one- dimensional heat conduction through the wall, (b) obtain a relation for the variation of the temperature in the wall by solving the differential equation, and (c) evaluate the temperature of the right surface of the wall at x=L. Ti до L Xarrow_forwardGiven the illustration and data below. Determine the heat lost per day. T1 = 1000°C T2 T3 = 80°C A = 1 m²2 5 cm 10 сm W k = 0.09 т-к %3D W k = 1.64; т-кarrow_forward
- A square steel bar of side length w = 0.21 m has a thermal conductivity of k = 15.6 J/(s⋅m⋅°C) and is L = 2.7 m long. One end is placed near a blowtorch so that the temperature is T1 = 95° C while the other end rests on a block of ice so that the temperature is a constant T2. a. input an expression for the heat transferred to the cold end of the bar as a function of time using A=w^2 as the cross-sectional area of the bar. b. how much energy in joules was conducted in 1 hour, assuming t2=0*C c. input an expression for the mass of the water melted in one hour using Q1 from above and Lf in the latent heat of fusion. mw=arrow_forwardThree (3) bricks, specifically A, B, and C were arranged horizontally in such a way that it can be illustrated as a sandwich panel. Consider the system to be in series and in the order of Brick A, Brick B and Brick C. The outside surface temperature of Brick A is 1,500℃ and 150 ℃ for the outside surface of Brick C. The thermal conductivities for Brick A, Brick B and Brick C, are 2 ?/? °? , 0.50 ?/? °? , 60 ?/? °?. The thickness of Brick A and Brick C are 50 cm and 22 cm. The rate of heat transfer per unit area is 1,000 ?/?2 . Determine the following: The thickness of Brick B in the unit of mm. Assume that all the conditions were retain except that the thickness of Brick B was increased to 800 mm, what is the new value for the rate of heat transfer per unit area in ???/ℎ? . ??2 please explain the principles to solve thisarrow_forwardQ1/ A thick wall consists two layers of Gypsum, insulation and brick as shown in figure below. The ambient temperature is 20 °C and heat transfer coefficient is 5 W/m2. K in the left side. The surface temperature of right side is 45 °C. find the heat losses per meter length. What will happen if the insulation's thickness * .increases by 25% Gypsum k = 0.04W/m. K T = 45 °C h=5 W/m'. K T. = 20 °C 5 cm Insulation k = 0.04W/m. K Brick k = 0.69 W/m. Karrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Principles of Heat Transfer (Activate Learning wi...Mechanical EngineeringISBN:9781305387102Author:Kreith, Frank; Manglik, Raj M.Publisher:Cengage Learning
Principles of Heat Transfer (Activate Learning wi...
Mechanical Engineering
ISBN:9781305387102
Author:Kreith, Frank; Manglik, Raj M.
Publisher:Cengage Learning
Understanding Conduction and the Heat Equation; Author: The Efficient Engineer;https://www.youtube.com/watch?v=6jQsLAqrZGQ;License: Standard youtube license