Chapter 3.1, Problem 52AYU

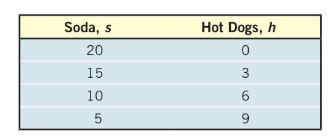
Developing a Linear Model from Data The following data represent the various combinations of soda and hot dogs that Yolanda can buy at a baseball game with $\$60$.

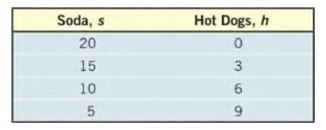
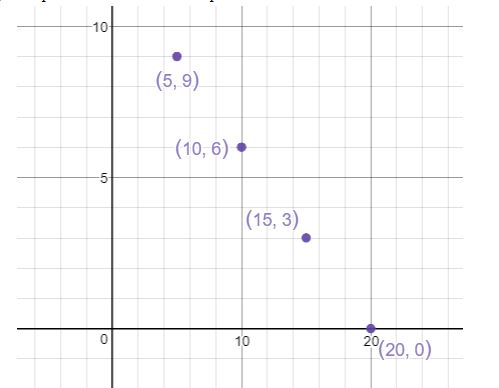
a. Plot the ordered pairs $(s,h)$ in a Cartesian plane.

b. Show that the number of hot dogs purchased $h$ is a linear function of the number of sodas purchased $s$.

c. Determine the linear function that describes the relation between $s$ and $h$.

d. What is the implied domain of the linear function?

e. Graph the linear function in the Cartesian plane drawn in part (a).

f. Interpret the slope.

g. Interpret the values of the intercepts.

To determine

To calculate:

a. Plot the ordered pairs $\left(s,h\right)$ in a Cartesian plane.

b. Show that the number of hot dogs purchased $h$ is a linear function of the number of sodas purchased $s$.

c. Determine the linear function that describes the relation between $s$ and $h$.

d. What is the implied domain of the linear function?

e. Graph the linear function in the Cartesian plane drawn in part (a..

f. Interpret the slope.

g. Interpret the values of the intercepts.

Answer to Problem 52AYU

Solution:

a. The plot of the data in Cartesian plane is as shown below:

b. Yes, it is proved that the number of hot dogs purchased $h$ is a linear function of the number of sodas purchased $s$.

c. The linear function of the given data is $y=-0.6x+12$.

d. The implied domain of the given function is $(-\infty ,\infty )$.

e. The graph is as shown below:

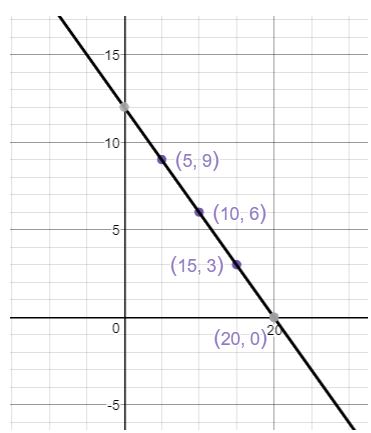
f. The function is an decreasing function.

g. Therefore, the $y\text{-intercept}$ is 12 and $x\text{-intercept}$ is 20.

Explanation of Solution

Given:

The given data is

Formula Used:

Average rate of change if a function $f(x)$ from $a$ to $b$ is $m=\frac{f(b)-f(a)}{b-a}$.

Point slope formula: $y-y_1=m(x-x_1)$

Calculation:

a. The plot of the given data in a Cartesian plane is

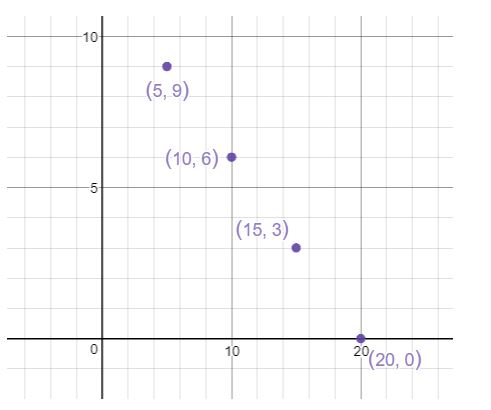
b. Here, in the above Cartesian plane, we can see that the points are arranged in a straight line format.

On joining the given points, we get a straight non vertical line.

Therefore, we can say that the given number of hot dogs purchased $h$ is a linear function of number of sodas purchased $s$.

c. From the given data, we can find the average rate of change.

When

$a=20$

$b=15$

$f(a)=0$

$f(b)=3$

Now, we get the average rate of change of the given function as

$m=\frac{3-0}{15-20}$

$=\frac{3}{-5}=-0.6$

Thus, the rate of change of the given function is $-0.6$.

Now, we have to use this in the point slope formula, where $x_1=20$ and $y_1=0$.

Thus, we get

$y-y_1=m(x-x_1)$

$\Rightarrow y-0=-0.6(x-20)$

$\Rightarrow y=-0.6x+12$

Therefore, the linear function of the given data is $y=-0.6x+12$.

d. We can see that the value of x in the linear function can be any number. Therefore, the implied domain of the given function is $(-\infty ,\infty )$.

e. The graph of the linear function is

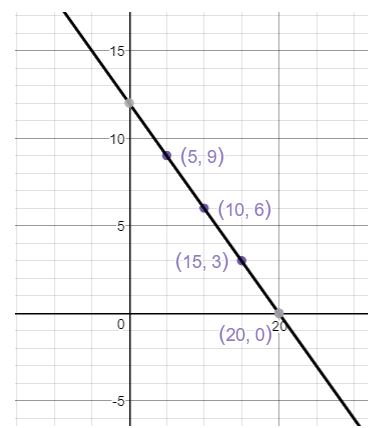
f. We know that the average rate of change of the function is itself its slope.

Thus, here we have seen that the slope is $-0.6$ which is a negative number.

Therefore, the function is an decreasing function.

g. $x\text{-intercept}$ is to be calculated when $x$ is equal to zero and $x$ intercept is to be calculated when $y$ is equal to zero.

From the function $y=-0.6x+12$, we can calculate the $x$ intercepts and $y$ intercept.

$x\text{-intercept}$:

$y=-0.6x+12$

$0=-0.6x+12$

$x=\frac{12}{0.6}$

$x=20$

$y\text{-intercept}$:

$y=-0.6(0)+12$

$y=12$

Therefore, the $y\text{-intercept}$ is 12 and $x\text{-intercept}$ is 20.