Chapter 3, Problem 19RE

In Problems 18-19, solve each quadratic inequality.

19. $3x^2 \ge 14x + 5$

To determine

To calculate: The solution to the given quadratic inequality.

Answer to Problem 19RE

The solution of the given inequality is $x\in \left[-0.333,5\right]$.

Explanation of Solution

Given:

The given inequality is $3x^2\ge 14x+5$.

Formula used:

In order to find the solution to the given quadratic inequality, we have to draw the graph of the quadratic equation and then, from the graph, determine where the function is increasing or decreasing.

Calculation:

The given inequality can also be written as

$3x^2\ge 14x+5$

$\Rightarrow 3x^2-14x-5\ge 0$

Here, we have to graph the quadratic equation $y=3x^2-14x-5$.

Let us find the $x$ and $y$ coordinates, by substituting $x=0$ and $y=0$, respectively, in the quadratic equation.

When $x=0$, we get

$y=3{(0)}^2-14(0)-5=-5$

When $y=0$, we get

$3x^2-14x-5=0$

$\Rightarrow x=\frac{-(-14)\pm \sqrt{{(-14)}^2-4(3)<mtext> </mtext>(-5)}}{2(3)}$

$\Rightarrow x=\frac{14\pm \sqrt{196+60}}{6}$

$\Rightarrow x=\frac{14\pm 16}{6}$

$\Rightarrow x=\frac{14-16}{6}=-\frac{1}{3}$

and

$\Rightarrow x=\frac{14+16}{6}=5$

Thus, the function intersects the $y\text{-axis}$ at $y=-5$ and the function has two $x\text{-intercepts}$, at $x=\frac{-1}{3}$ and $x=5$.

The vertex of the given function is

$\frac{-b}{2a}=\frac{-(-14)}{2(3)}=\frac{7}{3}$

$f\left(\frac{-b}{2a}\right)=f(2.33)=3{(\frac{7}{3})}^2-14(\frac{7}{3})-5=-\frac{64}{3}$

$\left(\frac{-b}{2a},f\left(\frac{-b}{2a}\right)\right)=\left(2.33,-21.33\right)$

Thus, the vertex is at $\left(2.33,-21.33\right)$.

The graph of the equation is

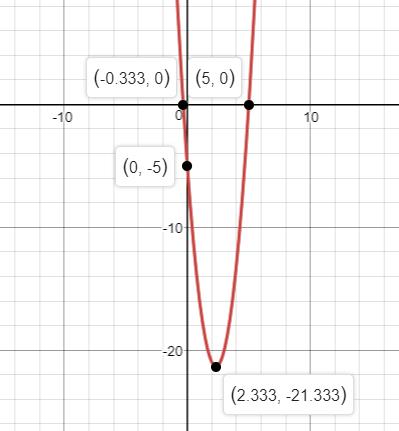
From the graph, we can see that the function is greater than or equal to 0 in the interval $\left[-0.333,5\right]$.

Thus, the solution to the given inequality is $x\in \left[-0.333,5\right]$.