
(a)
To calculate: The percentage of all eggs which weigh more than 95.5 g.
The percentage of all eggs weighing more than 95.5 g is approximately is 16%.
Given:
The mean weight of eggs is 90 g.
The standard deviation of the weight of eggs is
The distribution of weights of eggs laid by mature turkeys is
Concept used:
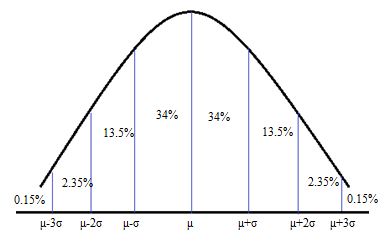
In a normal distribution, the percentage of data within one standard deviation of the mean is about 68%, the percentage of data within two standard deviations of the mean is about 95%, and the percentage of data within three standard deviations of the mean is about 99.7%. The Z score of a data value is the number of standard deviations from the mean and is calculated using the formula
where
Calculation:
The given data point is 95.5 and its Z score is calculated as shown below
The weight of 95.5 is one standard deviation more than the mean. With reference to the empirical rule, there is 68% of data within one standard deviation of the mean or there is 34% of the data between the mean and one standard deviation above the mean. Mean divides the entire data into two equal parts 50%, thus the percentage of data above 95.5 g or above one standard deviation from the mean is
Conclusion:
The percentage of eggs weighing more than 95.5 g is about 16%.
(b)
To calculate: The percentage of all eggs which are between weights 79 and 84.5 g.
The percentage of all eggs which are between weights 79 and 84.5 g is 13.5%.
Calculation:
With reference to the normal curve and empirical rule, there is about 95% of data within two standard deviations of the mean, and about 68% of the data within one standard deviation of the mean.
The Z scores for given weights are calculated as shown below
The given weights 79 g, and 84.5 g are two standard deviations less than mean and one standard deviation less than mean respectively.
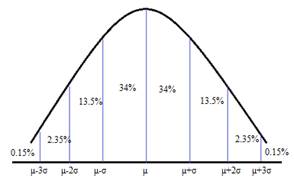
With reference to empirical rule the percentage of data between mean and one standard deviation less than mean is about 34% and the percentage of data between the mean and the two standard deviations less the mean is 47.5%. Thus the percentage of data between one standard deviation less than mean and two standard deviations less than mean is
Conclusion:
The percentage of eggs all weigh between 79 g. and 84.5 g. is about 13.5%.
(c)
To calculate: The weight of the egg which is in the top 2.5% of all eggs.
The weight of the egg which is in the top 2.5% of all eggs is 101 g.
Calculation:
With reference to the normal curve and empirical rule, there is about 95% of data within two standard deviations of the mean and about 2.5% of data in each tail.
Thus the top 2.5% of data will be over the two standard deviations above the mean and is calculated as shown below
The weight of egg 101g is two standard deviations above the mean which has the top 2.5% of the students who took the test.
Conclusion:A weight of 101 g is needed in to be the top 2.5% of all students who took the test.
(d)
To explain: If it is surprising to find a turkey egg that weigh only 72 g.
It is surprising to find an egg that weigh 72 g, as it is very less likely to occur.
Concept used:
According to empirical rule about 99.7% of data lie within three standard deviations of the mean and if any particular value has a Z score more than 3 or less than -3, then it is less very likely to occur or unusual.
Calculation:
The given weight of 72 g Z score is calculated as shown below
Interpretation:
The Z score of weight 72 g. is -3.27, so it is 3.27 standard deviations less than the mean. According to the empirical rule, about 99.7% of the data lie within three standard deviations of the mean. Thus the likelihood of an egg with a weight of 72 g. is very less. Thus it is surprising to find an egg with 72 g.
Chapter 10 Solutions
PRECALCULUS:GRAPHICAL,...-NASTA ED.
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





