Fundamentals of Heat and Mass Transfer
7th Edition
ISBN: 9780470917855
Author: Bergman, Theodore L./
Publisher: John Wiley & Sons Inc
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 5, Problem 5.88P
A simple procedure for measuring surface convection heat transfer coefficients involves coating the surface with a thin layer of material having a precise melting point temperature. The surface is then heated and, by determining the time required for melting to occur, theconvection coefficient is determined. following experimental arrangement uses the procedure to determine the convection coefficient for gas flow normal to a surface. Specifically, a long copper rod is encased in a super insulator of very low thermal conductivity, and a very thin coating is applied to its exposed surface.
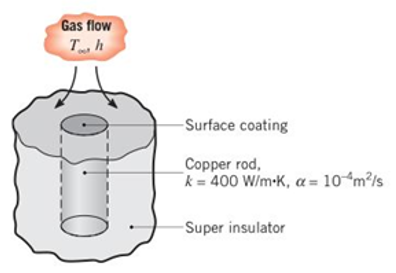
If the rod is initially at
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Composite walls with no heat generation may also be characterized by series-parallel
configurations, as shown in the following figure. Although the heat flow is now
multidimensional, it is often reasonable to assume one-dimensional conditions. Subject to this
assumption, two different thermal circuits may be used. For case (a), it is presumed that
surfaces normal to the x-direction are isothermal, while for case (b), it is assumed that surfaces
parallel to the x-direction are adiabatic. Different results are obtained for the total thermal
resistance for conduction. Here important geometrical information and thermal conductivity
information are as follows.
Length: LE-LF-LG=LH=L=1 m
Area perpendicular to x-axis: A for sections E and H, 4/2 for sections F and G where A=1 m²
Thermal conductivity: ke-ke-ku-k and ke-a-k where k-50 W/(mK) and a-10.
1-1) What is the total thermal resistance between T₁ and T2 for case (a), Rtota. The answer
should be given in the unit of K/W.
1-2)
What is the…
Please fats. The answer in the box is incorrect
=
A long rod of 60-mm diameter and thermophysical properties p 8000 kg/m³, c
C = 500 J/kg-K, and k = 50 W/m-K is initially at a
uniform temperature and is heated in a forced convection furnace maintained at 750 K. The convection coefficient is estimated to be
1000 W/m².K. What is the centerline temperature of the rod when the surface temperature is 570 K?
The centerline temperature of the rod is
i
K.
Chapter 5 Solutions
Fundamentals of Heat and Mass Transfer
Ch. 5 - Consider a thin electrical heater attached to a...Ch. 5 - The inner surface of a plane wall is insulated...Ch. 5 - A microwave oven operates on the principle that...Ch. 5 - A plate of thickness 2L, surface area As, mass M,...Ch. 5 - For each of the following cases, determine an...Ch. 5 - Steel balls 12 mm in diameter are annealed by...Ch. 5 - Consider the steel balls of Problem 5.6, except...Ch. 5 - The heat transfer coefficient for air flowing over...Ch. 5 - A solid steel sphere (AISI 1010), 300 mm in...Ch. 5 - A flaked cereal is of thickness 2L=1.2mm. The...
Ch. 5 - The base plate of an iron has a thickness of L=7mm...Ch. 5 - Thermal energy storage systems commonly involve a...Ch. 5 - A tool used for fabricating semiconductor devices...Ch. 5 - A copper sheet of thickness 2L=2mm has an initial...Ch. 5 - Carbon steel (AISI 1010) shafts of 0.1-m diameter...Ch. 5 - A thermal energy storage unit consists of a large...Ch. 5 - Small spherical particles of diameter D=50m...Ch. 5 - A spherical vessel used as a reactor for producing...Ch. 5 - Batch processes are often used in chemical and...Ch. 5 - An electronic device. such as a power transistor...Ch. 5 - Molecular electronics is an emerging field...Ch. 5 - A plane wall of a furnace is fabricated from plain...Ch. 5 - A steel strip of thickness =12mm is annealed by...Ch. 5 - In a material processing experiment conducted...Ch. 5 - Plasma spray-coating processes are often used to...Ch. 5 - The plasma spray-coating process of Problem 5.25...Ch. 5 - A chip that is of length L=5mm on a side and...Ch. 5 - Consider the conditions of Problem 5.27. In...Ch. 5 - A long wire of diameter D=1mm is submerged in an...Ch. 5 - Consider the system of Problem 5.1 where the...Ch. 5 - Shape memory alloys (SMAs) are metals that undergo...Ch. 5 - Before being injected into a furnace, pulverized...Ch. 5 - As noted in Problem 5.3, microwave ovens operate...Ch. 5 - A metal sphere of diameter D, which is at a...Ch. 5 - A horizontal structure consists of an LA=10...Ch. 5 - As permanent space stations increase in size....Ch. 5 - Thin film coatings characterized by high...Ch. 5 - A long. highly polished aluminum rod of diameter...Ch. 5 - Thermal stress testing is a common procedure used...Ch. 5 - The objective of this problem is to develop...Ch. 5 - In thermomechanical data storage, a processing...Ch. 5 - The melting of water initially at the fusion...Ch. 5 - Consider the series solution, Equation 5.42, for...Ch. 5 - Consider the one-dimensional wall shown in the...Ch. 5 - Copper-coated, epoxy-tilled fiberglass circuit...Ch. 5 - Circuit boards are treated by heating a stack of...Ch. 5 - A constant-property, one-dimensional plane slab of...Ch. 5 - Referring to the semiconductor processing tool of...Ch. 5 - Annealing is a process by which steel is reheated...Ch. 5 - Consider an acrylic sheet of thickness L=5mm that...Ch. 5 - The 150-mm-thick wall of a gas-fired furnace is...Ch. 5 - Steel is sequentially heated and cooled (annealed)...Ch. 5 - Stone mix concrete slabs are used to absorb...Ch. 5 - During transient operation, the steel nozzle of a...Ch. 5 - Two plates of the same material and thickness L...Ch. 5 - In a tempering process, glass plate, which is...Ch. 5 - The strength and stability of tires may be...Ch. 5 - A plastic coating is applied to wood panels by...Ch. 5 - A long rod of 60-mm diameter and thermophysical...Ch. 5 - A long cylinder of 30-mm diameter, initially at a...Ch. 5 - A long pyroceram rod of diameter 20 mm is clad...Ch. 5 - A long rod 40 mm in diameter, fabricated from...Ch. 5 - A cylindrical stone mix concrete beam of diameter...Ch. 5 - A long plastic rod of 30-mm diameter...Ch. 5 - As part of a heat treatment process, cylindrical,...Ch. 5 - In a manufacturing process, long rods of different...Ch. 5 - The density and specific heat of a particular...Ch. 5 - In heat treating to harden steel ball bearings...Ch. 5 - A cold air chamber is proposed for quenching steel...Ch. 5 - Stainless steel (AISI 304) ball bearings. which...Ch. 5 - A sphere 30 mm in diameter initially at 800K is...Ch. 5 - Spheres A and B are initially at 800K. and they...Ch. 5 - Spheres of 40-mm diameter heated to a uniform...Ch. 5 - To determine which parts of a spider's brain are...Ch. 5 - Consider the packed bed operating conditions of...Ch. 5 - Two large blocks of different materials. such as...Ch. 5 - A plane wall of thickness 0.6 m (L=0.3m) is made...Ch. 5 - Asphalt pavement may achieve temperatures as high...Ch. 5 - A thick steel slab...Ch. 5 - A tile-iron consists of a massive plate maintained...Ch. 5 - A simple procedure for measuring surface...Ch. 5 - An insurance company has hired you as a consultant...Ch. 5 - A procedure for determining the thermal...Ch. 5 - A very thick slab with thermal diffusivity...Ch. 5 - Standards for firewalls may be based on their...Ch. 5 - It is well known that, although two materials are...Ch. 5 - Two stainless steel plates...Ch. 5 - Special coatings are often formed by depositing...Ch. 5 - When a molten metal is cast in a mold that is a...Ch. 5 - Joints of high quality can be formed by friction...Ch. 5 - A rewritable optical disc (DVD) is formed by...Ch. 5 - Ground source heat pumps operate by using the...Ch. 5 - To enable cooking a wider range of foods in...Ch. 5 - Derive an expression for the ratio of the total...Ch. 5 - The structural components of modem aircraft are...Ch. 5 - Consider the plane wall of thickness 2L, the...Ch. 5 - Problem 4.9 addressed radioactive wastes stored...Ch. 5 - Derive an expression for the ratio of the total...Ch. 5 - Prob. 5.107PCh. 5 - Prob. 5.108PCh. 5 - A thin rod of diameter D is initially in...Ch. 5 - A one-dimensional slab of thickness 2L is...Ch. 5 - Prob. 5.114PCh. 5 - Prob. 5.115PCh. 5 - A molded plastic product...Ch. 5 - Prob. 5.133PCh. 5 - A thin circular disk is subjected to induction...Ch. 5 - Two very long (in the direction normal to the...Ch. 5 - Prob. 5S.2PCh. 5 - Prob. 5S.3PCh. 5 - Estimate the time required to cook a hot dog in...Ch. 5 - Prob. 5S.7PCh. 5 - Prob. 5S.9PCh. 5 - Prob. 5S.10PCh. 5 - Prob. 5S.11PCh. 5 - Prob. 5S.13P
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- 1.4 To measure thermal conductivity, two similar 1-cm-thick specimens are placed in the apparatus shown in the accompanying sketch. Electric current is supplied to the guard heater, and a wattmeter shows that the power dissipation is 10 W. Thermocouples attached to the warmer and to the cooler surfaces show temperatures of 322 and 300 K, respectively. Calculate the thermal conductivity of the material at the mean temperature in W/m K. Problem 1.4arrow_forwardA section of a composite wall with the dimensions shown below has uniform temperatures of 200C and 50C over the left and right surfaces, respectively. If the thermal conductivities of the wall materials are: kA=70W/mK,kB=60W/mK, kC=40W/mK, and kP=20W/mK, determine the rate of heat transfer through this section of the wall and the temperatures at the interfaces. Repeat Problem 1.34, including a contact resistance of 0.1 K/W at each of the interfaces.arrow_forwardBoth ends of a 0.6-cm copper U-shaped rod are rigidly affixed to a vertical wall as shown in the accompanying sketch. The temperature of the wall is maintained at 93C. The developed length of the rod is 0.6 m, and it is exposed to air at 38C. The combined radiation and convection heat transfer coefficient for this system is 34W/m2K. (a) Calculate the temperature of the midpoint of the rod. (b) What will the rate of heat transfer from the rod be?arrow_forward
- I need the answer as soon as possiblearrow_forwardStainless steel (AISI 304) ball bearings, which have been uniformly heated to 850°C, are hardened by quenching them in an oil bath that is maintained at 40°C. The ball diameter is 12.5 mm, and the convection coefficient associated with the oil bath is 1000 W/m².K. (a) If quenching is to occur until the surface temperature of the balls reaches 100°C, how long, in s, must the balls be kept in the oil? What is the center temperature, in °C, at the conclusion of the cooling period? (b) If 10,000 balls are to be quenched per hour, what is the rate at which energy must be removed, in kW, by the oil bath cooling system in order to maintain its temperature at 40°C? Evaluate the properties of the stainless steel (AISI 304) at 500 K. Part A If quenching is to occur until the surface temperature of the balls reaches 100°C, how long, in s, must the balls be kept in the oil? What is the center temperature, in °C, at the conclusion of the cooling period? t = T₁ = i i S °℃arrow_forwardThermal treatment of a steel cube, of 10 cm side length, L, requires a two step cooling process. In the first step, the cube at the uniform temperature of 500°C is placed in air where it is cooled to 250°C. In the second step, it is moved to large oil bath of temperature 20°C where the heat transfer coefficient at all surfaces is the same and equal to h = 50 W m-2 K-1. %3D Calculate the total amount of energy lost by the cube and show that the lumped capacitance method can be used to calculate the temperature during the second step of cooling. Calculate the time necessary to reduce the cube temperature to 50°C. Thermal properties Density = 8000 kg m-3 Cp= 480 J kg-1 K-1 k = 30 W m-1 K-1. %3Darrow_forward
- Sepleniber 02 Heat is generated in a homogeneous spherical piece of radioactive material (2D - 8 cm) at rate of 71.8 MW/m and is dissipated to the environment. The center and surface temperatures of the spheres are (491°C and 80°C) respectively, What is the thermal conductivity of the radioactive material "W/m.K"?arrow_forwardAn underwater sonar that maps the ocean bathymetry is encapsulated in a sphere with a diameter of 85 mm. During operation, the sonar generates heat at a rate of 300W. What is the sonar surface temperature when it’s located in a water column where the temperature is 15o C and the water current is 1 m/sec? The sonar was pulled out of the water without turning it off, thus, it was still working. The air temperature was 15o C and the air speed was 3 m/sec. What was the sonar surface temperature? Was there any reason for concern?arrow_forwardDon’t use Heissler charts to answer this question Heat sterilization of lumber, timbers, and pallets is used to kill insects to prevent their transfer between countries in international trade. This is analogous to food sterilization by heat. A typical requirement here is that the slowest heating point of any woodconfiguration be held at 56 °C for 30 minutes. Consider hot air heating of wooden boards that maintains their surface temperature at 70 °C. The boards are stacked outside and in the winter time they can be considered to be at 0 °Cwhen theyare brought in for heating. The thermal diffusivity of the wood is 9*10-8m2/s. a.Calculate the time from the start of heating for a 2.5 cm thick board to reach a sterilization temperature of 56 °C at its slowest heating point .b.Calculate the heating time when four such boards are stacked together. c.Calculate the ratio of the two heating times (for a single board versus when they are stacked), and explain the ratio. Note: You’re free to…arrow_forward
- To reduce heat flux, the skin is wrapped with a clothing material. What should be the thickness of the clothing material covering the surface of this skin tissue to reduce the heat flux to half of its original value? What is the temperature at the skin-clothing material interface? Note: if you think you need to have more information to solve this problem, you're free to make assumptions. Please state them clearly in your answer, if you need to make such assumptions.arrow_forwardThe wall of an industrial furnace, a 0.15 m thick chamotte with a heat conduction coefficient of 1.7 M / m.K It is made of bricks. Measurements made during continuous operation, internal and external surface temperatures showed that it was 1400 and 1150 K respectively. With side lengths of 0.5 m and 3 m calculate the heat passing through the wall per unit timearrow_forward(B) In order to measure the convection heat transfer coefficients one of the common methods involve bonding one surface of a thin metallic foil to an insulating material and exposing the other surface to the fluid flow conditions of interest. T, h -Foil (Pälec T) - Foam Insulation (k) Figure1: Heat transfer direction By passing an electric current through the foil, heat is dissipated uniformly within the foil and the corresponding flux (P elec) may be inferred from related voltage and current measurements. Consider conditions for which To = Tb = 25°C, Pelec = 2000 W/m2, L = 7 mm, and k = 0.05 W/m.K. (1) With water flow over the surface, the foil temperature measurement yields Ts= 30°C. Determine the convection heat transfer coefficient for both cases below. The foil has an emissivity of 0.15 and is exposed to large surroundings at 25°C. (2) On the other hand, if air flows over the surface and the temperature measurement yields Ts = 120°C, how the convection heat transfer coefficient…arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Principles of Heat Transfer (Activate Learning wi...Mechanical EngineeringISBN:9781305387102Author:Kreith, Frank; Manglik, Raj M.Publisher:Cengage Learning
Principles of Heat Transfer (Activate Learning wi...
Mechanical Engineering
ISBN:9781305387102
Author:Kreith, Frank; Manglik, Raj M.
Publisher:Cengage Learning
Heat Transfer – Conduction, Convection and Radiation; Author: NG Science;https://www.youtube.com/watch?v=Me60Ti0E_rY;License: Standard youtube license