Chapter 2.2, Problem 30AYU

(a)

To determine

To find: the initial velocity with which the ball must be shot in order for the ball to go through the hoop.

Answer to Problem 30AYU

The initial velocity is 30 feet per second.

Explanation of Solution

Given information:

A player shoots an underhand foul shot, releasing the ball at a 70 degree angle from a position 3.5 feet above the floor, then the ball can be modeled by the function $h\left(x\right)=-\frac{136x^2}{v^2}+2.7x+3.5$ , where $h$ is the height of the ball above the floor, $x$ is the forward distance of the ball in front of the foul line, and $v$ is the initial velocity with which the ball is shot in feet per second.

Calculation:

Substitute 10 for $h$ , and 15 for $x$ in the given model.

  $10=-\frac{136{\left(15\right)}^2}{v^2}+2.7\left(15\right)+3.5$

Simplify.

  $\begin{array}{l}10=-\frac{30600}{v^2}+40.5+3.5\\ 10=-\frac{30600}{v^2}+44\end{array}$

Isolate $v^2$ . Subtract 44 from each side.

  $\begin{array}{l}10-44=-\frac{30600}{v^2}+44-44\\ -34=-\frac{30600}{v^2}\end{array}$

Multiply $-\frac{v^2}{34}$ to each side.

  $\begin{array}{c}\left(-34\right)\left(-\frac{v^2}{34}\right)=\left(-\frac{30600}{v^2}\right)\left(-\frac{v^2}{34}\right)\\ v^2=\frac{30600}{34}\\ v^2=900\end{array}$

Take the positive square root on both the sides.

  $\begin{array}{c}\sqrt{v^2}=\sqrt{900}\\ v=30\end{array}$

The initial velocity is 30 feet per second.

(b)

To determine

To write: the function for the path of the ball using the velocity found in part (a).

Answer to Problem 30AYU

The function is

  $h\left(x\right)=-\frac{136x^2}{900}+2.7x+3.5$ .

Explanation of Solution

Given information:

A player shoots an underhand foul shot, releasing the ball at a 70 degree angle from a position 3.5 feet above the floor, then the ball can be modeled by the function $h\left(x\right)=-\frac{136x^2}{v^2}+2.7x+3.5$ , where $h$ is the height of the ball above the floor, $x$ is the forward distance of the ball in front of the foul line, and $v$ is the initial velocity with which the ball is shot in feet per second.

Calculation:

We know that the initial velocity is 30 per second. Substitute 30 for $v$ in the given model and simplify.

  $\begin{array}{l}h\left(x\right)=-\frac{136x^2}{{30}^2}+2.7x+3.5\\ h\left(x\right)=-\frac{136x^2}{900}+2.7x+3.5\end{array}$

(c)

To determine

To find: the height of the ball after it has traveled 9 feet in front of the foul line.

Answer to Problem 30AYU

The height of the ball after it has traveled 9 feet in front of the foul line is 15.56 feet.

Explanation of Solution

Given information:

A player shoots an underhand foul shot, releasing the ball at a 70 degree angle from a position 3.5 feet above the floor, then the ball can be modeled by the function $h\left(x\right)=-\frac{136x^2}{v^2}+2.7x+3.5$ , where $h$ is the height of the ball above the floor, $x$ is the forward distance of the ball in front of the foul line, and $v$ is the initial velocity with which the ball is shot in feet per second.

Calculation:

We know that the function obtained is $h\left(x\right)=-\frac{136x^2}{900}+2.7x+3.5$ . Substitute 9 for $x$ in the model and evaluate.

  $\begin{array}{c}h\left(x\right)=-\frac{136x^2}{900}+2.7x+3.5\\ =-\frac{11016}{900}+24.3+3.5\\ =-\frac{11016}{900}+27.8\\ =15.56\end{array}$

Therefore, the height of the ball after it has traveled 9 feet in front of the foul line is 15.56 feet.

(d)

To determine

To find: the height of the ball after it has traveled 9 feet in front of the foul line.

Answer to Problem 30AYU

The height of the ball will be 9.7feet in negative direction.

Explanation of Solution

Given information:

A player shoots an underhand foul shot, releasing the ball at a 70 degree angle from a position 3.5 feet above the floor, then the ball can be modeled by the function $h\left(x\right)=-\frac{136x^2}{v^2}+2.7x+3.5$ , where $h$ is the height of the ball above the floor, $x$ is the forward distance of the ball in front of the foul line, and $v$ is the initial velocity with which the ball is shot in feet per second.

Calculation:

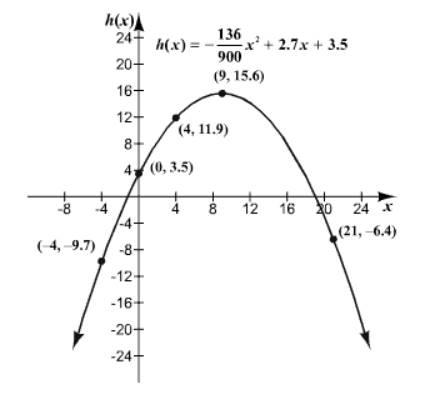
We know that a point on the graph is $\left(9,15.56\right)$ . Substitute any value, say $-4$ for $x$ in

  $h\left(x\right)=-\frac{136x^2}{900}+2.7x+3.5$ and evaluate.

  $\begin{array}{c}h\left(-4\right)=-\frac{136{\left(-4\right)}^2}{900}+2.7\left(-4\right)+3.5\\ =-\frac{2176}{900}-10.8+3.5\\ \approx -9.7\end{array}$

Choose some more value for $x$ and find the corresponding $y$ values. Organize the results in a table.

$x$	$\left(x,h\left(x\right)\right)$
$-4$	$-9.7$
0	3.5
4	11.9
9	15.6
21	$-6.4$

Plot the points from the table on a coordinate plane and connect them.