7th Edition

ISBN: 9780470917855

Author: Bergman, Theodore L./

Publisher: John Wiley & Sons Inc

See similar textbooks

Videos

Textbook Question

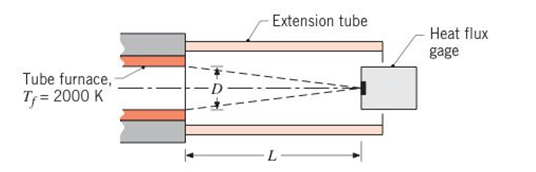
Chapter 12, Problem 12.30P

A furnace with a long, isothermal, graphite tube of diameter D = 12.5 m m is maintained at T f = 2000 K and is used as a blackbody source to calibrate heat flux gages. Traditional heat flux gages are constructed as blackened thin films with thermopiles to indicate the temperature change caused by absorption of the incident radiant power over the entire spectrum. The traditional gage of interest has a sensitive area of 5 mm2 and is mounted coaxial with the furnace centerline, but positioned at a distance of L = 60 m m from the beginning of the heated section. The cool extension tube serves to shield the gage from extraneous radiation sources and to contain the inert gas required to prevent rapid oxidation of the graphite tube

Chapter 12, Problem 12.30P, A furnace with a long, isothermal, graphite tube of diameter D=12.5mm is maintained at Tf=2000K and

(a) Calculate the heat flux (W/m2) on the traditional gage for this condition, assuming that the extension tube is cold relative to the furnace.

(b) The traditional gage is replaced by a solid-state (photoconductive) heat flux gage of the same area, but sensitive only to the spectral region between 0.4 a n d 2.5 μ m . Calculate the radiant heat flux incident on the solid-state gage within the pre- scribed spectral region.

(c) Calculate and plot the total heat flux and the heat flux in the prescribed spectral region for the solid- state gage as a function of furnace temperature for the range . Which gage will have an output signal that is more sensitive to changes in the furnace temperature?

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 12, Problem 12.28P

Chapter 12, Problem 12.33P

Students have asked these similar questions

An infrared camera is used to measure a temperature at a tissue location. The infrared camera uses the same equation as that in the lecture notes. When the total hemispherical emissivity is selected as &=1.0, the temperature reading on the camera is 45°C. (a) Based on the equation given in the notes, please calculate the radiation heat flux received by the camera qck. The Stefan-Boltzmann's constant ois 5.67*108 W/(m²K¹). (b) However, you notice that the actual emissivity of the tissue surface should be 0.95. The room temperature is 20°C. Use the equation again to calculate the temperature of the tissue location, note that qck should be the same as in (a). What is the absolute error of the measurement if both the room temperature and deviation from a perfect blackbody surface are not considered?

Using a polynomial equation solver, determine the steady-state surface temperature (Ts) of the passive radiative cooler below that is exposed to a windy convective enviroment h=40 W/m2*K and T∞= 25°C. The surrounding atmosphere is fixed at Tsur= 10°C. Set up the energy balance/conservation equation.

A long, horizontal, cylindrical steel reactor, 1 m in diameter, has a surface temperature of 300ºC. The emissivity of the steel is 0.6, and the heat transfer coefficient for natural convection is 5 W m−2 K−1 . Heat is lost by convection to the air at 15ºC, and also by radiation to the surroundings, which can be considered to be a black body at 15ºC. a) Calculate the total heat loss per metre length of the reactor, and the proportions lost by convection and radiation b) The reactor is then insulated with a thin layer of insulation material to reduce the total heat loss to one-tenth of its original value. This causes the surface temperature of the steel to rise to 400ºC. The thermal conductivity of the insulation is 0.01 W m−1 K−1 , and its surface emissivity is 0.2. Show that the resulting surface temperature of the insulation is about 89ºC, and calculate the thickness of insulation required, stating any assumptions made. Specifically need help with part b

Chapter 12 Solutions

Fundamentals of Heat and Mass Transfer

Ch. 12 - Consider an opaque horizontal plate that is well...Ch. 12 - A horizontal, opaque surface at a steady-state...Ch. 12 - The top surface of an L=5mmthick anodized aluminum...Ch. 12 - A horizontal semitransparent plate is uniformly...Ch. 12 - What is the irradiation at surfaces A2 , A3 , and...Ch. 12 - According to its directional distribution, solar...Ch. 12 - Solar radiation incident on the earth’s surface...Ch. 12 - On an overcast day the directional distribution of...Ch. 12 - During radiant heat treatment of a thin-film...Ch. 12 - A small radiant heat source of area A1=2x104m2...

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.

Solution Summary: The author calculates the heat flux to the gage (or the irradiation) using the following formula: q=9840W/m2

Videos

Want to see the full answer?

Chapter 12 Solutions