
Growers use giant fans to prevent grapes from freezing when the effective sky temperature is low. The grape, which may be viewed as a thin skin of negligible thermal resistance enclosing a volume of sugar water, is exposed to ambient air and is irradiated from the sky above and ground below. Assume the grape to be an isothermal sphere of 15-mm diameter, and assume uniform blackbody irradiation over itstop and bottom hemispheres due to emission from the sky and the earth, respectively.
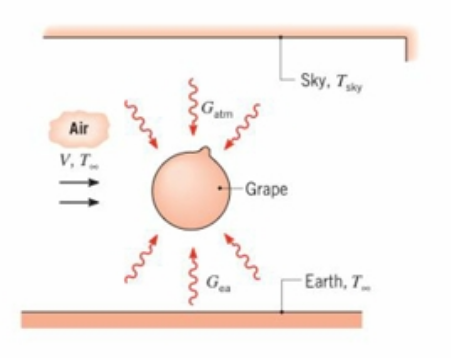
(a) Derive an expression for the rate of change of the grape temperature. Express your result in terms of a convection coefficient and appropriate temperatures and radiative quantities.
(b) Under conditions for which
(c) With all conditions remaining the same, except that the fans are now operating with
Want to see the full answer?
Check out a sample textbook solution
Chapter 12 Solutions
Fundamentals of Heat and Mass Transfer
- Two large parallel plates with surface conditions approximating those of a blackbody are maintained at 816C and 260C, respectively. Determine the rate of heat transfer by radiation between the plates in W/m2 and the radiative heat transfer coefficient in W/m2K.arrow_forward11.31 A large slab of steel 0.1 m thick contains a 0.1 -m-di- ameter circular hole whose axis is normal to the surface. Considering the sides of the hole to be black, specify the rate of radiative heat loss from the hole. The plate is at 811 K, and the surroundings are at 300 K.arrow_forward1.28 The sun has a radius of and approximates a blackbody with a surface temperature of about 5800 K. Calculate the total rate of radiation from the sun and the emitted radiation flux per square meter of surface area.arrow_forward
- Determine the total average hemispherical emissivity and the emissive power of a surface that has a spectral hemispherical emissivity of 0.8 at wavelengths less than 1.5m, 0.6 at wavelengths from 1.5to2.5m, and 0.4 at wavelengths longer than 2.5m. The surface temperature is 1111 K.arrow_forwardA tungsten filament is heated to 2700 K. At what wavelength is the maximum amount of radiation emitted? What fraction of the total energy is in the visible range (0.4to0.75m)? Assume that the filament radiates as a graybody.arrow_forwardLiquid oxygen is stored in a thin-walled, spherical container 0.8 m in diameter, which is enclosed within a second thin-walled, spherical container 1.4 m in diameter. The opaque, diffuse, gray container surfaces have an emissivity of 0.05 and are separated by an evacuated space. If the outer surface is at 275 K and the inner surface is at 95 K, what is the mass rate of oxygen lost due to evaporation, in kg/s? (The latent heat of vaporization of oxygen is 2.13 × 105 J/kg.)arrow_forward
- A gray surface has an emissivity at a temperature of 550 K source. If the surface is opaque, (a) Calculate its reflectivity for black body radiation coming from a 550 K source. (b) A small 25 mm square hole is made in the thin-walled door of a furnace whose inside walls are at 920 K. if the emissivity of the walls is 0.72, calculate the rate at which radiant energy escapes from the furnace through the hole to the room.arrow_forward Two perfectly black parallel planes 1.2 by 1.2mare separated by a distance of 1.2 m. One plane is maintained at 800 K and the other at 500 K. The plates are located in a large room whose walls are at 300 K. What is the net heat transfer between the planes?arrow_forwardQuestion #9 A circular ceramic plate that can be modelled as a blackbody is being heated by an electrical heater. The plate is 30cm in diameter and is situated in a surrounding ambient temperature of 15°C where the natural convection heat transfer coefficient is 12W/m² K. The efficiency of the electrical heater to transfer heat to the plate is 80%, the electric power is required such that the heater needs to keep the surface temperature of the plate at 200°C. Ambient 15°C Tsurr = 15°C h = 12 W/m².K Ceramic plate -T₂ = 200°C Welec (A) Determine the heat emitted from the plate, as a blackbody. (B) Determine the radiation incident on the plate from the surroundings. (C) Determine the heat transfer from the plate to the surroundings. (D) Determine the required electric power.arrow_forward
- A spherical satellite of 2m diameter circles the earth at an altitude of 600 miles. Assuming all surfaces are black, calculate the equilibrium temperature of the satellite on the 'dark' side and on the 'bright' side of the earth. Assume the earth has a radius of 4,000 miles and a temperature of 200C. The suns temperature can be taken to be 6,700K and it has a radius of 433,000 miles. The distance between the sun and the earth is 93,000,000 miles. View factor of a small sphere to a much larger one: S→L Where R = '2 ri is the radius of the large sphere and x is the separation distance between the spheres.arrow_forwardAn engineered passive radiative cooler coating is placed under the Sun. Provided the following simplified spectral emissivity/absorptivity plot below, calculate the total diffuse emissivity and absorptivity if its uniform surface temperature is a Ts=20°C. Assume the Sun's irradiation onk Earth is Gsun=1380 W/m2 and its blackbody temperature is Tsun= 5800K. Ignore the radiation from the atmosphere (surroundings).arrow_forwardAn opaque, diffuse, gray, square (200 mm x 200 mm) plate with an emissivity of 0.8 is placed over the opening of a furnace (L = 200 mm) and the plate temperature is known to be 400 K at a certain instant. The bottom of the furnace, having the same dimensions as the plate, is black and operates at 1040 K. The sidewalls of the furnace are well insulated. The top of the plate is exposed to ambient air with a convection coefficient of 25 W/m².K and to large surroundings. The air and surroundings are each at 300 K. Air 9₁ = To h = W -Plate (a) Evaluate the net radiative heat transfer to the bottom surface of the plate, in W. T SUT -Insulated sidewalls -Furnace bottom O (b) If the plate has mass and specific heat of 2 kg and 900 J/kg-K, respectively, what will be the change in temperature of the plate with time, dTp/dt, in K/s? Assume convection to the bottom surface of the plate to be negligible. dT₁ = K/s dtarrow_forward
 Principles of Heat Transfer (Activate Learning wi...Mechanical EngineeringISBN:9781305387102Author:Kreith, Frank; Manglik, Raj M.Publisher:Cengage Learning
Principles of Heat Transfer (Activate Learning wi...Mechanical EngineeringISBN:9781305387102Author:Kreith, Frank; Manglik, Raj M.Publisher:Cengage Learning
