Fundamentals of Physics Extended
10th Edition
ISBN: 9781118230725
Author: David Halliday, Robert Resnick, Jearl Walker
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 6, Problem 55P
A bolt is threaded onto one end of a thin horizontal rod, and the rod is then rotated horizontally about its other end. An engineer monitors the motion by flashing a strobe lamp onto the rod and bolt, adjusting the strobe rate until the bolt appears to be in the same eight places during each full rotation of the rod (Fig. 6-42). The strobe rate is 2000 flashes per second; the bolt has mass 30 g and is at radius 3.5 cm. What is the magnitude of the force on the bolt from the rod?
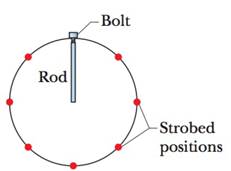
Figure 6-42 Problem 55.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
(a) Evaluate the gravitational potential energy (in J) between two 4.00 kg spherical steel balls separated by a center-to-center distance of 19.0 cm.
(b) Assuming that they are both initially at rest relative to each other in deep space, use conservation of energy to find how fast (in m/s) will they each be traveling upon impact. Each sphere has a radius of 5.50 cm.
m/s
Very far from earth (at R = o0), a spacecraft has run out of
fuel and its kinetic energy is zero. If only the gravitational
force of the earth were to act on it (i.e., neglect the forces
from the sun and other solar system objects), the spacecraft
would eventually crash into the earth. The mass of the earth
is Me and its radius is Re. Neglect air resistance throughout
this problem, since the spacecraft is primarily moving through
the near vacuum of space.
Part A
Find the speed se of the spacecraft when it crashes into the earth.
Express the speed in terms of Me, Re, and the universal gravitational constant G.
Se =
Part B
Now find the spacecraft's speed when its distance from the center of the earth is R= aRe, where the coefficient a >1.
Express the speed in terms of se and a.
Sa =
Very far from earth (at R=∞), a spacecraft has run out of fuel and its kinetic energy is zero. If only the gravitational force of the earth were to act on it (i.e., neglect the forces from the sun and other solar system objects), the spacecraft would eventually crash into the earth. The mass of the earth is Me and its radius is Re. Neglect air resistance throughout this problem, since the spacecraft is primarily moving through the near vacuum of space.
Find the speed se of the spacecraft when it crashes into the earth.
Express the speed in terms of Me, Re, and the universal gravitational constant G. Use a conservation-law approach. Specifically, consider the mechanical energy of the spacecraft when it is (a) very far from the earth and (b) at the surface of the earth.
Chapter 6 Solutions
Fundamentals of Physics Extended
Ch. 6 - In Fig. 6-12, if the box is stationary and the...Ch. 6 - Prob. 2QCh. 6 - In Fig. 6-13, horizontal force F1 of magnitude 10...Ch. 6 - In three experiments, three different horizontal...Ch. 6 - If you press an apple crate against a wall so hard...Ch. 6 - In Fig. 6-14, a block of mass m is held stationary...Ch. 6 - Reconsider Question 6 but with the force F now...Ch. 6 - In Fig. 6-15, a horizontal force of 100 N is to be...Ch. 6 - Prob. 9QCh. 6 - Prob. 10Q
Ch. 6 - A person riding a Ferris wheel moves through...Ch. 6 - During a routine flight in 1956, test pilot Tom...Ch. 6 - A box is on a ramp that is at angle to the...Ch. 6 - The floor of a railroad flatcar is loaded with...Ch. 6 - In a pickup game of dorm shuffleboard, students...Ch. 6 - SSM WWW A bedroom bureau with a mass of 45 kg,...Ch. 6 - A slide-loving pig slides down a certain 35 slide...Ch. 6 - GO A 2.5 kg block is initially at rest on a...Ch. 6 - A baseball player with mass m 79 kg, sliding into...Ch. 6 - SSM ILW A person pushes horizontally with a force...Ch. 6 - The mysterious sliding stones. Along the remote...Ch. 6 - GO A 3.5 kg block is pushed along a horizontal...Ch. 6 - Figure 6-20 shows an initially stationary block of...Ch. 6 - SSM A 68 kg crate is dragged across a floor by...Ch. 6 - In about 1915, Henry Sincosky of Philadelphia...Ch. 6 - A worker pushes horizontally on a 35 kg crate with...Ch. 6 - Figure 6-22 shows the cross section of a road cut...Ch. 6 - The coefficient of static friction between Teflon...Ch. 6 - A loaded penguin sled weighing 80 N rests on a...Ch. 6 - In Fig. 6-24, a force P acts on a block weighing...Ch. 6 - GO You testify as an expert witness in a case...Ch. 6 - A 12 N horizontal force F pushes a block weighing...Ch. 6 - GO In Fig. 6-27, a box of Cheerios mass mC = 1.0...Ch. 6 - An initially stationary box of sand is to be...Ch. 6 - GO In Fig. 6-23, a sled is held on an inclined...Ch. 6 - When the three blocks in Fig. 6-29 are released...Ch. 6 - A 4.10 kg block is pushed along a floor by a...Ch. 6 - SSM WWW Block B in Fig. 6-31 weighs 711 N. The...Ch. 6 - GO Figure 6-32 shows three crates being pushed...Ch. 6 - GO Body A in Fig. 6-33 weighs 102 N, and body B...Ch. 6 - In Fig. 6-33, two blocks are connected over a...Ch. 6 - GO In Fig. 6-34, blocks A and B have weights of 44...Ch. 6 - A toy chest and its contents have a combined...Ch. 6 - SSM Two blocks, of weights 3.6 N and 7.2 N, are...Ch. 6 - GO A block is pushed across a floor by a constant...Ch. 6 - SSM A 1000 kg boat is traveling at 90 km/h when...Ch. 6 - GO In Fig. 6-37, a slab of mass m1= 40 kg rests on...Ch. 6 - ILW The two blocks m = 16 kg and M = 88 kg in Fig....Ch. 6 - The terminal speed of a sky diver is 160 km/h in...Ch. 6 - Continuation of Problem 8. Now assume that Eq....Ch. 6 - Assume Eq. 6-14 gives the drag force on a pilot...Ch. 6 - Calculate the ratio of the drag force on a jet...Ch. 6 - In downhill speed skiing a skier is retarded by...Ch. 6 - A cat dozes on a stationary merry-go-round in an...Ch. 6 - Suppose the coefficient of static friction between...Ch. 6 - ILW What is the smallest radius of an unbanked...Ch. 6 - During an Olympic bobsled run, the Jamaican team...Ch. 6 - SSM ILW A student of weight 667 N rides a steadily...Ch. 6 - A police officer in hot pursuit drives her car...Ch. 6 - A circular-motion addict of mass 80 kg rides a...Ch. 6 - A roller-coaster car at an amusement park has a...Ch. 6 - GO In Fig. 6-39, a car is driven at constant speed...Ch. 6 - An 85.0 kg passenger is made to move along a...Ch. 6 - SSM WWW An airplane is flying in a horizontal...Ch. 6 - An amusement park ride consists of a car moving in...Ch. 6 - An old streetcar rounds a flat corner of radius...Ch. 6 - In designing circular rides for amusement parks,...Ch. 6 - A bolt is threaded onto one end of a thin...Ch. 6 - GO A banked circular highway curve is designed for...Ch. 6 - GO A puck of mass m = 1.50 kg slides in a circle...Ch. 6 - Brake or turn? Figure 6- 44 depicts an overhead...Ch. 6 - SSM ILW In Fig. 6-45, a 1.34 kg ball is connected...Ch. 6 - GO In Fig. 6-46, a box of ant aunts total mass m1...Ch. 6 - SSM A block of mass mt = 4.0 kg is put on top of a...Ch. 6 - A 5.00 kg stone is rubbed across the horizontal...Ch. 6 - In Fig. 6-49, a 49 kg rock climber is climbing a...Ch. 6 - A high-speed railway car goes around a flat,...Ch. 6 - Continuation of Problems 8 and 37. Another...Ch. 6 - GO In Fig. 6-50, block 1 of mass m1 = 2.0 kg and...Ch. 6 - In Fig. 6-51, a crate slides down an inclined...Ch. 6 - Engineering a highway curve. If a car goes through...Ch. 6 - A student, crazed by final exams, uses a force P...Ch. 6 - GO Figure 6-53 shows a conical pendulum, in which...Ch. 6 - An 8.00 kg block of steel is at rest on a...Ch. 6 - A box of canned goods slides down a ramp from...Ch. 6 - In Fig. 6-54, the coefficient of kinetic friction...Ch. 6 - A 110 g hockey puck sent sliding over ice is...Ch. 6 - A locomotive accelerates a 25-car train along a...Ch. 6 - A house is built on the top of a hill with a...Ch. 6 - What is the terminal speed of a 6.00 kg spherical...Ch. 6 - A student wants to determine the coefficients of...Ch. 6 - SSM Block A in Fig. 6-56 has mass mA = 4.0 kg, and...Ch. 6 - Calculate the magnitude of the drag force on a...Ch. 6 - SSM A bicyclist travels in a circle of radius 25.0...Ch. 6 - In Fig. 6-57, a stuntman drives a car without...Ch. 6 - You must push a crate across a floor to a docking...Ch. 6 - In Fig. 6-58, force F is applied to a crate of...Ch. 6 - In the early afternoon, a car is parked on a...Ch. 6 - A sling-thrower puts a stone 0.250 kg in the...Ch. 6 - SSM A car weighing 10.7 kN and traveling at 13.4...Ch. 6 - In Fig. 6-59, block 1 of mass m1 = 2.0 kg and...Ch. 6 - SSM A filing cabinet weighing 556 N rests on the...Ch. 6 - In Fig. 6-60, a block weighing 22 N is held at...Ch. 6 - Prob. 91PCh. 6 - A circular curve of highway is designed for...Ch. 6 - A 1.5 kg box is initially at rest on a horizontal...Ch. 6 - A child weighing 140 N sits at rest at the top of...Ch. 6 - In Fig. 6-61 a fastidious worker pushes directly...Ch. 6 - A child places a picnic basket on the outer rim of...Ch. 6 - SSM A warehouse worker exerts a constant...Ch. 6 - In Fig. 6-62, a 5.0 kg block is sent sliding up a...Ch. 6 - An 11 kg block of steel is at rest on a horizontal...Ch. 6 - A ski that is placed on snow will stick to the...Ch. 6 - Playing near a road construction site, a child...Ch. 6 - A 100 N force, directed at an angle above a...Ch. 6 - A certain string can withstand a maximum tension...Ch. 6 - A four-person bobsled total mass = 630 kg comes...Ch. 6 - As a 40 N block slides down a plane that is...
Additional Science Textbook Solutions
Find more solutions based on key concepts
2. Whether an allele is dominant or recessive depends on
a. how common the allele is, relative to other alleles...
Campbell Biology: Concepts & Connections (9th Edition)
What are genomic imprinting and nondisjunction?
Principles of Anatomy and Physiology
A wind turbine can extract at most a fraction 16/27 of the wind kinetic energy. With a rotor diameter of 15 m a...
Fundamentals Of Thermodynamics
SCIENTIFIC INQUIRY You are handed a mystery pea plant with tall stems and axial flowers and asked to determine ...
Campbell Biology (11th Edition)
Explain all answers clearly, with complete sentences and proper essay structure if needed. An asterisk (*) desi...
Cosmic Perspective Fundamentals
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- (a) Imagine that a space probe could be fired as a projectile from the Earth's surface with an initial speed of 5.48 x 10 m/s relative to the Sun. What would its speed be when it is very far from the Earth (in m/s)? Ignore atmospheric friction, the effects of other planets, and the rotation of the Earth. (Consider the mass of the Sun in your calculations.) Your response differs from the correct answer by more than 10%. Double check your calculations. m/s (b) What If? The speed provided in part (a) is very difficult to achieve technologically. Often, Jupiter is used as a "gravitational slingshot" to increase the speed of a probe to the escape speed from the solar system, which is 1.85 x 10 m/s from a point on Jupiter's orbit around the Sun (if Jupiter is not nearby). If the probe is launched from the Earth's surface at a speed of 4.10 x 10 m/s relative to the Sun, what is the increase in speed needed from the gravitational slingshot at Jupiter for the space probe to escape the solar…arrow_forwardVery far from earth (at R=∞), a spacecraft has run out of fuel and its kinetic energy is zero. If only the gravitational force of the earth were to act on it (i.e., neglect the forces from the sun and other solar system objects), the spacecraft would eventually crash into the earth. The mass of the earth is Me and its radius is Re. Neglect air resistance throughout this problem, since the spacecraft is primarily moving through the near vacuum of space. Find the speed se of the spacecraft when it crashes into the earth. Express the speed in terms of Me, Re, and the universal gravitational constant G. Now find the spacecraft's speed when its distance from the center of the earth is R=αRe, where the coefficient α≥1. Express the speed in terms of se and α.arrow_forwardA cyclotron is a machine that can be used to accelerate charged particles to achieve large kinetic energies. The resulting beams of highly energetic particles then can be used for many medical applications, including Proton Therapy (a more precise form of "radiation" used in the treatment of some cancers). If a proton (of mass 1.673x10-27kg) is accelerated to its maximum velocity inside a dee with radius 4.46cm (this is the radius you would use for the "r" term in the centripetal acceleration), and if the magnetic field has a magnitude of 3.49x10-2T, what is the resulting velocity of the proton in units of km/s (kilometer per second)?arrow_forward
- When you look at the surface of a music CD, you see the colors of a rainbow. (a) Estimate the distance between the curved lines (to be read by the laser). (b) Estimate the distance between lines, noting that a CD contains at most 80 min of music, that it rotates at speeds from 200 to 500 rev/min, and that 2/3 of its 6-cm radius contains the lines.arrow_forwardAn accelerometer C is mounted to the side of the roller-coaster car and records a total acceleration of 3.9g as the empty car passes the bottommost position of the track as shown. If the speed of the car at this position is 190 km/h and is decreasing at the rate of 16 km/h every second, determine the radius of curvature p of the track at the position shown. Answer: p= i V Co- 3 0.96 m Varrow_forwardIf the magnitude of the force is 18 N to cause a 1 kg object to move in a circle of radius 2 m. The speed of the object is:arrow_forward
- . (II) In traveling to the Moon, astronauts aboard theApollo spacecraft put the spacecraft into a slow rotation todistribute the Sun’s energy evenly (so one side would notbecome too hot). At the start of their trip, they acceleratedfrom no rotation to 1.0 revolution every minute during a12-min time interval. Think of the spacecraft as a cylinderwith a diameter of 8.5 m rotating about its cylindrical axis.Determine (a) the angular acceleration, and (b) the radialand tangential components of the linear acceleration of apoint on the skin of the ship 6.0 min after it started thisaccelerationarrow_forwardAn asteroid, headed directly toward Earth, has a speed of 12 km/s relative to the planet when the asteroid is 10 Earth radii from Earth’s center. Neglecting the effects of Earth’s atmosphere on the asteroid, find the asteroid’s speed v when it reaches Earth’s surface.arrow_forward55-56. Ganymede, one of Jupiter's moons, is the largest moon in our solar system. It has a mass of 1.48 × 1023 kg and a radius of 2.63 × 10 m. A 15.0 kg lunar rover is on Ganymede's surface. 55. As the rover orbits Ganymede, it is observed that during a particular segment of time, its speed decreases. During this segment of time, the work done by gravity on the rover is: a positive b) negative c zero d) more info is needed to determine 56. Find Ganymede's escape speed (the minimum launch speed from Ganymede's surface such that the projectile never falls back). a) 2.39 km/s b) 2.74 km/s c) 3.09 km/s d) 3.44 km/s e) 3.79 km/sarrow_forward
- (a) Imagine that a space probe could be fired as a projectile from the Earth's surface with an initial speed of 5.34 x 104 m/s relative to the Sun. What would its speed be when it is very far from the Earth (in m/s)? Ignore atmospheric friction, the effects of other planets, and the rotation of the Earth. (Consider the mass of the Sun in your calculations.) m/s (b) What If? The speed provided in part (a) is very difficult to achieve technologically. Often, Jupiter is used as a "gravitational slingshot" to increase the speed of a probe to the escape speed from the solar system, which is 1.85 x 104 m/s from a point on Jupiter's orbit around the Sun (if Jupiter is not nearby). If the probe is launched from the Earth's surface at a speed of 4.10 x 104 m/s relative to the Sun, what is the increase in speed needed from the gravitational slingshot at Jupiter for the space probe to escape the solar system (in m/s)? (Assume that the Earth and the point on Jupiter's orbit lie along the same…arrow_forward(c) A small object was found to drop above the surface of a big planet with no initial velocity and it fell 13.5 m in 3 s. If the radius of the planet is 5.82 x 10° m, calculate the small object's acceleration during the fall and the mass of the big planet.arrow_forward*13-128. A rocket is in free-flight elliptical orbit around the planet Venus. Knowing that the periapsis and apoapsis of the orbit are 8 Mm and 26 Mm, respectively, determine (a) the speed of the rocket at point A', (b) the required speed it must attain at A just after braking so that it undergoes an 8-Mm free-flight circular orbit around Venus, and (c) the periods of both the circular and elliptical orbits. The mass of Venus is 0.816 times the mass of the earth. A' 8 Mm -18 Mmarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Gravitational Force (Physics Animation); Author: EarthPen;https://www.youtube.com/watch?v=pxp1Z91S5uQ;License: Standard YouTube License, CC-BY