Mechanics of Materials (10th Edition)
10th Edition
ISBN: 9780134319650
Author: Russell C. Hibbeler
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 3.7, Problem 3.29P
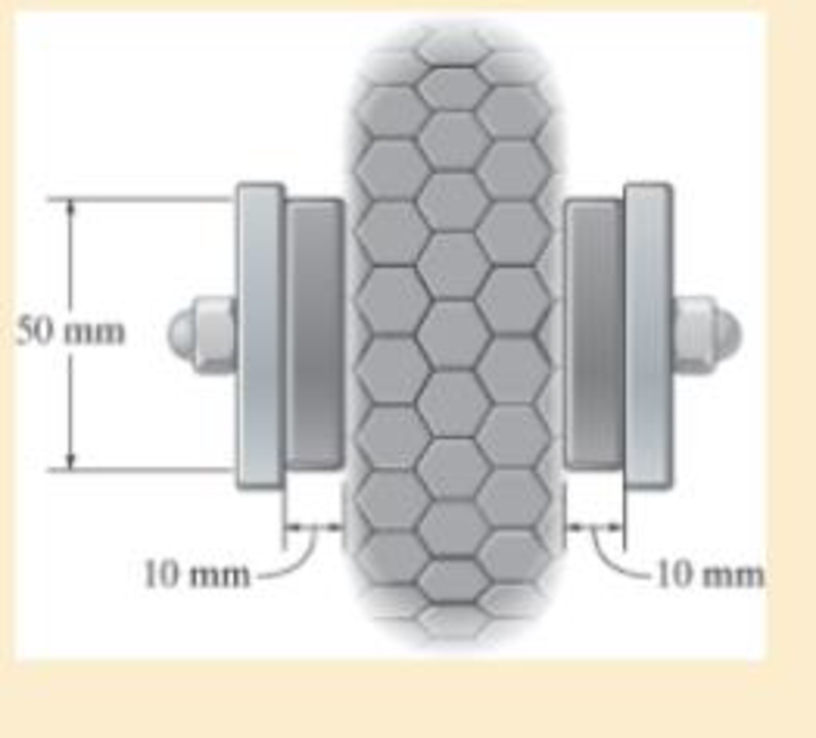
The brake pads for a bicycle tire are made of rubber. If a frictional force of 50 N is applied to each side of the tires, determine the average shear strain in the rubber. Each pad has cross-sectional dimensions of 20 mm and 50 mm. Gr = 0.20 MPa.
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
Determine the vertical displacement of plate A due to shear deformations in the rubber. The cross-sectional dimensions of each shoe are 35mm X 25mm. G = 0.3 Mpa,
The support consists of three rigid plates, which are connected
together using two symmetrically placed rubber pads as shown in
(Figure 1). Each pad has cross-sectional dimensions of 30 mm and
20 mm. G, = 0.20 MPa.
Part A
If a vertical force of P = 6 N is applied to plate A, determine the approximate vertical displacement of this plate due to shear strains in the rubber.
Express your answer to three significant figures and include the appropriate units.
µA
?
8 =
Value
mm
Submit
Request Answer
Figure
< 1 of 1
Provide Feedback
40 mm
40 mm
A
Determine the change in length, width and thickness of steel bar which is 4m long, 30mm wide
and 20mm thick and is subjected to an axial pull of 30 kN in the direction of length. Take E = 200
GPa and poison's ratio of 0.3. Also determine the volumetric strain and change in volume.
Chapter 3 Solutions
Mechanics of Materials (10th Edition)
Ch. 3.4 - Define a homogeneous material.Ch. 3.4 - Indicate the points on the stress-strain diagram...Ch. 3.4 - Define the modulus of elasticity E.Ch. 3.4 - At room temperature, mild steel is a ductile...Ch. 3.4 - Engineering stress and strain are calculated using...Ch. 3.4 - As the temperature increases the modulus of...Ch. 3.4 - A 100-mm-long rod has a diameter of 15 mm. If an...Ch. 3.4 - A bar has a length of 8 in. and cross-sectional...Ch. 3.4 - A 10-mm-diameter rod has a modulus of elasticity...Ch. 3.4 - The material for the 50-mm-long specimen has the...
Ch. 3.4 - The material for the 50-mm-long specimen has the...Ch. 3.4 - If the elongation of wire BC is 0.2 mm after the...Ch. 3.4 - A tension test was performed on a steel specimen...Ch. 3.4 - Data taken from a stress-strain test for a ceramic...Ch. 3.4 - Data taken from a stress-strain test for a ceramic...Ch. 3.4 - The stress-strain diagram for a steel alloy having...Ch. 3.4 - The stress-strain diagram for a steel alloy having...Ch. 3.4 - The stress-strain diagram for a steel alloy having...Ch. 3.4 - The rigid beam is supported by a pin at C and an...Ch. 3.4 - The rigid beam is supported by a pin at C and an...Ch. 3.4 - Acetal plastic has a stress-strain diagram as...Ch. 3.4 - The stress-strain diagram for an aluminum alloy...Ch. 3.4 - The stress-strain diagram for an aluminum alloy...Ch. 3.4 - The stress-strain diagram for an aluminum alloy...Ch. 3.4 - A bar having a length of 5 in. and cross-sectional...Ch. 3.4 - The rigid pipe is supported by a pin at A and an...Ch. 3.4 - The rigid pipe is supported by a pin at A and an...Ch. 3.4 - Direct tension indicators are sometimes used...Ch. 3.4 - The rigid beam is supported by a pin at C and an...Ch. 3.4 - The rigid beam is supported by a pin at C and an...Ch. 3.4 - The stress-strain diagram for a bone is shown, and...Ch. 3.4 - The stress-strain diagram for a bone is shown and...Ch. 3.4 - The two bars are made of a material that has the...Ch. 3.4 - The two bars are made of a material that has the...Ch. 3.4 - The pole is supported by a pin at C and an A-36...Ch. 3.4 - The bar DA is rigid and is originally held in the...Ch. 3.7 - A 100-mm-long rod has a diameter of 15 mm. If an...Ch. 3.7 - A solid circular rod that is 600 mm long and 20 mm...Ch. 3.7 - A 20-mm-wide block is firmly bonded to rigid...Ch. 3.7 - A 20-mm-wide block is bonded to rigid plates at...Ch. 3.7 - The acrylic plastic rod is 200 mm long and 15 mm...Ch. 3.7 - The plug has a diameter of 30 mm and fits within a...Ch. 3.7 - The elastic portion of the stress-strain diagram...Ch. 3.7 - The elastic portion of the stress-strain diagram...Ch. 3.7 - The brake pads for a bicycle tire are made of...Ch. 3.7 - The lap joint is connected together using a 1.25...Ch. 3.7 - The lap joint is connected together using a 1.25...Ch. 3.7 - The rubber block is subjected to an elongation of...Ch. 3.7 - The shear stress-strain diagram for an alloy is...Ch. 3.7 - A shear spring is made from two blocks of rubber,...Ch. 3 - The elastic portion of the tension stress-strain...Ch. 3 - The elastic portion of the tension stress-strain...Ch. 3 - The rigid beam rests in the horizontal position on...Ch. 3 - The wires each have a diameter of 12 in., length...Ch. 3 - The wires each have a diameter of 12 in., length...Ch. 3 - diameter steel bolts. If the clamping force in...Ch. 3 - The stress-strain diagram for polyethylene, which...Ch. 3 - The pipe with two rigid caps attached to its ends...Ch. 3 - The 8-mm-diameter bolt is made of an aluminum...Ch. 3 - An acetal polymer block is fixed to the rigid...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- The rivet group connects two narrow lengths of plate, one of which carries a 15 kN load. If the ultimate shear strength t of a rivet is 350 N/mm² and its failure strength in compression stress is 600 N/mm², determine the minimum allowable values of rivet diameter d (mm) and plate thickness t (mm).arrow_forwardA paper tube is formed by rolling a cardboard strip in a spiral and then gluing the edges together as shown. Determine the shear stress acting along the seam, which is at 50° from the horizontal, when the tube is subjected to an axial compressive force of 200 N. The paper is 2 mm thick and the tube has an outer diameter of 100 mm.arrow_forwardThe normal strain in a suspended bar of material of varying cross section due to its own weight is given by the expression γy/3E where γ = 2.9 lb/in.3 is the specific weight of the material, y = 3.4 in. is the distance from the free (i.e., bottom) end of the bar, L = 17 in. is the length of the bar, and E = 24000 ksi is a material constant. Determine, (a) the change in length of the bar due to its own weight. (b) the average normal strain over the length L of the bar. (c) the maximum normal strain in the bar.arrow_forward
- An air-filled rubber ball has a diameter of 6 in. If the air pressure within the ball is increased until the diameter becomes 7 in., determine the average normal strain in the rubber.arrow_forwardThe normal strain in a suspended bar of material of varying cross section due to its own weight is given by the expression yy/3E where y=2.4 lb/in.³ is the specific weight of the material, y = 0.6 in. is the distance from the free (i.e., bottom) end of the bar, L = 6 in. is the length of the bar, and E=30000 ksi is a material constant. Determine, (a) the change in length of the bar due to its own weight. (b) the average normal strain over the length L of the bar (c) the maximum normal strain in the bar. Answer: (a) 5 = 1 (b) Sa (c) Emax= i x10-6 in. με μεarrow_forwardAn air-filled rubber ball has a diameter of 150 mm. If the air pressure within it is increased until the ball’s diameter becomes 175 mm, determine the average normal strain in the rubber.arrow_forward
- The normal strain in a suspended bar of material of varying cross section due to its own weight is given by the expression yy/3E where y = 2.9 lb/in.³ is the specific weight of the material, y = 0.5 in. is the distance from the free (i.e., bottom) end of the bar, L = 5 in. is the length of the bar, and E = 25000 ksi is a material constant. Determine, (a) the change in length of the bar due to its own weight. (b) the average normal strain over the length L of the bar. (c) the maximum normal strain in the bar. Part 1 * Your answer is incorrect. Calculate the change in length of the bar due to its own weight. Answer: d= i 2.416 eTextbook and Media x10-6 in.arrow_forwardThe normal strain in a suspended bar of material of varying cross section due to its own weight is given by the expression yy/3E where y = 2.9 lb/in.³ is the specific weight of the material, y = 0.5 in. is the distance from the free (i.e., bottom) end of the bar, L = 5 in. is the length of the bar, and E = 25000 ksi is a material constant. Determine, (a) the change in length of the bar due to its own weight. (b) the average normal strain over the length L of the bar. (c) the maximum normal strain in the bar.arrow_forwardThe normal strain in a suspended bar of material of varying cross section due to its own weight is given by the expression yy/3E where y = 2.0 lb/in.³ is the specific weight of the material, y = 2.8 in. is the distance from the free (i.e., bottom) end of the bar, L = 14 in. is the length of the bar, and E = 20000 ksi is a material constant. Determine, (a) the change in length of the bar due to its own weight. (b) the average normal strain over the length L of the bar (c) the maximum normal strain in the bar. Answer: (a) 8- i (b) Eavg (c) Emax = i x10-6 in. με μεarrow_forward
- The normal strain in a suspended bar of material of varying cross section due to its own weight is given by the expression yy/3E where y = 3.0 lb/in.³ is the specific weight of the material, y = 6.3 in. is the distance from the free (i.e., bottom) end of the bar, L = 21 in. is the length of the bar, and E = 27000 ksi is a material constant. Determine, (a) the change in length of the bar due to its own weight. (b) the average normal strain over the length L of the bar (c) the maximum normal strain in the bar. Answer: (a) d = i (b) avg (c) Emax i x10-6 in. με μεarrow_forwardPart of a control linkage for an airplane consists of a rigid member CB and a flexible cable AB. If a force is applied to the end B of the member and causes a normal strain in the cable of 0.004 mm>mm, determine the displacement of point B. Originally the cable is unstretched.arrow_forwardThe normal strain in a suspended bar of material of varying cross section due to its own weight is given by the expression yy/3E wherey= 2.2 lb/in.³ is the specific weight of the material, y = 5.0 in. is the distance from the free (i.e., bottom) end of the bar, L = 25 in. is the length of the bar, and E = 23000 ksi is a material constant. Determine, (a) the change in length of the bar due to its own weight. (b) the average normal strain over the length of the bar (c) the maximum normal strain in the bar. Answer: (a) ō = i (b) Eave = i (c) Emax = i x10-in. με μεarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
Mechanical Design (Machine Design) Clutches, Brakes and Flywheels Intro (S20 ME470 Class 15); Author: Professor Ted Diehl;https://www.youtube.com/watch?v=eMvbePrsT34;License: Standard Youtube License