Chemistry: Atoms First
Chemistry: Atoms First
2nd Edition
ISBN: 9780073511184
Author: Julia Burdge, Jason Overby Professor
Publisher: McGraw-Hill Education
bartleby

Videos

Question
Book Icon
Chapter 3, Problem 3.89QP

(a)

Interpretation Introduction

Interpretation:

The orbital which is higher in energy should be identified in the given pairs of hydrogen orbitals.

Concept Introduction:

The energies of orbitals in the hydrogen atom depend on the value of the principal quantum number (n).  When n increases, energy also increases.  For this reason, orbitals in the same shell have the same energy in spite of their subshell.  The increasing order of energy of hydrogen orbitals is

1s < 2s = 2p < 3s = 3p = 3d < 4s = 4p = 4d = 4f

In the case of one 2s and three 2p orbitals in the second shell, they have the same energy.  In the third shell, all nine orbitals (one 3s, three 3p and five 3d) have the same energy.  All sixteen orbitals (one 4s, three 4p, five 4d and seven 4f) in the fourth shell have the same energy.

Chemistry: Atoms First, Chapter 3, Problem 3.89QP , additional homework tip 1

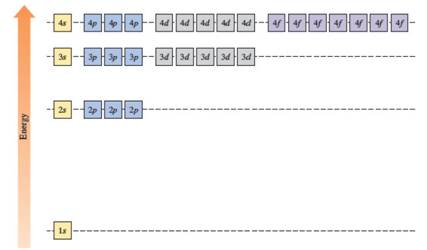
The energy levels of the different orbitals in hydrogen atom are easily explained by considering the given diagram.  Here, each box represents one orbital. Orbitals with the same principal quantum number (n) have the same energy.

Principal Quantum Number (n)

The principal quantum number (n) assigns the size of the orbital and specifies the energy of an electron.  If the value of n is larger, then the average distance of an electron in the orbital from the nucleus will be greater.  Therefore the size of the orbital is large. The principal quantum numbers have the integral values of 1, 2, 3 and so forth and it corresponds to the quantum number in Bohr’s model of the hydrogen atom.  If all orbitals have the same value of ‘n’, they are said to be in the same shell (level).  The total number of orbitals for a given n value is n2.  As the value of ‘n’ increases, the energy of the electron also increases.

To find: Identify the orbital which is higher in energy in the given pair 1s, 2s orbitals of hydrogen.

Find the value of ‘n’

(b)

Interpretation Introduction

Interpretation:

The orbital which is higher in energy should be identified in the given pairs of hydrogen orbitals.

Concept Introduction:

The energies of orbitals in the hydrogen atom depend on the value of the principal quantum number (n).  When n increases, energy also increases.  For this reason, orbitals in the same shell have the same energy in spite of their subshell.  The increasing order of energy of hydrogen orbitals is

1s < 2s = 2p < 3s = 3p = 3d < 4s = 4p = 4d = 4f

In the case of one 2s and three 2p orbitals in the second shell, they have the same energy.  In the third shell, all nine orbitals (one 3s, three 3p and five 3d) have the same energy.  All sixteen orbitals (one 4s, three 4p, five 4d and seven 4f) in the fourth shell have the same energy.

Chemistry: Atoms First, Chapter 3, Problem 3.89QP , additional homework tip 2

The energy levels of the different orbitals in hydrogen atom are easily explained by considering the given diagram.  Here, each box represents one orbital. Orbitals with the same principal quantum number (n) have the same energy.

Principal Quantum Number (n)

The principal quantum number (n) assigns the size of the orbital and specifies the energy of an electron.  If the value of n is larger, then the average distance of an electron in the orbital from the nucleus will be greater.  Therefore the size of the orbital is large. The principal quantum numbers have the integral values of 1, 2, 3 and so forth and it corresponds to the quantum number in Bohr’s model of the hydrogen atom.  If all orbitals have the same value of ‘n’, they are said to be in the same shell (level).  The total number of orbitals for a given n value is n2.  As the value of ‘n’ increases, the energy of the electron also increases.

To find: Identify the orbital which is higher in energy in the given pair 2p, 3p orbitals of hydrogen

Find the value of ‘n’

(c)

Interpretation Introduction

Interpretation:

The orbital which is higher in energy should be identified in the given pairs of hydrogen orbitals.

Concept Introduction:

The energies of orbitals in the hydrogen atom depend on the value of the principal quantum number (n).  When n increases, energy also increases.  For this reason, orbitals in the same shell have the same energy in spite of their subshell.  The increasing order of energy of hydrogen orbitals is

1s < 2s = 2p < 3s = 3p = 3d < 4s = 4p = 4d = 4f

In the case of one 2s and three 2p orbitals in the second shell, they have the same energy.  In the third shell, all nine orbitals (one 3s, three 3p and five 3d) have the same energy.  All sixteen orbitals (one 4s, three 4p, five 4d and seven 4f) in the fourth shell have the same energy.

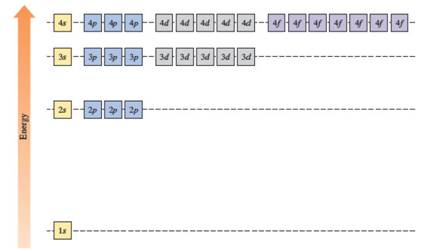
Chemistry: Atoms First, Chapter 3, Problem 3.89QP , additional homework tip 3

The energy levels of the different orbitals in hydrogen atom are easily explained by considering the given diagram.  Here, each box represents one orbital. Orbitals with the same principal quantum number (n) have the same energy.

Principal Quantum Number (n)

The principal quantum number (n) assigns the size of the orbital and specifies the energy of an electron.  If the value of n is larger, then the average distance of an electron in the orbital from the nucleus will be greater.  Therefore the size of the orbital is large. The principal quantum numbers have the integral values of 1, 2, 3 and so forth and it corresponds to the quantum number in Bohr’s model of the hydrogen atom.  If all orbitals have the same value of ‘n’, they are said to be in the same shell (level).  The total number of orbitals for a given n value is n2.  As the value of ‘n’ increases, the energy of the electron also increases.

To find: Identify the orbital which is higher in energy in the given pair 3dxy, 3dyz orbitals of hydrogen

Find the value of ‘n’

(d)

Interpretation Introduction

Interpretation:

The orbital which is higher in energy should be identified in the given pairs of hydrogen orbitals.

Concept Introduction:

The energies of orbitals in the hydrogen atom depend on the value of the principal quantum number (n).  When n increases, energy also increases.  For this reason, orbitals in the same shell have the same energy in spite of their subshell.  The increasing order of energy of hydrogen orbitals is

1s < 2s = 2p < 3s = 3p = 3d < 4s = 4p = 4d = 4f

In the case of one 2s and three 2p orbitals in the second shell, they have the same energy.  In the third shell, all nine orbitals (one 3s, three 3p and five 3d) have the same energy.  All sixteen orbitals (one 4s, three 4p, five 4d and seven 4f) in the fourth shell have the same energy.

Chemistry: Atoms First, Chapter 3, Problem 3.89QP , additional homework tip 4

The energy levels of the different orbitals in hydrogen atom are easily explained by considering the given diagram.  Here, each box represents one orbital. Orbitals with the same principal quantum number (n) have the same energy.

Principal Quantum Number (n)

The principal quantum number (n) assigns the size of the orbital and specifies the energy of an electron.  If the value of n is larger, then the average distance of an electron in the orbital from the nucleus will be greater.  Therefore the size of the orbital is large. The principal quantum numbers have the integral values of 1, 2, 3 and so forth and it corresponds to the quantum number in Bohr’s model of the hydrogen atom.  If all orbitals have the same value of ‘n’, they are said to be in the same shell (level).  The total number of orbitals for a given n value is n2.  As the value of ‘n’ increases, the energy of the electron also increases.

To find: Identify the orbital which is higher in energy in the given pair 3s, 3d orbitals of hydrogen

Find the value of ‘n’

(e)

Interpretation Introduction

Interpretation:

The orbital which is higher in energy should be identified in the given pairs of hydrogen orbitals.

Concept Introduction:

The energies of orbitals in the hydrogen atom depend on the value of the principal quantum number (n).  When n increases, energy also increases.  For this reason, orbitals in the same shell have the same energy in spite of their subshell.  The increasing order of energy of hydrogen orbitals is

1s < 2s = 2p < 3s = 3p = 3d < 4s = 4p = 4d = 4f

In the case of one 2s and three 2p orbitals in the second shell, they have the same energy.  In the third shell, all nine orbitals (one 3s, three 3p and five 3d) have the same energy.  All sixteen orbitals (one 4s, three 4p, five 4d and seven 4f) in the fourth shell have the same energy.

Chemistry: Atoms First, Chapter 3, Problem 3.89QP , additional homework tip 5

The energy levels of the different orbitals in hydrogen atom are easily explained by considering the given diagram.  Here, each box represents one orbital. Orbitals with the same principal quantum number (n) have the same energy.

Principal Quantum Number (n)

The principal quantum number (n) assigns the size of the orbital and specifies the energy of an electron.  If the value of n is larger, then the average distance of an electron in the orbital from the nucleus will be greater.  Therefore the size of the orbital is large. The principal quantum numbers have the integral values of 1, 2, 3 and so forth and it corresponds to the quantum number in Bohr’s model of the hydrogen atom.  If all orbitals have the same value of ‘n’, they are said to be in the same shell (level).  The total number of orbitals for a given n value is n2.  As the value of ‘n’ increases, the energy of the electron also increases.

To find: Identify the orbital which is higher in energy in the given pair 4f, 5s orbitals of hydrogen

Find the value of ‘n’

Expert Solution & Answer
Check Mark

Want to see the full answer?

Check out a sample textbook solution
Blurred answer
Previous
Chapter 3, Problem 3.88QP
Next
Chapter 3, Problem 3.90QP
Students have asked these similar questions
Assign theses carbons
Assign these proton
Could you please solve the first problem in this way and present it similarly but color-coded or step by step so I can understand it better? Thank you!

Chapter 3 Solutions

Chemistry: Atoms First

Ch. 3.1 - Calculate the kinetic energy of a helium atom...Ch. 3.1 - Calculate the energy in joules of a 5.25-g object...Ch. 3.1 - Prob. 1PPBCh. 3.1 - Prob. 1PPCCh. 3.1 - Prob. 3.2WECh. 3.1 - How much greater is the electrostatic potential...Ch. 3.1 - What must the separation between charges of +2 and...Ch. 3.1 - Prob. 2PPCCh. 3.1 - Prob. 3.1.1SRCh. 3.1 - Prob. 3.1.2SR
Ch. 3.1 - Prob. 3.1.3SRCh. 3.2 - One type of laser used in the treatment of...Ch. 3.2 - What is the wavelength (in meters) of an...Ch. 3.2 - What is the frequency (in reciprocal seconds) of...Ch. 3.2 - Which of the following sets of waves best...Ch. 3.2 - Prob. 3.2.1SRCh. 3.2 - Prob. 3.2.2SRCh. 3.2 - Prob. 3.2.3SRCh. 3.2 - Prob. 3.2.4SRCh. 3.3 - Calculate the energy (in joules) of (a) a photon...Ch. 3.3 - Calculate the energy (in joules) of (a) a photon...Ch. 3.3 - (a) Calculate the wavelength (in nanometers) of...Ch. 3.3 - Prob. 3.3.1SRCh. 3.3 - Prob. 3.3.2SRCh. 3.3 - Prob. 3.3.3SRCh. 3.3 - Prob. 3.3.4SRCh. 3.3 - Prob. 3.3.5SRCh. 3.4 - Calculate the wavelength (in nanometers) of the...Ch. 3.4 - What is the wavelength (in nanometers) of a photon...Ch. 3.4 - What is the value of ni for an electron that emits...Ch. 3.4 - For each pair of transitions, determine which one...Ch. 3.4 - Prob. 3.4.1SRCh. 3.4 - Prob. 3.4.2SRCh. 3.4 - Prob. 3.4.3SRCh. 3.4 - Prob. 3.4.4SRCh. 3.5 - Calculate the de Broglie wavelength of the...Ch. 3.5 - Calculate the de Broglie wavelength (in...Ch. 3.5 - Use Equation 3.11 to calculate the momentum, p...Ch. 3.5 - Consider the impact of early electron diffraction...Ch. 3.5 - Prob. 3.5.1SRCh. 3.5 - Prob. 3.5.2SRCh. 3.5 - Prob. 3.5.3SRCh. 3.6 - An electron in a hydrogen atom is known to have a...Ch. 3.6 - Prob. 7PPACh. 3.6 - (a) Calculate the minimum uncertainty in the...Ch. 3.6 - Using Equation 3.13, we can calculate the minimum...Ch. 3.6 - Prob. 3.6.1SRCh. 3.6 - Prob. 3.6.2SRCh. 3.7 - What are the possible values for the magnetic...Ch. 3.7 - Prob. 8PPACh. 3.7 - Prob. 8PPBCh. 3.7 - Prob. 8PPCCh. 3.7 - Prob. 3.7.1SRCh. 3.7 - Prob. 3.7.2SRCh. 3.7 - Prob. 3.7.3SRCh. 3.7 - Prob. 3.7.4SRCh. 3.8 - Prob. 3.9WECh. 3.8 - Prob. 9PPACh. 3.8 - Prob. 9PPBCh. 3.8 - Prob. 9PPCCh. 3.8 - Prob. 3.8.1SRCh. 3.8 - Prob. 3.8.2SRCh. 3.8 - Prob. 3.8.3SRCh. 3.8 - Prob. 3.8.4SRCh. 3.9 - Write the electron configuration and give the...Ch. 3.9 - Prob. 10PPACh. 3.9 - Write the electron configuration and give the...Ch. 3.9 - Prob. 10PPCCh. 3.9 - Prob. 3.9.1SRCh. 3.9 - Prob. 3.9.2SRCh. 3.9 - Prob. 3.9.3SRCh. 3.10 - Without referring to Figure 3.26, write the...Ch. 3.10 - Prob. 11PPACh. 3.10 - Prob. 11PPBCh. 3.10 - Consider again the alternate universe and its...Ch. 3.10 - Prob. 3.10.1SRCh. 3.10 - Prob. 3.10.2SRCh. 3.10 - Prob. 3.10.3SRCh. 3.10 - Prob. 3.10.4SRCh. 3 - Define these terms: potential energy, kinetic...Ch. 3 - What are the units for energy commonly employed in...Ch. 3 - A truck initially traveling at 60 km/h is brought...Ch. 3 - Describe the interconversions of forms of energy...Ch. 3 - Prob. 3.5QPCh. 3 - Prob. 3.6QPCh. 3 - Prob. 3.7QPCh. 3 - Prob. 3.8QPCh. 3 - Prob. 3.9QPCh. 3 - (a) How much greater is the electrostatic energy...Ch. 3 - Prob. 3.11QPCh. 3 - Prob. 3.12QPCh. 3 - List the types of electromagnetic radiation,...Ch. 3 - Prob. 3.14QPCh. 3 - Prob. 3.15QPCh. 3 - Prob. 3.16QPCh. 3 - The SI unit of time is the second, which is...Ch. 3 - Prob. 3.18QPCh. 3 - Prob. 3.19QPCh. 3 - Four waves represent light in four different...Ch. 3 - Prob. 3.21QPCh. 3 - Prob. 3.22QPCh. 3 - Prob. 3.23QPCh. 3 - What is a photon? What role did Einsteins...Ch. 3 - A photon has a wavelength of 705 nm. Calculate the...Ch. 3 - The blue color of the sky results from the...Ch. 3 - A photon has a frequency of 6.5 109 Hz. (a)...Ch. 3 - Prob. 3.28QPCh. 3 - Prob. 3.29QPCh. 3 - Prob. 3.30QPCh. 3 - Prob. 3.31QPCh. 3 - A particular form of electromagnetic radiation has...Ch. 3 - Photosynthesis makes use of visible light to bring...Ch. 3 - The retina of a human eye can detect light when...Ch. 3 - Prob. 3.35QPCh. 3 - The binding energy of magnesium metal is 5.86 ...Ch. 3 - What is the kinetic energy of the ejected electron...Ch. 3 - A red light was shined onto a metal sample and the...Ch. 3 - A photoelectric experiment was performed by...Ch. 3 - Which of the following best explains why we see...Ch. 3 - One way to see the emission spectrum of hydrogen...Ch. 3 - How many lines would we see in the emission...Ch. 3 - For a hydrogen atom in which the electron has been...Ch. 3 - Prob. 3.40QPCh. 3 - Prob. 3.41QPCh. 3 - Briefly describe Bohrs theory of the hydrogen atom...Ch. 3 - Explain the meaning of the negative sign in...Ch. 3 - Consider the following energy levels of a...Ch. 3 - Prob. 3.45QPCh. 3 - Calculate the wavelength (in nanometers) of a...Ch. 3 - Calculate the frequency (hertz) and wavelength...Ch. 3 - What wavelength of light is needed to excite the...Ch. 3 - An electron in the hydrogen atom makes a...Ch. 3 - Explain why elements produce their own...Ch. 3 - Some copper-containing substances emit green light...Ch. 3 - Prob. 3.52QPCh. 3 - Prob. 3.53QPCh. 3 - Prob. 3.54QPCh. 3 - Why is Equation 3.11 meaningful only for...Ch. 3 - Prob. 3.56QPCh. 3 - Thermal neutrons are neutrons that move at speeds...Ch. 3 - Protons can be accelerated to speeds near that of...Ch. 3 - Prob. 3.59QPCh. 3 - Prob. 3.60QPCh. 3 - Prob. 3.61QPCh. 3 - Prob. 3.62QPCh. 3 - What are the inadequacies of Bohrs theory?Ch. 3 - What is the Heisenberg uncertainty principle? What...Ch. 3 - Prob. 3.65QPCh. 3 - Prob. 3.66QPCh. 3 - Prob. 3.67QPCh. 3 - The speed of a thermal neutron (see Problem 3.57)...Ch. 3 - Alveoli are tiny sacs of air in the lungs. Their...Ch. 3 - In the beginning of the twentieth century, some...Ch. 3 - Suppose that photons of blue light (430 nm) are...Ch. 3 - Prob. 3.72QPCh. 3 - Prob. 3.73QPCh. 3 - Which of the four quantum numbers (n, , m, ms)...Ch. 3 - Prob. 3.75QPCh. 3 - Prob. 3.76QPCh. 3 - Prob. 3.77QPCh. 3 - Prob. 3.78QPCh. 3 - Describe the shapes of s, p, and d orbitals. How...Ch. 3 - Prob. 3.80QPCh. 3 - Describe the characteristics of an s orbital, p...Ch. 3 - Why is a boundary surface diagram useful in...Ch. 3 - Prob. 3.83QPCh. 3 - Give the values of the four quantum numbers of an...Ch. 3 - Describe how a 1s orbital and a 2s orbital are...Ch. 3 - Prob. 3.86QPCh. 3 - Prob. 3.87QPCh. 3 - Make a chart of all allowable orbitals in the...Ch. 3 - Prob. 3.89QPCh. 3 - Prob. 3.90QPCh. 3 - A 3s orbital is illustrated here. Using this as a...Ch. 3 - Prob. 3.92QPCh. 3 - Prob. 3.93QPCh. 3 - State the Aufbau principle, and explain the role...Ch. 3 - Indicate the total number of (a) p electrons in N...Ch. 3 - Calculate the total number of electrons that can...Ch. 3 - Determine the total number of electrons that can...Ch. 3 - Determine the maximum number of electrons that can...Ch. 3 - Prob. 3.99QPCh. 3 - The electron configuration of an atom in the...Ch. 3 - List the following atoms in order of increasing...Ch. 3 - Determine the number of unpaired electrons in each...Ch. 3 - Determine the number of impaired electrons in each...Ch. 3 - Determine the number of unpaired electrons in each...Ch. 3 - Prob. 3.105QPCh. 3 - Portions of orbital diagrams representing the...Ch. 3 - Prob. 3.107QPCh. 3 - Prob. 3.108QPCh. 3 - Prob. 3.109QPCh. 3 - Define the following terms and give an example of...Ch. 3 - Explain why the ground-state electron...Ch. 3 - Write the election configuration of a xenon core.Ch. 3 - Comment on the correctness of the following...Ch. 3 - Prob. 3.114QPCh. 3 - Prob. 3.115QPCh. 3 - Write the ground-state electron configurations for...Ch. 3 - Write the ground-state electron configurations for...Ch. 3 - What is the symbol of the element with the...Ch. 3 - Prob. 3.119QPCh. 3 - Prob. 3.120QPCh. 3 - Discuss the current view of the correctness of the...Ch. 3 - Distinguish carefully between the following terms:...Ch. 3 - What is the maximum number of electrons in an atom...Ch. 3 - Prob. 3.124QPCh. 3 - Prob. 3.125QPCh. 3 - A baseball pitchers fastball has been clocked at...Ch. 3 - A ruby laser produces radiation of wavelength 633...Ch. 3 - Four atomic energy levels of an atom are shown...Ch. 3 - Prob. 3.129QPCh. 3 - Spectral lines of the Lyman and Balmer series do...Ch. 3 - Only a fraction of the electric energy supplied to...Ch. 3 - The figure here illustrates a series of...Ch. 3 - When one of heliums electrons is removed, the...Ch. 3 - The retina of a human eye can detect light when...Ch. 3 - An electron in an excited state in a hydrogen atom...Ch. 3 - Prob. 3.136QPCh. 3 - The election configurations described in this...Ch. 3 - Draw the shapes (boundary surfaces) of the...Ch. 3 - Prob. 3.139QPCh. 3 - Consider the graph here. (a) Calculate the binding...Ch. 3 - Scientists have found interstellar hydrogen atoms...Ch. 3 - Ionization energy is the minimum energy required...Ch. 3 - Prob. 3.143QPCh. 3 - Prob. 3.144QPCh. 3 - The cone cells of the human eye are sensitive to...Ch. 3 - (a) An electron in the ground state of the...Ch. 3 - Prob. 3.147QPCh. 3 - Prob. 3.148QPCh. 3 - When an election makes a transition between energy...Ch. 3 - Blackbody radiation is the term used to describe...Ch. 3 - Suppose that photons of red light (675 nm) are...Ch. 3 - In an election microscope, electrons are...Ch. 3 - According to Einsteins special theory of...Ch. 3 - The mathematical equation for studying the...
Knowledge Booster
Background pattern image
Chemistry
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, chemistry and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
  • Text book image
    Chemistry for Engineering Students
    Chemistry
    ISBN:9781337398909
    Author:Lawrence S. Brown, Tom Holme
    Publisher:Cengage Learning
    Text book image
    Chemistry: The Molecular Science
    Chemistry
    ISBN:9781285199047
    Author:John W. Moore, Conrad L. Stanitski
    Publisher:Cengage Learning
    Text book image
    General Chemistry - Standalone book (MindTap Cour...
    Chemistry
    ISBN:9781305580343
    Author:Steven D. Gammon, Ebbing, Darrell Ebbing, Steven D., Darrell; Gammon, Darrell Ebbing; Steven D. Gammon, Darrell D.; Gammon, Ebbing; Steven D. Gammon; Darrell
    Publisher:Cengage Learning
  • Text book image
    Chemistry
    Chemistry
    ISBN:9781133611097
    Author:Steven S. Zumdahl
    Publisher:Cengage Learning
    Text book image
    Chemistry
    Chemistry
    ISBN:9781305957404
    Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCoste
    Publisher:Cengage Learning
    Text book image
    Chemistry: An Atoms First Approach
    Chemistry
    ISBN:9781305079243
    Author:Steven S. Zumdahl, Susan A. Zumdahl
    Publisher:Cengage Learning
Text book image
Chemistry for Engineering Students
Chemistry
ISBN:9781337398909
Author:Lawrence S. Brown, Tom Holme
Publisher:Cengage Learning
Text book image
Chemistry: The Molecular Science
Chemistry
ISBN:9781285199047
Author:John W. Moore, Conrad L. Stanitski
Publisher:Cengage Learning
Text book image
General Chemistry - Standalone book (MindTap Cour...
Chemistry
ISBN:9781305580343
Author:Steven D. Gammon, Ebbing, Darrell Ebbing, Steven D., Darrell; Gammon, Darrell Ebbing; Steven D. Gammon, Darrell D.; Gammon, Ebbing; Steven D. Gammon; Darrell
Publisher:Cengage Learning
Text book image
Chemistry
Chemistry
ISBN:9781133611097
Author:Steven S. Zumdahl
Publisher:Cengage Learning
Text book image
Chemistry
Chemistry
ISBN:9781305957404
Author:Steven S. Zumdahl, Susan A. Zumdahl, Donald J. DeCoste
Publisher:Cengage Learning
Text book image
Chemistry: An Atoms First Approach
Chemistry
ISBN:9781305079243
Author:Steven S. Zumdahl, Susan A. Zumdahl
Publisher:Cengage Learning
SEE MORE TEXTBOOKS
Quantum Numbers, Atomic Orbitals, and Electron Configurations; Author: Professor Dave Explains;https://www.youtube.com/watch?v=Aoi4j8es4gQ;License: Standard YouTube License, CC-BY
QUANTUM MECHANICAL MODEL/Atomic Structure-21E; Author: H to O Chemistry;https://www.youtube.com/watch?v=mYHNUy5hPQE;License: Standard YouTube License, CC-BY