Chapter 11.10, Problem 112RP

An air conditioner operates on the vapor-compression refrigeration cycle with refrigerant-134a as the refrigerant. The air conditioner is used to keep a space at 21°C while rejecting the waste heat to the ambient air at 37°C. The refrigerant enters the compressor at 180 kPa superheated by 2.7°C at a rate of 0.06 kg/s and leaves the compressor at 1200 kPa and 60°C. R-134a is subcooled by 6.3°C at the exit of the condenser. Determine (a) the rate of cooling provided to the space, in Btu/h, and the COP, (b) the isentropic efficiency and the exergy efficiency of the compressor, (c) the exergy destruction in each component of the cycle and the total exergy destruction in the cycle, and (d) the minimum power input and the second-law efficiency of the cycle.

To determine

The rate of cooling provided to the space.

The coefficient of performance of the air conditioner.

Answer to Problem 112RP

The rate of cooling provided to the space is $\underset{\_}{\text{28,020}\text{Btu/h}}$.

The coefficient of performance of the air conditioner is $\underset{\_}{3.076}$.

Explanation of Solution

Show the T-s diagram as in Figure (1).

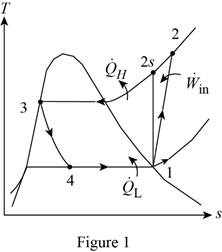
Express the refrigeration load.

${\dot{Q}}_L=\dot{m}\left(h_1-h_4\right)$ (I)

Here, specific enthalpy at state 1 and 4 is $h_1$ and $h_4$ and mass flow rate of refrigerant is $\dot{m}$.

Express the rate of work input.

${\dot{W}}_{\text{in}}=\dot{m}\left(h_2-h_1\right)$ (II)

Express the coefficient of performance of the air conditioner.

$\text{COP}=\frac{{\dot{Q}}_L}{{\dot{W}}_{\text{in}}}$ (III)

Conclusion:

Perform the unit conversion of pressure at state 1 from $\text{kPa}\text{to}\text{MPa}$.

$\begin{array}{c}{P}_1=180\text{kPa}\\ =180\text{kPa}\left[\frac{\text{MPa}}{1000\text{kPa}}\right]\\ =0.18\text{MPa}\end{array}$

Refer to Table A-13, obtain the saturation temperature at a pressure of 0.18 MPa as $-12.7°\text{C}$.

Calculate the temperature at state 1.

$T_1=T_{\text{sat}@180\text{kPa}}+2.7°\text{C}$

Substitute $-12.7°\text{C}$ for $T_{\text{sat@}0.18\text{MPa}}$.

$\begin{array}{c}{T}_1=-12.7°\text{C}+2.7°\text{C}\\ =-10.0°\text{C}\end{array}$

Refer Table A-13, “superheated refrigerant-134a”, and write the properties corresponding to pressure and inlet temperature of $\text{0}\text{.18}\text{MPa}$ and $-10.0°\text{C}$.

$\begin{array}{l}{h}_1=245.15\text{kJ}/\text{kg}\\ s_1=0.9484\text{kJ}/\text{kg}\cdot \text{K}\end{array}$

Here, specific enthalpy and entropy is $h_1\text{and}{s}_1$ respectively.

Write the formula of interpolation method of two variables.

$y_2=\frac{\left(x_2-x_1\right)\left(y_3-y_1\right)}{\left(x_3-x_1\right)}+y_1$ (IV)

Here, the variables denote by x and y is specific entropy at state 2 and specific enthalpy at state 2s respectively.

Show the specific enthalpy at state 2s corresponding to specific entropy by referring the table A-13 at pressure of 1.2 MPa and specific entropy of $\left(s_1=s_2=0.9484\text{kJ}/\text{kg}\cdot \text{K}\right)$ using interpolation method from Equation (IV) as in Table (1).

Specific entropy at state 2 $s_2\left(\text{kJ}/\text{kg}\cdot \text{K}\right)$	Specific enthalpy at state 2s $h_{2s}\left(\text{kJ}/\text{kg}\right)$
0.9268 $\left(x_1\right)$	278.28 $\left(y_1\right)$
0.9484 $\left(x_2\right)$	$\left(y_2=?\right)$
0.9615 $\left(x_3\right)$	289.66 $\left(y_3\right)$

Substitute the Table (1) values in Equation (IV) to get 285.34 kJ/kg.

Thus, the specific enthalpy at state 2s is,

$h_{2s}=285.34\text{kJ}/\text{kg}$

Refer Table A-13, “superheated refrigerant-134a”, and write the properties corresponding to exit state of pressure and temperature of $1.2\text{MPa}$ and $60°\text{C}$.

$\begin{array}{l}{h}_2=289.66\text{kJ}/\text{kg}\\ s_2=0.9615\text{kJ}/\text{kg}\cdot \text{K}\end{array}$

Here, specific enthalpy and entropy at exit state are $h_2\text{and}{s}_2$ respectively.

Refer to Table A-13, obtain the saturation temperature at a pressure of 1.2 MPa as $46.3°\text{C}$.

Refer Table A-13, “superheated refrigerant-134a”, and write the properties corresponding to state 3 of pressure and temperature of $1.2\text{MPa}$ and $\left(T_3=\left(46.3-6.3\right)°\text{C}=40°\text{C}\right)$.

$\begin{array}{l}{h}_3=h_{f@40°\text{C}}=108.27\text{kJ}/\text{kg}\\ s_3=s_{f@40°\text{C}}=0.3949\text{kJ}/\text{kg}\cdot \text{K}\end{array}$

Here, specific enthalpy and entropy at state 3 are $h_3\text{and}{s}_3$ respectively.

Refer Table A-13, “superheated refrigerant-134a”, and write the properties corresponding to state 4 of pressure and specific enthalpy of $0.18\text{MPa}$ and $\left(h_4=h_3=108.26\text{kJ/kg}\right)$.

$s_4=0.4229\text{kJ}/\text{kg}\cdot \text{K}$

Substitute 0.06 kg/s for $\dot{m}$, 245.15 kJ/kg for $h_1$, and 108.27 kJ/kg for $h_4$ in Equation (I).

$\begin{array}{c}{\dot{Q}}_L=0.06\text{kg/s}\left(\text{245}\text{.15}\text{kJ/kg}-108.27\text{kJ/kg}\right)\\ =8.213\frac{\text{kJ}}{\text{s}}\times \frac{\text{1}\text{kW}}{\left(\frac{\text{kJ}}{\text{s}}\right)}\\ =8.213\text{kW}\times \frac{\text{3412}\text{Btu/h}}{1\text{kW}}\\ =28,020\text{Btu/h}\end{array}$

Thus, the rate of cooling provided to the space is $\underset{\_}{\text{28,020}\text{Btu/h}}$.

Substitute 0.06 kg/s for $\dot{m}$, 245.15 kJ/kg for $h_1$, and 289.66 kJ/kg for $h_2$ in Equation (II).

$\begin{array}{c}{\dot{W}}_{\text{in}}=0.06\text{kg}/\text{s}\left(289.66\text{kJ/kg}-245.15\text{kJ/kg}\right)\\ =2.670\text{kg}/\text{s}\times \frac{\text{1}\text{kW}}{\text{kg}/\text{s}}\\ =2.67\text{0}\text{kW}\end{array}$

Substitute 2.670 kW for ${\dot{W}}_{\text{in}}$ and $8.213\text{kW}$ for ${\dot{Q}}_L$ in Equation (III).

$\begin{array}{c}\text{COP}=\frac{8.213\text{kW}}{2.670\text{kW}}\\ =3.076\end{array}$

Thus, the coefficient of performance of the air conditioner is $\underset{\_}{3.076}$.

To determine

The isentropic efficiency of the compressor.

The exergy efficiency of the compressor.

Answer to Problem 112RP

The isentropic efficiency of the compressor is $\underset{\_}{90.3\%}$.

The exergy efficiency of the compressor is $\underset{\_}{90.9\%}$.

Explanation of Solution

Express the isentropic efficiency of the compressor.

${\eta }_C=\frac{h_{2s}-h_1}{h_2-h_1}\times 100\%$ (V)

Here, specific enthalpy at state 2s is $h_{2s}$, isentropic efficiency is ${\eta }_C$ and specific enthalpy at state 1 and 2 is $h_1\text{and}{h}_2$ respectively.

Calculate the reversible power for the compressor.

${\dot{W}}_{\text{rev}}=\dot{m}\left[\left(h_2-h_1\right)-T_0\left(s_2-s_1\right)\right]$ (VI)

Here, ambient air temperature is $T_0$.

Calculate the exergy efficiency for the compressor.

${\eta }_{\text{ex},C}=\frac{{\dot{W}}_{\text{rev}}}{{\dot{W}}_{\text{in}}}\times 100\%$ (VII)

Conclusion:

Substitute 285.34 kJ/kg for $h_{2s}$, 245.15 kJ/kg for $h_1$, and 289.66 kJ/kg for $h_2$ in Equation (V).

$\begin{array}{c}{\eta }_C=\frac{285.34\text{kJ/kg}-245.15\text{kJ/kg}}{289.66\text{kJ/kg}-245.15\text{kJ/kg}}\times 100\%\\ =0.903\times 100\%\\ =90.3\%\end{array}$

Thus, the isentropic efficiency of the compressor is $\underset{\_}{90.3\%}$.

Substitute 0.06 kg/s for $\dot{m}$, 245.15 kJ/kg for $h_1$, 289.66 kJ/kg for $h_2$, $37°\text{C}$ for $T_0$, $0.9615\text{kJ}/\text{kg}\cdot \text{K}$ for $s_2$, and $0.9484\text{kJ}/\text{kg}\cdot \text{K}$ for $s_1$ in Equation (VI).

$\begin{array}{c}{\dot{W}}_{\text{rev}}=0.06\text{kg/s}\left[\left(289.66\text{kJ/kg}-245.15\text{kJ/kg}\right)-37°\text{C}\left(\begin{array}{l}0.9615\text{kJ}/\text{kg}\cdot \text{K}-\\ 0.9484\text{kJ}/\text{kg}\cdot \text{K}\end{array}\right)\right]\\ =0.06\text{kg/s}\left[\left(\begin{array}{l}289.66\text{kJ/kg}-\\ 245.15\text{kJ/kg}\end{array}\right)-\left(37+273\right)\text{K}\left(\begin{array}{l}0.9615\text{kJ}/\text{kg}\cdot \text{K}-\\ 0.9484\text{kJ}/\text{kg}\cdot \text{K}\end{array}\right)\right]\\ =2.428\text{kJ/s}\times \frac{\text{1}\text{kW}}{\text{kJ/s}}\\ =2.428\text{kW}\end{array}$

Substitute 2.428 kW for ${\dot{W}}_{\text{rev}}$ and 2.670 kW for ${\dot{W}}_{\text{in}}$ in Equation (VII).

$\begin{array}{c}{\eta }_{\text{ex},C}=\frac{2.428\text{kW}}{2.670\text{kW}}\times 100\%\\ =0.909\times 100\%\\ =90.9\%\end{array}$

Thus, the exergy efficiency of the compressor is $\underset{\_}{90.9\%}$.

To determine

The exergy destruction in each component of the cycle.

The total exergy destruction in the cycle.

Answer to Problem 112RP

The exergy destruction in each component of the cycle are $\underset{\_}{\text{0}\text{.2426}\text{kW}}\text{,}\underset{\_}{\text{0}\text{.3452}\text{kW}}\text{,}\underset{\_}{\text{0}\text{.5202}\text{kW}}\text{,}\text{and}\underset{\_}{1.115\text{kW}}$ respectively.

The total exergy destruction in the cycle is $\underset{\_}{\text{2}\text{.223}\text{kW}}$.

Explanation of Solution

Compressor

Express the entropy generation for cycle 1-2.

${\dot{S}}_{\text{gen,1-2}}=\dot{m}\left(s_2-s_1\right)$ (VIII)

Express the exergy destruction for the cycle 1-2.

$\dot{E}{x}_{\text{dest},1-2}=T_0{\dot{S}}_{\text{gen},1-2}$ (IX)

Condenser

Express the entropy generation for cycle 2-3.

${\dot{S}}_{\text{gen,2-3}}=\dot{m}\left(s_3-s_2\right)+\frac{{\dot{Q}}_H}{T_H}$ (X)

Here, the rate of heat rejected is ${\dot{Q}}_H$.

Calculate the value of ${\dot{Q}}_H$.

${\dot{Q}}_H=\dot{m}\left(h_2-h_3\right)$

Substitute $\dot{m}\left(h_2-h_3\right)$ for ${\dot{Q}}_H$ in Equation (X).

${\dot{S}}_{\text{gen,2-3}}=\dot{m}\left(s_3-s_2\right)+\frac{\dot{m}\left(h_2-h_3\right)}{T_H}$ (XI)

Express the exergy destruction for the cycle 2-3.

$\dot{E}{x}_{\text{dest},2-3}=T_0{\dot{S}}_{\text{gen},2-3}$ (XII)

Expansion valve

Express the entropy generation for cycle 3-4.

${\dot{S}}_{\text{gen,}\text{3-4}}=\dot{m}\left(s_4-s_3\right)$ (XIII)

Express the exergy destruction for the cycle 3-4.

$\dot{E}{x}_{\text{dest},3-4}=T_0{\dot{S}}_{\text{gen},3-4}$ (XIV)

Evaporator

Express the entropy generation for cycle 4-1.

${\dot{S}}_{\text{gen,4-1}}=\dot{m}\left(s_1-s_4\right)+\frac{{\dot{Q}}_L}{T_L}$ (XV)

Here, temperature at refrigeration load is $T_L$.

Express the exergy destruction for the cycle 4-1.

$\dot{E}{x}_{\text{dest},4-1}=T_0{\dot{S}}_{\text{gen},4-1}$ (XVI)

Calculate the total exergy destruction.

$\dot{E}{x}_{\text{dest,}\text{total}}=\dot{E}{x}_{\text{dest},1-2}+\dot{E}{x}_{\text{dest},2-3}+\dot{E}{x}_{\text{dest},3-4}+\dot{E}{x}_{\text{dest},4-1}$ (XVII)

Conclusion:

Substitute 0.06 kg/s for $\dot{m}$, $0.9615\text{kJ}/\text{kg}\cdot \text{K}$ for $s_2$, and $0.9484\text{kJ}/\text{kg}\cdot \text{K}$ for $s_1$ in Equation (VIII).

$\begin{array}{c}{\dot{S}}_{\text{gen,1-2}}=0.06\text{kg/s}\left(0.9615\text{kJ}/\text{kg}\cdot \text{K}-0.9484\text{kJ}/\text{kg}\cdot \text{K}\right)\\ =0.0007827\text{kW/K}\end{array}$

Substitute $0.0007827\text{kW/K}$ for ${\dot{S}}_{\text{gen,1-2}}$ and $37°\text{C}$ for $T_0$ in Equation (IX).

$\begin{array}{c}\dot{E}{x}_{\text{dest},1-2}=37°\text{C}\left(0.0007827\text{kW/K}\right)\\ =\left(37+273\right)\text{K}\left(0.0007827\text{kW/K}\right)\\ =0.2426\text{kW}\end{array}$

Substitute 0.06 kg/s for $\dot{m}$, $0.9615\text{kJ}/\text{kg}\cdot \text{K}$ for $s_2$, $0.3949\text{kJ}/\text{kg}\cdot \text{K}$ for $s_3$, 289.66 kJ/kg for $h_2$, 108.27 kJ/kg for $h_3$, and $37°\text{C}$ for $T_H$ in Equation (XI).

$\begin{array}{c}{\dot{S}}_{\text{gen,2-3}}=0.06\text{kg/s}\left(\begin{array}{l}0.3949\text{kJ}/\text{kg}\cdot \text{K}-\\ 0.9615\text{kJ}/\text{kg}\cdot \text{K}\end{array}\right)+\frac{0.06\text{kg/s}\left(108.27\text{kJ/kg}-289.66\text{kJ/kg}\right)}{37°\text{C}}\\ =0.001114\text{kW/K}\end{array}$

Substitute $0.001114\text{kW/K}$ for ${\dot{S}}_{\text{gen,2-3}}$ and $37°\text{C}$ for $T_0$ in Equation (XII).

$\begin{array}{c}\dot{E}{x}_{\text{dest},2-3}=37°\text{C}\left(0.001114\text{kW/K}\right)\\ =\left(37+273\right)\text{K}\left(0.001114\text{kW/K}\right)\\ =0.3452\text{kW}\end{array}$

Substitute 0.06 kg/s for $\dot{m}$, $0.4229\text{kJ}/\text{kg}\cdot \text{K}$ for $s_3$, and $0.3949\text{kJ}/\text{kg}\cdot \text{K}$ for $s_4$ in Equation (XIII).

$\begin{array}{c}{\dot{S}}_{\text{gen,3-4}}=0.06\text{kg/s}\left(0.4229\text{kJ}/\text{kg}\cdot \text{K}-0.3949\text{kJ}/\text{kg}\cdot \text{K}\right)\\ =0.001678\text{kW/K}\end{array}$

Substitute $0.001678\text{kW/K}$ for ${\dot{S}}_{\text{gen,1-2}}$ and $37°\text{C}$ for $T_0$ in Equation (XIV).

$\begin{array}{c}\dot{E}{x}_{\text{dest},3-4}=37°\text{C}\left(0.001678\text{kW/K}\right)\\ =\left(37+273\right)\text{K}\left(0.001678\text{kW/K}\right)\\ =0.5202\text{kW}\end{array}$

Substitute 0.06 kg/s for $\dot{m}$, $0.9484\text{kJ}/\text{kg}\cdot \text{K}$ for $s_1$, $0.4229\text{kJ}/\text{kg}\cdot \text{K}$ for $s_4$, 8.213 kW for ${\dot{Q}}_L$, and $21°\text{C}$ for $T_L$ in Equation (XV).

$\begin{array}{c}{\dot{S}}_{\text{gen,4-1}}=0.06\text{kg/s}\left(\begin{array}{l}0.9484\text{kJ}/\text{kg}\cdot \text{K}-\\ 0.4229\text{kJ}/\text{kg}\cdot \text{K}\end{array}\right)-\frac{8.213\text{kW}}{21°\text{C}}\\ =0.06\text{kg/s}\left(\begin{array}{l}0.9484\text{kJ}/\text{kg}\cdot \text{K}-\\ 0.4229\text{kJ}/\text{kg}\cdot \text{K}\end{array}\right)-\frac{8.213\text{kW}}{\left(21+273\right)\text{K}}\\ =0.003597\text{kW/K}\end{array}$

Substitute $0.003597\text{kW/K}$ for ${\dot{S}}_{\text{gen,4-1}}$ and $37°\text{C}$ for $T_0$ in Equation (XVI).

$\begin{array}{c}\dot{E}{x}_{\text{dest},4-1}=37°\text{C}\left(0.003597\text{kW/K}\right)\\ =\left(37+273\right)\text{K}\left(0.003597\text{kW/K}\right)\\ =1.115\text{kW}\end{array}$

Thus, the exergy destruction in each component of the cycle are $\underset{\_}{\text{0}\text{.2426}\text{kW}}\text{,}\underset{\_}{\text{0}\text{.3452}\text{kW}}\text{,}\underset{\_}{\text{0}\text{.5202}\text{kW}}\text{,}\text{and}\underset{\_}{1.115\text{kW}}$ respectively.

Substitute 0.2426 kW for $\dot{E}{x}_{\text{dest},1-2}$, 0.3452 kW for $\dot{E}{x}_{\text{dest},2-3}$, 0.5202 kW for $\dot{E}{x}_{\text{dest},3-4}$, and 1.115 kW for $\dot{E}{x}_{\text{dest},4-1}$ in Equation (XVII).

$\begin{array}{c}\dot{E}{x}_{\text{dest,}\text{total}}=\left(0.2426\text{kW}\right)+\left(0.3452\text{kW}\right)+\left(0.5202\text{kW}\right)+\left(1.115\text{kW}\right)\\ =2.223\text{kW}\end{array}$

Thus, the total exergy destruction in the cycle is $\underset{\_}{\text{2}\text{.223}\text{kW}}$.

To determine

The minimum power input of the cycle.

The second law efficiency of the cycle.

Answer to Problem 112RP

The minimum power input of the cycle is $\underset{\_}{\text{0}\text{.4470}\text{kW}}$.

The second law efficiency of the cycle is $\underset{\_}{16.7\%}$.

Explanation of Solution

Express the exergy of the heat transferred from the low-temperature medium.

$\dot{E}{x}_{{\dot{Q}}_L}=-{\dot{Q}}_L\left(1-\frac{T_0}{T_L}\right)$ (XVIII)

Here, minimum power input of the cycle is the exergy of the heat transferred from the low-temperature medium.

Calculate the second law efficiency of the cycle.

${\eta }_{\text{II}}=\frac{{\dot{W}}_{\text{in,min}}}{{\dot{W}}_{\text{in}}}\times 100\%$ (XIX)

Calculate the total exergy destruction in the cycle.

$\dot{E}{x}_{\text{dest,}\text{total}}={\dot{W}}_{\text{in}}-E{x}_{{\dot{Q}}_L}$ (XX)

Conclusion:

Substitute 8.213 kW for ${\dot{Q}}_L$, $37°\text{C}$ for $T_0$, and $21°\text{C}$ for $T_L$ in Equation (XVIII).

$\begin{array}{c}\dot{E}{x}_{{\dot{Q}}_L}=-8.213\text{kW}\left(1-\frac{37°\text{C}}{21°\text{C}}\right)\\ =-8.213\text{kW}\left(1-\frac{\left(37+273\right)\text{K}}{\left(21+273\right)\text{K}}\right)\\ =0.4470\text{kW}\end{array}$

Thus, the minimum power input to the cycle is $\underset{\_}{\text{0}\text{.4470}\text{kW}}$.

Substitute 0.4470 kW for ${\dot{W}}_{\text{in,min}}$ and 2.670 kW for ${\dot{W}}_{\text{in}}$ in Equation (XIX).

$\begin{array}{c}{\eta }_{\text{II}}=\frac{0.4470\text{kW}}{2.670\text{kW}}\times 100\%\\ =0.167\times 100\%\\ =16.7\%\end{array}$

Thus, the second law efficiency of the cycle is $\underset{\_}{16.7\%}$.