
Concept explainers
Waves in the Earth and the Ocean
In December 2004, a large earthquake off the coast of Indonesia produced a devastating water wave, called a tsunami, that caused tremendous destruction thousands of miles away from the earthquake's epicenter. The tsunami was a dramatic illustration of the energy carried by waves.
It was also a call to action. Many of the communities hardest hit by the tsunami were struck hours after the waves were generated, long after seismic waves from the earthquake that passed through the earth had been detected al distant recording stations, long after the possibility of a tsunami was first discussed. With better detection and more accurate models of how a tsunami is formed and how a tsunami propagates, the affected communities could have received advance warning. The study of physics may seem an abstract undertaking with few practical applications, but on this day a better scientific understanding of these waves could have averted tragedy.
Let’s use our knowledge of waves to explore the properties of a tsunami. In Chapter 15, we saw that a vigorous shake of one end of a rope causes a pulse to travel
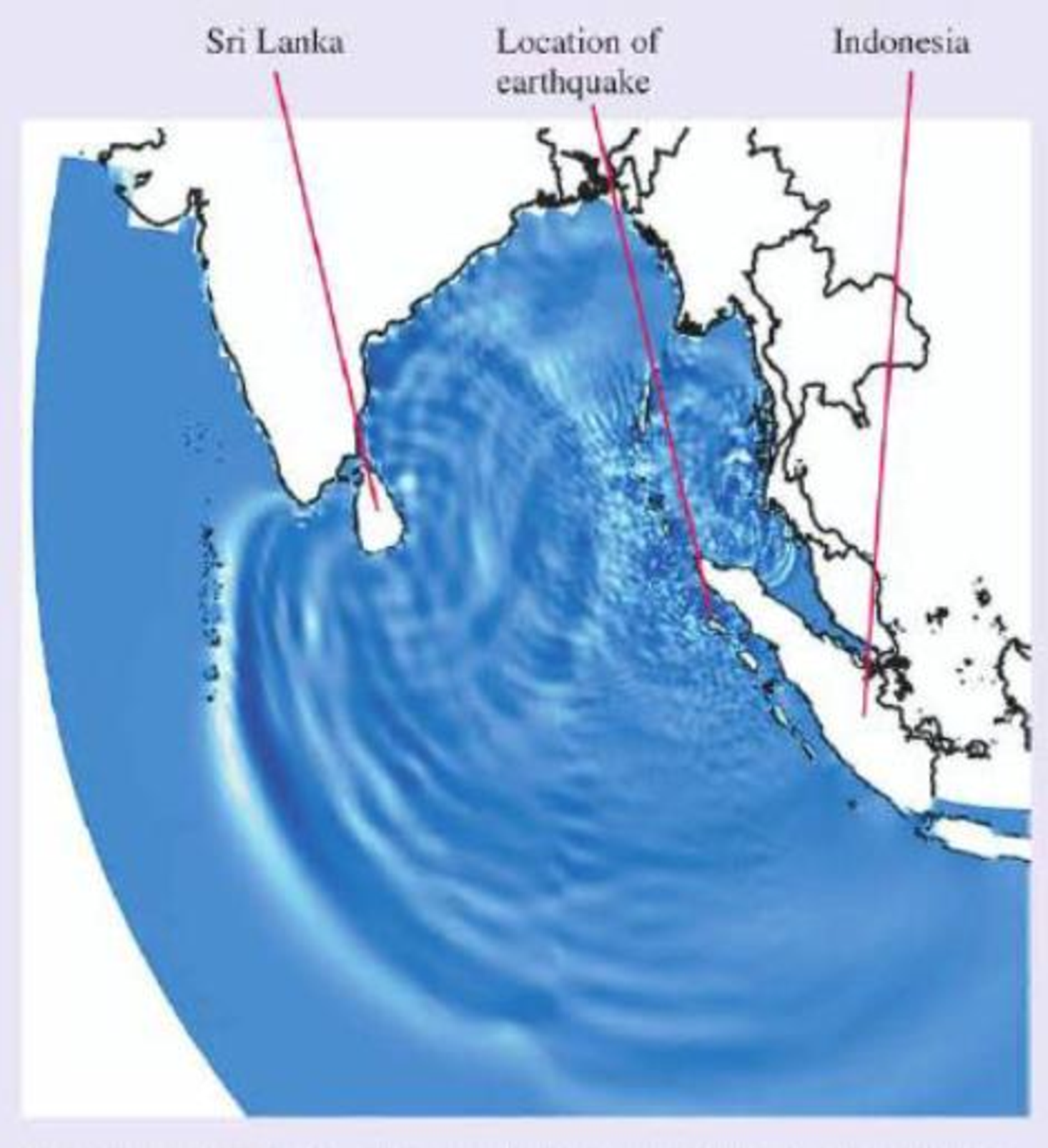
One frame from a computer simulation of the Indian Ocean tsunami three hours after the earthquake that produced it. The disturbance propagating outward from the earthquake is clearly seen, as are wave reflections from the island of Sri Lanka.
along it, carrying energy as it goes. The earthquake that produced the Indian Ocean tsunami of 2004 caused a sudden upward displacement of the seafloor that produced a corresponding rise in the surface of the ocean. This was the disturbance that produced the tsunami, very much like a quick shake on the end of a rope. The resulting wave propagated through the ocean, as we see in the figure.
This simulation of the tsunami looks much like the ripples that spread when you drop a pebble into a pond. But there is a big difference—the scale. The fact that you can see the individual waves on this diagram that spans 5000 km is quite revealing. To show up so clearly, the individual wave pulses must be very wide—up to hundreds of kilometers from front to back.
A tsunami is actually a “shallow water wave,” even in the deep ocean, because the depth of the ocean is much less than the width of the wave. Consequently, a tsunami travels differently than normal ocean waves. In Chapter 15 we learned that wave speeds are fixed by the properties of the medium. That is true for normal ocean waves, but the great width of the wave causes a tsunami to “feel the bottom.” Its wave speed is determined by the depth of the ocean: The greater the depth, the greater the speed. In the deep ocean, a tsunami travels at hundreds of kilometers per hour, much faster than a typical ocean wave. Near shore, as the ocean depth decreases, so docs the speed of the wave.
The height of the tsunami in the open ocean was about half a meter. Why should such a small wave—one that ships didn't even notice as it passed—be so fearsome? Again, it's the width of the wave that matters. Because a tsunami is the wave motion of a considerable mass of water, great energy is involved. As the front of a tsunami wave nears shore, its speed decreases, and the back of the wave moves faster than the front. Consequently, the width decreases. The water begins to pile up, and the wave dramatically increases in height.
The Indian Ocean tsunami had a height of up to 15 m when it reached shore, with a width of up to several kilometers. This tremendous mass of water was still moving at high speed, giving it a great deal of energy. A tsunami reaching the shore isn’t like a typical wave that breaks and crashes. It is a kilometers-wide wall of water that moves onto the shore and just keeps on coming. In many places, the water reached 2 km inland.
The impact of the Indian Ocean tsunami was devastating, but it was the first tsunami for which scientists were able to use satellites and ocean sensors to make planet-wide measurements. An analysis of the data has helped us better understand the physics of these ocean waves. We won’t be able to stop future tsunamis, but with a better knowledge of how they are formed and how they travel, we will be better able to warn people to get out of their way.
The following questions are related to the passage “Waves in the Earth and the Ocean” on the previous page.
The tsunami described in the passage produced a very erratic pattern of damage, with some areas seeing very large waves and nearby areas seeing only small waves. Which of the following is a possible explanation?
A. Certain areas saw the wave from the primary source, others only the reflected waves.
B. The superposition of waves from the primary source and reflected waves produced regions of constructive and destructive interference.
C. A tsunami is a standing wave, and certain locations were at nodal positions, others at antinodal positions.
Want to see the full answer?
Check out a sample textbook solution
Chapter P Solutions
College Physics: A Strategic Approach (3rd Edition)
Additional Science Textbook Solutions
Microbiology: An Introduction
Chemistry (7th Edition)
Organic Chemistry (8th Edition)
Anatomy & Physiology (6th Edition)
Applications and Investigations in Earth Science (9th Edition)
Human Physiology: An Integrated Approach (8th Edition)
- Help me make a visualize experimental setup using a word document. For the theory below.arrow_forwardHow to solve this, given answerarrow_forwardThree point-like charges are placed at the corners of a square as shown in the figure, 28.0 cm on each side. Find the minimum amount of work required by an external force to move the charge q1 to infinity. Let q1=-2.10 μC, q2=+2.40 μС, q3=+3.60 μC.arrow_forward
- A point charge of -4.00 nC is at the origin, and a second point charge of 6.00 nC is on the x axis at x= 0.820 mm . Find the magnitude and direction of the electric field at each of the following points on the x axis. x2 = 19.0 cmarrow_forwardFour point-like charges are placed as shown in the figure, three of them are at the corners and one at the center of a square, 36.0 cm on each side. What is the electric potential at the empty corner? Let q1=q3=+26.0 µС, q2=-28.0 μC, and q4=-48.0μc Varrow_forwardPLS HELparrow_forward
 Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College
College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College An Introduction to Physical SciencePhysicsISBN:9781305079137Author:James Shipman, Jerry D. Wilson, Charles A. Higgins, Omar TorresPublisher:Cengage Learning
An Introduction to Physical SciencePhysicsISBN:9781305079137Author:James Shipman, Jerry D. Wilson, Charles A. Higgins, Omar TorresPublisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning





