Chapter 4.1, Problem 97AYU

To determine

To analyze: the given polynomial function

Answer to Problem 97AYU

  

  

Explanation of Solution

Given:

  $f\left(x\right)=x^3+x^2-12x$

Calculation:

Let us consider the function $f\left(x\right)=x^3+x^2-12x$

This polynomial function $f\left(x\right)$ can be factored as

  $\begin{array}{l}f\left(x\right)=x^3+x^2-12x\hfill \\ \begin{array}{l}=x\left(x^2+x-12x\right)\\ =x\left(x^2+4x-3x-12\right)\\ =x\left\{x\left(x+4\right)-3\left(x+4\right)\right\}\\ =x\left(x-3\right)\left(x+4\right)\end{array}\hfill \end{array}$

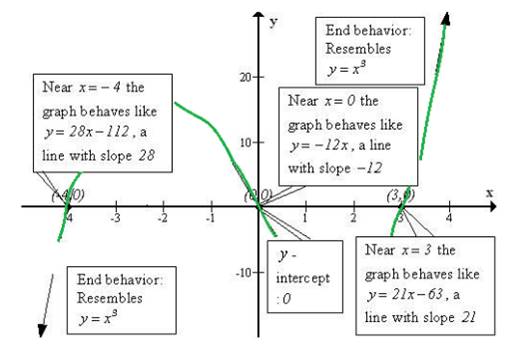
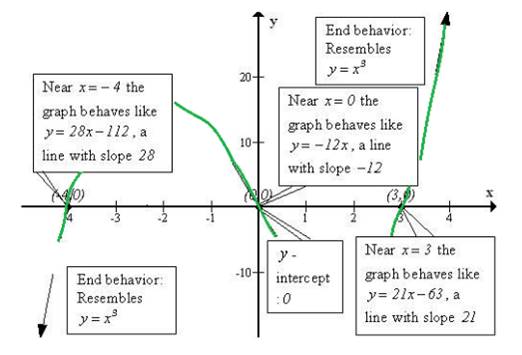
Step1: Determine the end behavior of the graph of the function

Expand the polynomial to write it in the form

  $\begin{array}{l}\begin{array}{l}f\left(x\right)=a_n{x}^n+a_{n-1}{x}^{n-1}+\mathrm{...}+a_{1}x+a_0\\ f\left(x\right)=x\left(x+4\right)\left(x-3\right)\end{array}\hfill \\ =x\left(x^2+4x-3x-12\right)\hfill \\ \begin{array}{l}=x\left(x^2+x-12\right)\\ =x^3+x^2-12x\end{array}\hfill \end{array}$

The polynomial function f is of degree 3. The graph of f behaves like $y=x^3$ for large values of $\left|x\right|$ .

Step2: find the x -and y -intercepts of the graph of the function

The y -intercept is $f\left(0\right)=0$ . To find the x -intercepts, solve $f\left(x\right)=0$

  $x\left(x+4\right)\left(x-3\right)=0$

A product can be zero when any of the factors is zero.

So, $x=0,-4,3$ are the x -intercepts of the given polynomial.

Step3: determine the zeros of the function and their multiplicity. Use this information to determine whether the graph crosses or touches the x -axis at each x -intercept.

The zeros or roots of fare -4, 0,3. The root-4is a zero of multiplicity 1, so the graph off crosses the x -axis at $x=-4$ . The root 0 is a zero of multiplicity1. So the graph of f crosses thex -axis at the origin. The root 3 is a zero of multiplicity 1. So the graph of f crosses the x -axis at $x=3$ .

Step 4: Determine the maximum number of turning points on the graph of the function.

While the polynomial function is of degree 3 (step1), the graph of the function will have at most $3-1=2$ points.

Step5: Determine the behavior of the graph of f near each x -intercept.

Thex -intercepts are - 4,0, and 3.

So, the behavior of the function near -4 is

  $\begin{array}{l}f\left(x\right)=x\left(x+4\right)\left(x-3\right)\\ \approx \left(-4\right)\left(x+4\right)\left(-4-3\right)\\ \approx 28\left(x+4\right)\\ \approx 28x+112\end{array}$

This is a line with slope 28

The behavior of the function near 0:

  $\begin{array}{l}f\left(x\right)=x\left(x+4\right)\left(x-3\right)\\ \approx x\left(0+4\right)\left(0-3\right)\\ \approx -12x\end{array}$

This is also a line with slope -12

The behavior of the function near 3:

  $\begin{array}{l}f\left(x\right)=x\left(x+4\right)\left(x-3\right)\\ \approx 3\left(3+4\right)\left(x-3\right)\\ \approx 21\left(x-3\right)\\ \approx 21x-63\end{array}$

This is a line with slope 21.

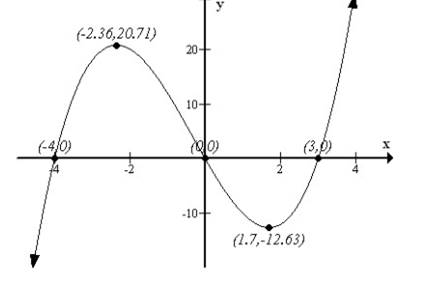
Step 6: Put all the information from Steps 1 through 5 together to obtain the graph of f Figure 1(a) illustrates the information obtained from Steps 1 through 5. Evaluatef at -2.36, 1.7 to help establish the scale on the y -axis. The graph of f is given in Figure 1(b).

  

  

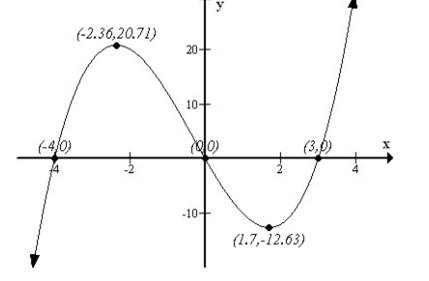
Conclusion:

Therefore, the polynomial function $f\left(x\right)=x^3+x^2-12x$ is analysed with graph.