Chapter 4, Problem 85RE

To determine

The complex zeros of each polynomial function $f(x)$ and write $f$ in factored form.

  $f(x)=2x^4+2x^3-11x^2+x-6$

Answer to Problem 85RE

The complex zeros of $f(x)=2x^4+2x^3-11x^2+x-6$ are $3,2,-\frac{\sqrt{2}}{2}i,\frac{\sqrt{2}}{2}i$ .

Explanation of Solution

Given:

  $f(x)=2x^4+2x^3-11x^2+x-6$

Calculation:

Given polynomial function is $f(x)=2x^4+2x^3-11x^2+x-6$ , now determine the complex zeros of the polynomial function.

First determine maximum number of zeros of the polynomial. The maximum number of zeros of a polynomial is equal to the degree of the polynomial. So here the maximum number of the polynomial is $4$ as the degree of the polynomial is $4$ .

Now, determine all the possible rational zeros of the polynomial.

For that first find out the values of $p,q$ .

Where, $p=$ factors of $a_0=$ factors of $2$

So now,

  $\begin{array}{l}p=\pm 6,\pm 2,\pm 3,\pm 1\\ q=\pm 2,\pm 1\end{array}$

Now find out all of the possible quotients, $\frac{p}{q}$

So, now

  $\frac{p}{q}=\pm \frac{6}{2},\pm \frac{3}{2},\pm \frac{2}{2},\pm \frac{1}{2},\pm \frac{6}{1},\pm \frac{3}{1},\pm \frac{2}{1},\pm \frac{1}{1}$

Here some of the values are repeating, so take unique once.

  $\frac{p}{q}=\pm 6,\pm 2,\pm 3,\pm 1,\pm \frac{3}{1},\pm \frac{1}{1}$

Now plot the graph of the function,

  

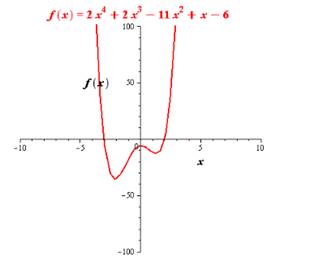
From the above the graph $f$ is intersects $x-$ axis at two distinct points, which are $x=-3,x=2$ .

So, two of the four zeros of the polynomial are $-3$ and $2$ . Therefore, two factors are $\left(x+3\right)$ and $\left(x-2\right)$ . To find the remaining two zeros, divide the polynomial by one of these factors.

First divide the polynomial by $\left(x+3\right)$ using the synthetic method,

  $\begin{array}{cccccc}-3& 2& 2& -11& 1& -6\\ & & -6& 12& -3& 6\\ & 2& -4& 1& -2& 0\end{array}$

So, the factor $f$ as,

  $f\left(x\right)=\left(x+3\right)\left(2x^3-4x^2+1x-2\right)$

Now, solve the depressed equation by grouping,

  $\begin{array}{l}2x^3-4x^2+1x-2=2x^2\left(x-2\right)+1\left(x-2\right)\\ 2x^3-4x^2+1x-2=\left(2x^2+1\right)\left(x-2\right)\end{array}$

So,

  $\begin{array}{l}x-2=0\\ x=2\end{array}$

Also,

  $\begin{array}{l}2x^2+1=0\\ 2x^2=-1\\ x^2=\frac{-1}{2}\\ x=\sqrt{-\frac{1}{2}}\end{array}$

This can be written as,

  $\begin{array}{l}\sqrt{-\frac{1}{2}}=\pm \frac{1}{\sqrt{2}}i\\ \sqrt{-\frac{1}{2}}=\pm \frac{\sqrt{2}}{2}i\end{array}$

Therefore, the zeros of the polynomial function $f(x)=2x^4+2x^3-11x^2+x-6$ are $3,2,-\frac{\sqrt{2}}{2}i,\frac{\sqrt{2}}{2}i$ .

Conclusion:

Hence,the complex zeros of $f(x)=2x^4+2x^3-11x^2+x-6$ are $3,2,-\frac{\sqrt{2}}{2}i,\frac{\sqrt{2}}{2}i$ .