Chapter 4.1, Problem 100AYU

To determine

To analyze: the given polynomial function

Answer to Problem 100AYU

Figure 1(a)

  

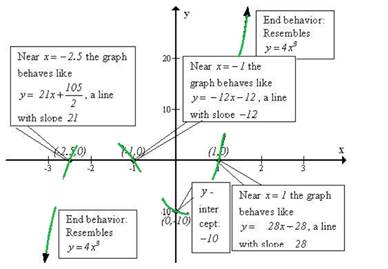
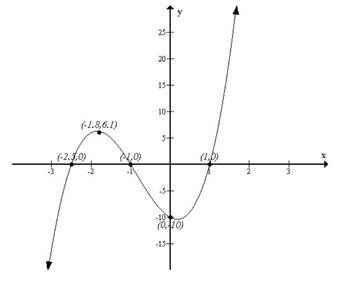
Figure 1(b)

  

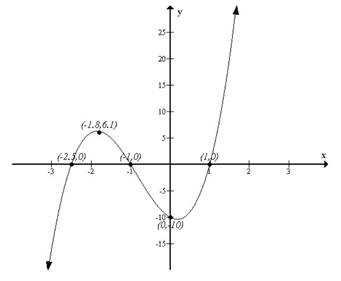
Explanation of Solution

Given:

  $f\left(x\right)=4x^3+10x^2-4x-10$

Calculation:

Let us consider $f\left(x\right)=4x^3+10x^2-4x-10$

This polynomial function can be factored as

  $\begin{array}{c}f\left(x\right)=4x^3+10x^2-4x-10\\ =2x^2\left(2x+5\right)-2\left(2x+5\right)\\ =2\left(x^2-1\right)\left(2x+5\right)\\ =2\left(x-1\right)\left(x+1\right)\left(2x+5\right)\end{array}$

Step1: determine the end behavior of the graph of the function

Expand the polynomial to write it in the form

  $f\left(x\right)=a_n{x}^n+a_{n-1}{x}^{n-1}+\mathrm{...}+a_{1}x+a_0$

In view of this, consider

  $\begin{array}{c}f\left(x\right)=2\left(x-1\right)\left(x+1\right)\left(2x+5\right)\\ =2\left(x^2-1\right)\left(2x+5\right)\\ =\left(2x^2-2\right)\left(2x+5\right)\\ =4x^3+10x^2-4x-10\end{array}$

The polynomial function f is of degree 3. The graph of f behaves like $y=4x^3$ for large positive values of $\left|x\right|$ .

Step2: find the x-and y -intercepts of the graph of the function

  $f\left(x\right)=2\left(x-1\right)\left(x+1\right)\left(2x+5\right)=0$

The product of factors is zero if and only if any one of the factors is zero.

So, $x=1,-1,\frac{-5}{2}$ are the zeroes of roots of the given polynomial.

Step3: to determine the zeros of the function and their multiplicityto graph the function such that the behavior of the function at the intercepts can be observed.

The root $x=\frac{-5}{2}$ is having the multiplicity 1 where the function crosses then function $1^{\text{st}}$ time from left to right of x-axis.

The zero $x=-1$ isof multiplicity 1 andso, the function crosses x-axis $2^{\text{nd}}$ time.

The root $x=1$ is of multiplicity 1 andso, the function crosses x-axis $3^{\text{rd}}$ time.

So, the function crosses x-axis three times.

Step4: Determine the maximum number of turning points on the graph of the function.

While the polynomial function is of degree 3 (step1), the graph of the function will have at most $3-1=2$ turning points.

Step5: determine the behavior of the graph of f near each x-intercept

Near the x-intercept are $1,-1,\frac{-5}{2}$ ,

The behavior of f near $\frac{-5}{2}$ is

  $\begin{array}{c}f\left(x\right)=2\left(2x+5\right)\left(x+1\right)\left(x-1\right)\\ \approx 2\left(2x+5\right)\left(\frac{-5}{2}+1\right)\left(\frac{-5}{2}-1\right)\\ \approx \frac{21}{2}\left(2x+5\right)\\ \approx 21x+\frac{105}{2}\end{array}$

This is a straight line having the slope 21.

The behavior of f near $-1$ is

  $\begin{array}{c}f\left(x\right)=2\left(2x+5\right)\left(x+1\right)\left(x-1\right)\\ \approx 2\left(2-\left(-1\right)+5\right)\left(x+1\right)\left(-1-1\right)\\ \approx -12\left(x+1\right)\\ \approx -12x-12\end{array}$

This is a straight line having the negative slope $-12$ .

The behavior of f near 1 is

  $\begin{array}{c}f\left(x\right)=2\left(2x+5\right)\left(x+1\right)\left(x-1\right)\\ \approx 2\left(2\left(1\right)+5\right)\left(1+1\right)\left(x-1\right)\\ \approx 28\left(x-1\right)\\ \approx 28x-28\end{array}$

This is a straight line having the slope 28.

Step 6: Put all the information from Steps 1 through 5 together to obtain the graph of f Figure 1 (a) illustrates the information obtained from Steps 1 through 5. Evaluatef at $-1,8,0$ to help establish the scale on the y-axis. The graph of f is given in Figure 1 (b).

Figure 1(a)

  

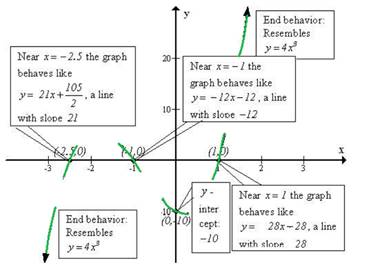
Figure 1(b)

  

Conclusion:

Therefore, the given polynomial function is analyzed.