9th Edition

ISBN: 9780321716835

Author: Michael Sullivan

Publisher: Addison Wesley

See similar textbooks

Videos

Question

Chapter 4.3, Problem 41AYU

To determine

To analyze: the graph of given function

Expert Solution & Answer

Answer to Problem 41AYU

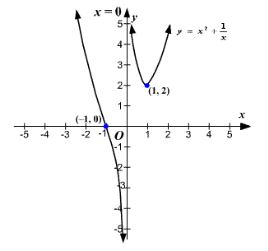
Precalculus, Chapter 4.3, Problem 41AYU , additional homework tip 1

Explanation of Solution

Given:

$f(x)=x2+1x$

Calculation:

STEP 1: Rewrite the function as $f(x)=x3+1x$

Now, factor the function $f$ .

$f(x)=(x+1)(x2−x+1)x$

The domain of $f$ is ${x|x=0}$ .

Since $0$ is in the domain, there is no $y−intercept$ .

STEP 2: The function $f$ is in lowest terms. Since $x+1=0$ has the real solution $x=−1,−1 is the x−intercept$ .

Determine the behavior of the graph near the $x−intercept$ .

$R(x)=x3+1−1≈−1(x3+1)$

Plot the point $(−1, 0)$ and indicate a line with slope $−1$ on the graph.

STEP 3: $f$ is in lowest terms. So, $x=0$ is a vertical asymptote of $f$ . Graph the line $x=0$ using a dashed line.

STEP 4: Degree of the numerator is greater than the degree of the denominator. So, the rational function is improper. To find any horizontal or oblique asymptote, we use the long division.

$xx2x3+1x3 1$

The quotient is $x2$ . So, the graph does not have any horizontal or oblique asymptote. But the graph of $f$ will approach the graph of $y=x2 as x→−∞ and x→∞$ . The graph of $f$ does not intersect with $y=x2$ .

STEP 5: The numerator has one zero at $−1$ and the denominator has one zero at $0$ . Therefore, we divide the $x−axis$ into three intervals. The three intervals are $(−∞, −1), (−1, 0), (0, ∞)$ .

Now, construct the table.

Precalculus, Chapter 4.3, Problem 41AYU , additional homework tip 2

Interval Number chosen Value of f Location of graph Point on graph

$(−∞,−1)−2f(−2)=3.5Above x−axis(−2,3.5)$

$(−1,0)−12 f(−12)=−1.75Below x−axis(−12,−1.75)$

$(0,∞)1f(1)=2Above x−axis(1,2)$

Plot the points from the table obtained.

Precalculus, Chapter 4.3, Problem 41AYU , additional homework tip 3

STEP 6: Since the graph of $f$ is above the $x−axis for x<−1$ , we place arrows above the $x−axis$ as shown in the figure. Similarly, as the graph of $f$ is below the $x−axis for −1<x<0$ , we place arrows below the $x−axis$ . Also, as the graph of $f$ is above the $x−axis for x>0$ , we place arrows above the $x−axis$ as shown in the figure.

Precalculus, Chapter 4.3, Problem 41AYU , additional homework tip 4

STEP 7: The figure shows the final graph.

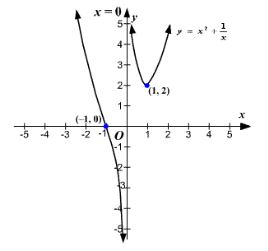
Precalculus, Chapter 4.3, Problem 41AYU , additional homework tip 5

Conclusion:

Thus, the graph of $f$ is obtained which illustrates the information obtained from Steps 1 through 6.

Chapter 4.3, Problem 40AYU

Chapter 4.3, Problem 42AYU

Chapter 4 Solutions

Precalculus

Ch. 4.1 - The intercepts of the equation 9 x 2 +4y=36 are...Ch. 4.1 - Is the expression 4 x 3 3.6 x 2 2 a polynomial?...Ch. 4.1 - To graph y= x 2 4 , you would shift the graph of...Ch. 4.1 - Prob. 4AYU Ch. 4.1 - Prob. 5AYU Ch. 4.1 - Prob. 6AYU Ch. 4.1 - The graph of every polynomial function is both...Ch. 4.1 - If r is a real zero of even multiplicity of a...Ch. 4.1 - The graphs of power functions of the form f(x)= x...Ch. 4.1 - If r is a solution to the equation f(x)=0 , name...

Additional Math Textbook Solutions

Find more solutions based on key concepts

In Exercises 13–16, find the margin of error for the values of c, ?, and n. 13. c = 0.95, ? = 5.2, n = 30

Elementary Statistics: Picturing the World (7th Edition)

Find how many SDs above the mean price would be predicted to cost.

Intro Stats, Books a la Carte Edition (5th Edition)

CHECK POINT I Let p and q represent the following statements: p : 3 + 5 = 8 q : 2 × 7 = 20. Determine the truth...

Thinking Mathematically (6th Edition)

Find the limits in Exercises 11–20. 11.

University Calculus: Early Transcendentals (4th Edition)

Second derivatives Find d2ydx2. 35. e2y + x = y

Calculus: Early Transcendentals (2nd Edition)

The table by using the given graph of h.

Calculus for Business, Economics, Life Sciences, and Social Sciences (14th Edition)

Knowledge Booster

$Calculus$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.