Chapter 11.5, Problem 22PSC

To determine

To calculate: Thetotal area of shaded regions.

Answer to Problem 22PSC

The total area of shaded regions is $12\sqrt{3}$ .

Explanation of Solution

Given information:

A set of four concentric regular hexagons, each with a radius 1 unit longer than that of the next smaller hexagon.

Formula used:

Apothem of polygon:

  $a=\frac{s}{2\tan \left(\frac{{180}^{\circ }}{n}\right)}$

wheres = side of polygon,

n = number of sides

  $Areaofregularpolygon=\frac{1}{2}\times a\times P$

wherea = Apothem,

P = Perimeter

Calculation:

  

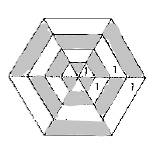
Since half of the total area of the hexagon is shaded,

  $A_{ShadedRegion}=\frac{1}{2}\times A_{Hexagon}$

The radius of hexagon is 4.

A ${30}^{\circ }{60}^{\circ }{90}^{\circ }\Delta$ is formed with hypotenuse = 4.

n =6

Apothem of polygon:

  $a=\frac{4}{2\tan \left(\frac{{180}^{\circ }}{6}\right)}=\frac{4}{2\tan \left({30}^{\circ }\right)}=\frac{4}{2\times \frac{1}{\sqrt{3}}}=2\sqrt{3}$

  $P=4+4+4+4+4+4=24$

Apothem is $2\sqrt{3}$ and Perimeter is $24$ .

  $\begin{array}{l}{A}_{RegularPolygon}=\frac{1}{2}\times a\times P\\ A_{RegularPolygon}=\frac{1}{2}\times (2\sqrt{3})(24)\\ A_{RegularPolygon}=24\sqrt{3}\\ A_{Shaded}=\frac{1}{2}\times A_{RegularPolygon}\\ A_{Shaded}=\frac{1}{2}\times 24\sqrt{3}\\ A_{Shaded}=12\sqrt{3}\end{array}$