Chapter 10.2, Problem 68AYU

Suspension Bridge The cables of a suspension bridge are in the shape of a parabola. The towers supporting the cable are 400 feet apart and 100 feet high. If the cables are at a height of 10 feet midway between the towers, what is the height of the cable at a point 50 feet from the center of the bridge?

To determine

To find: The height of the cable from the road at a point 50 feet from the centre of the bridge if the towers supporting the cable are 400 feet apart and 100 feet high and the cables are at the height of 10 feet midway between the towers.

Answer to Problem 68AYU

The height of the cable from the road at the point 50 feet from the center of the bridge is $\text{15}\text{.625}$ feet.

Explanation of Solution

Given:

The cables of a suspension bridge are in the shape of a parabola. The towers supporting the cable are 400 feet apart and 100 feet high. The cables are at a height of 10 feet midway between the towers.

Formula used:

Vertex	Focus	Directrix	Equation	Description
$\left(h,k\right)$	$(h,k+a)$	$y=k-a$	$\left(x-h\right)²=4a\left(y-k\right)$	Parabola, axis of symmetry is parallel to $y\text{-axis}$, opens up.

Calculation:

Let the vertex of the parabola be $\left(0,10\right)$ and the bridge (parabola) opens up.

The equation of the parabola is given by $\left(x-h\right)²=4a\left(y-k\right)$.

Substituting $\left(0,10\right)$ in $\left(x-h\right)²=4a\left(y-k\right)$ we get,

$x²=4a\left(y-10\right)$

From the given data, length of the bridge between two towers is 400 feet, Hence $\left(200,100\right)$ and $\left(-200,100\right)$ lies on the parabola.

Substituting the points in the equation $x²=4a\left(y-10\right)$, we get,

$200²=4*a*\left(100-10\right)$

$a=\frac{{200}^2}{4*90}=\frac{444.4444}{4}$

Hence the equation of the parabola is,

$x²=4*100\left(y-10\right)$

$x²=444.4444\left(y-10\right)$

Parabolic representation is given below.

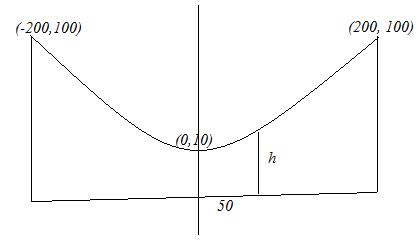
We have to find the height of the cable when it is at a distance of 50 feet from the centre of the bridge. Hence the point $\left(50, h\right)$ is on the parabola.

Substituting the point $\left(50, h\right)$ in the equation of the parabola, we get,

$x²=444.4444\left(y-10\right)$

$50²=444.4444*\left(h-10\right)$

$h-10=\frac{2500}{444.4444}$

$h=5.625+10\approx 15.625$ feet.

The height of the cable from the road at the point 50 feet from the center of the bridge is $\text{15}\text{.625}$ feet.