
Concept explainers
Assume steady-state, one-dimensional heat conduction through the axisymmetric shape shown below.
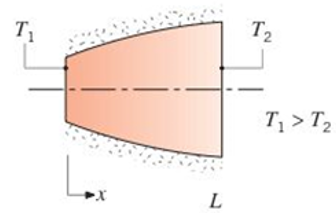
Assuming constant properties and no internal heat generation, sketch the temperature distribution on
To Sketch:The temperature distribution on T-x coordinates along with the explanation of the shape.
Explanation of Solution
Draw the axisymmetric shape as shown below:
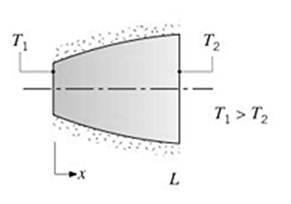
Write the expression as per Fourier law.
Here,
Sincethe heat transfer through the body remains constant and thermal conductivity of the body remains constant the Fourier law can be explained as:
The above expression indicated that temperature and thickness is inversely proportional to one another.
On T-x curve the independent variable is x and the dependent variable is T .
Draw the T-x curve for axisymmetric shape as shown below:
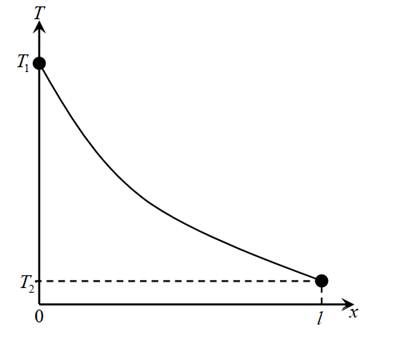
The T-xcurve for axisymmetric shape is shown above and the slope
Want to see more full solutions like this?
Chapter 2 Solutions
Introduction to Heat Transfer
- Problem: You and your team are tasked with designing an around-coil system for an air-conditioning unit that will be installed in a hospital. The system must provide a controlled environment for the hospital's air-conditioning needs, ensuring that fresh air is brought in while exhaust air is expelled, without allowing contamination between the two airflow directions. The air-conditioning system will include two coils and two pumps (one duty pump and one standby pump) in a configuration that accommodates both exhaust and supply airflows. The system needs to comply with ASHRAE Standards, and ASME BPVC Section VIII, with attention to safety, reliability, and efficiency. The system will be used in a climate with moderate temperature variations, and its design will need to consider a range of operating and design specifications. Given data Desired Pressure in the Pipe: According to the (ASHRAE) standard for pressure drop limits. Pipe Diameter: Must comply with standard pipe diameter…arrow_forwardAssis+ 2019-2018 Assi A. SALE تمارين السيطرة النوعية 1- سحبت عينة عشوائية يومياً تتكون من 50 دائرة كهربائية تستخدم لـ صناعة إحدى الأجهزة الأليكترونية ولمدة 20 يوماً ، وبعد فحصها كانت عدد الدوائر المعابة لكل يوم كالآتي : 43.2.6.3.1.3.2.9.3.5.3.2.5.2.2.1.3.2.1 أوجد حدي السيطرة النوعية لنسبة الوحدات المعابة (ans.0, 0.1533) 2- سحبت 10 عينات عشوائية من مصنع لتعبئة الأسماك البحرية وكل عينة تحتوي على كانت أوزانها ( باوند ( كالآتي : ب ، 1 71 X₁ X₂ 1.04 1.01 measurements X3 X4 X5 0.98 1.02 1.00 2 1.02 0.97 0.96 1.01 1.02 3 1.01 1.07 4 0.98 0.97 1.02 0.99 1.03 1.00 0.98 0,98 5 6 0.99 1.03 1.02 0.95 0.98 1.02 1.01 1.04 1.02 0.95 7 1.00 0.99 0.99 1.02 1.03 1.04 0.99 1.02 0.94 8 9 10 1.02 0.98 1.00 0.99 1.02 1.01 1.02 1.01 1.00 1.04 1.09 أوجد حدي السيطرة النوعية : أ) للوسط الحسابي و ب) للمدى (ans. a) 0.9679, 1.0429, b) 0, 0.137) 18 محاضرة السيطرة النوعية / الهندسة الصناعية - 4 ميكانيك / هندسة ج تكريت 3- كون مخطط سيطرة نوعية مناسب للعملية الإنتاجية المتمثلة بالبيانات التالية التي جمعت خلال شهر معين…arrow_forward42 VOLTE 4G+ EV Suggested Que... Problem: You and your team are tasked with designing an around-coil system for an air- conditioning unit that will be installed in a hospital. The system must provide a controlled environment for the hospital's air-conditioning needs, ensuring that fresh air is brought in while exhaust air is expelled, without allowing contamination between the two airflow directions. The air-conditioning system will include two coils and two pumps (one duty pump and one standby pump) in a configuration that accommodates both exhaust and supply airflows. The system needs to comply with ASHRAE Standards, and ASME BPVC Section VIII, with attention to safety, reliability, and efficiency. The system will be used in a climate with moderate temperature variations, and its design will need to consider a range of operating and design specifications. Given data Desired Pressure in the Pipe: According to the (ASHRAE) standard for pressure drop limits. Pipe Diameter: Must comply…arrow_forward
- compute the work done by a fuel-water interaction assuming that the 40,000 kg of mixed oxide fuel and 4000 kg of water expand independently and isentropically to 1 atmosphere. Assume that the initial fuel and water conditions are such that equilibrium mixture temperature achieved is 1945 K. Other water conditions are as follows: Tinitial = 400K, ro initial = 945 kg/m^3, cv = 4184 J/kg-K. Caution: Equation 6.9 is inappropriate for these conditions since the cooland at state e is supercritial.arrow_forwardIf the W12x50 beam below is made of steel having an allowable bending stress of 36 ksi and an allowable shear stress of 15 ksi, determine the maximum cable force, P, that can safely be supported by the beam.arrow_forwardThis is a tilt and rotation question. Here are notes attached for reference. I prefer handwritten solutions. ONLY UPLOAD A SOLUTION IF YOU ARE SURE ABOUT THE ANSWER PLEASE. I prefer handwritten solutions.(If you had once answered this question don't answer it as I am looking for a different solution)arrow_forward
- 3- Find the optimum of y = 9x - 0.1x ^ 2 in the interval 0 <= x <= 100 and alpha = 0.05 Use two and three experiments sequential search methods?arrow_forward1- A manufacturing company is optimizing the cooling time of a newly developed plastic molding process. The goal is to minimize the total production cost, which depends on the cooling time (t) in minutes. The production cost (C, in dollars) is given by: C=50+10(t)-0.5(t)^2 where: 5 st≤ 20 (cooling time in minutes) Using the Two-Experiments Sequential Method (up to five cycles) find the optimal cooling time (t) that minimizes the production cost. 3:29 مarrow_forward2- Find the optimum minimum point of y = x²-6x + 2 in the interval 0 ≤ x ≤ 10 using sequential search method with three experiments. the accuracy a = 0.06. 3- Find the optimum of y = 9x -0.1 x² in the interval 0 ≤ x ≤ 100, and α = 0.05 Use two and three experiments sequential search methods?arrow_forward
 Principles of Heat Transfer (Activate Learning wi...Mechanical EngineeringISBN:9781305387102Author:Kreith, Frank; Manglik, Raj M.Publisher:Cengage Learning
Principles of Heat Transfer (Activate Learning wi...Mechanical EngineeringISBN:9781305387102Author:Kreith, Frank; Manglik, Raj M.Publisher:Cengage Learning
