VECTOR MECH...,DYNAMICS(LOOSE)-W/ACCESS
12th Edition
ISBN: 9781260265521
Author: BEER
Publisher: MCG
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 17.3, Problem 17.114P
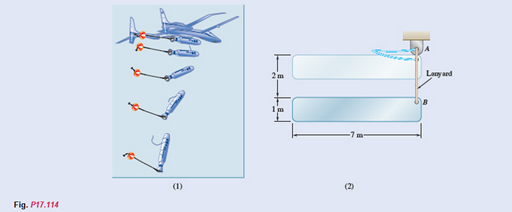
The trapeze/lanyard air drop
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
A projectile object reaches a height of 430 m, if it is fired straight up using a spring-loaded gun. Using the same gun, what is the maximum height (m) that can be reached by the projectile if it fired at an angle of 31 ° from the vertical?
Note:
Please provide a clear, step-by-step handwritten calculation (no written explanations), ensuring it is expertly completed without any AI assistance. I expect an advanced-level handwritten solution and will be evaluated based on both precision and quality. Carefully double-check all calculations to ensure absolute accuracy before submission.
Question:
in Figure 2 , a 500-g collar freely slides on the curved rod BC in a horizontal plane. Given that the undeformed length of the spring is 80 mm and its spring constant, k, is 400 KN/m,
determine:
the initial velocity required at point A for the collar to arrive at point B with zero velocity,
the collar's velocity when it reaches point C.
As speed as possible please
Chapter 17 Solutions
VECTOR MECH...,DYNAMICS(LOOSE)-W/ACCESS
Ch. 17.1 - A round object of mass m and radius r is released...Ch. 17.1 - Prob. 17.CQ2PCh. 17.1 - Prob. 17.CQ3PCh. 17.1 - Prob. 17.CQ4PCh. 17.1 - Slender bar A is rigidly connected to a massless...Ch. 17.1 - A 200-kg flywheel is at rest when a constant 300 N...Ch. 17.1 - The rotor of an electric motor has an angular...Ch. 17.1 - Two uniform disks of the same material are...Ch. 17.1 - Two disks of the same material are attached to a...Ch. 17.1 - Prob. 17.5P
Ch. 17.1 - Prob. 17.6PCh. 17.1 - Prob. 17.7PCh. 17.1 - Prob. 17.8PCh. 17.1 - Prob. 17.9PCh. 17.1 - Prob. 17.10PCh. 17.1 - Each of the gears A and B has a mass of 10 kg and...Ch. 17.1 - Solve Prob. 17.11, assuming that the 6 N m couple...Ch. 17.1 - The gear train shown consists of four gears of the...Ch. 17.1 - Prob. 17.14PCh. 17.1 - Prob. 17.15PCh. 17.1 - Prob. 17.16PCh. 17.1 - The 15-kg rear hatch of a vehicle opens as shown...Ch. 17.1 - A slender 9-lb rod can rotate in a vertical plane...Ch. 17.1 - Prob. 17.19PCh. 17.1 - Prob. 17.20PCh. 17.1 - A collar with a mass of 1 kg is rigidly attached...Ch. 17.1 - Prob. 17.22PCh. 17.1 - Prob. 17.23PCh. 17.1 - The 30-kg turbine disk has a centroidal radius of...Ch. 17.1 - A 100-kg solid cylindrical disk, 800 mm in...Ch. 17.1 - Prob. 17.26PCh. 17.1 - Prob. 17.27PCh. 17.1 - Prob. 17.28PCh. 17.1 - Prob. 17.29PCh. 17.1 - A half-cylinder with mass m and radius r is...Ch. 17.1 - Prob. 17.31PCh. 17.1 - Two uniform cylinders, each of weight W=14 lb and...Ch. 17.1 - Two uniform cylinders, each of weight W=14 lb and...Ch. 17.1 - A bar of mass m=5 kg is held as shown between four...Ch. 17.1 - The 1.5-kg uniform slender bar AB is connected to...Ch. 17.1 - Prob. 17.36PCh. 17.1 - A 5-m-long ladder has a mass of 15 kg and is...Ch. 17.1 - Prob. 17.38PCh. 17.1 - Prob. 17.39PCh. 17.1 - The mechanism shown is one of two identical...Ch. 17.1 - The mechanism shown is one of two identical...Ch. 17.1 - Each of the two rods shown is of length L=1 m and...Ch. 17.1 - The 4-kg rod AB is attached to a collar of...Ch. 17.1 - If in Prob. 17.43 the angular velocity of the...Ch. 17.1 - The uniform rods AB and BC are of mass 3 kg and 8...Ch. 17.1 - The uniform rods AB and BC weigh 2.4 kg and 4 kg,...Ch. 17.1 - The 80-mm-radius gear shown has a mass of 5 kg and...Ch. 17.1 - Prob. 17.48PCh. 17.1 - Three shafts and four gears are used to form a...Ch. 17.1 - Prob. 17.50PCh. 17.1 - The drive belt on a vintage sander transmits 12 hp...Ch. 17.2 - Slender bar A is rigidly connected to a massless...Ch. 17.2 - A 1-m-long uniform slender bar AB has an angular...Ch. 17.2 - The 350-kg flywheel of a small hoisting engine has...Ch. 17.2 - A sphere of radius r and mass m is placed on a...Ch. 17.2 - Prob. 17.F3PCh. 17.2 - Prob. 17.52PCh. 17.2 - Prob. 17.53PCh. 17.2 - Prob. 17.54PCh. 17.2 - Prob. 17.55PCh. 17.2 - Prob. 17.56PCh. 17.2 - A disk of constant thickness, initially at rest,...Ch. 17.2 - Prob. 17.58PCh. 17.2 - A cylinder of radius r and weight W with an...Ch. 17.2 - Each of the double pulleys shown has a centroidal...Ch. 17.2 - Prob. 17.61PCh. 17.2 - Prob. 17.62PCh. 17.2 - Prob. 17.63PCh. 17.2 - A tape moves over the two drums shown. Drum A...Ch. 17.2 - Prob. 17.65PCh. 17.2 - Prob. 17.66PCh. 17.2 - Prob. 17.67PCh. 17.2 - Consider a rigid body initially at rest and...Ch. 17.2 - Prob. 17.69PCh. 17.2 - Prob. 17.70PCh. 17.2 - Prob. 17.71PCh. 17.2 - Prob. 17.72PCh. 17.2 - Prob. 17.73PCh. 17.2 - Prob. 17.74PCh. 17.2 - Prob. 17.75PCh. 17.2 - Prob. 17.76PCh. 17.2 - A sphere of radius r and mass m is projected along...Ch. 17.2 - Prob. 17.78PCh. 17.2 - Prob. 17.79PCh. 17.2 - Prob. 17.80PCh. 17.2 - Two 10-lb disks and a small motor are mounted on a...Ch. 17.2 - Prob. 17.82PCh. 17.2 - A 1.6-kg tube AB can slide freely on rod DE, which...Ch. 17.2 - In the helicopter shown, a vertical tail propeller...Ch. 17.2 - Prob. 17.85PCh. 17.2 - The 4-kg uniform disk B is attached to the shaft...Ch. 17.2 - Prob. 17.87PCh. 17.2 - Prob. 17.88PCh. 17.2 - Prob. 17.89PCh. 17.2 - Prob. 17.90PCh. 17.2 - Prob. 17.91PCh. 17.2 - Prob. 17.92PCh. 17.2 - Prob. 17.93PCh. 17.2 - Prob. 17.94PCh. 17.2 - Prob. 17.95PCh. 17.3 - A uniform slender rod AB ofmass m is at rest on a...Ch. 17.3 - Prob. 17.F5PCh. 17.3 - Prob. 17.F6PCh. 17.3 - Prob. 17.96PCh. 17.3 - A bullet weighing 0.08 lb is fired with a...Ch. 17.3 - Prob. 17.98PCh. 17.3 - Prob. 17.99PCh. 17.3 - Prob. 17.100PCh. 17.3 - Prob. 17.101PCh. 17.3 - A 45-g bullet is fired with a velocity of 400 m/s...Ch. 17.3 - Prob. 17.103PCh. 17.3 - Prob. 17.104PCh. 17.3 - A uniform slender rod AB of mass m is at rest on a...Ch. 17.3 - Prob. 17.106PCh. 17.3 - Prob. 17.107PCh. 17.3 - Prob. 17.108PCh. 17.3 - Determine the height h at which the bullet of...Ch. 17.3 - A uniform slender bar of length L=200 mm and mass...Ch. 17.3 - A uniform slender rod of length L is dropped onto...Ch. 17.3 - A uniform slender rod AB has a mass m, a length L,...Ch. 17.3 - Prob. 17.113PCh. 17.3 - The trapeze/lanyard air drop (t/LAD) launch is a...Ch. 17.3 - The uniform rectangular block shown is moving...Ch. 17.3 - The 40-kg gymnast drops from her maximum height of...Ch. 17.3 - Prob. 17.117PCh. 17.3 - A uniformly loaded square crate is released from...Ch. 17.3 - A 1-oz bullet is fired with a horizontal velocity...Ch. 17.3 - For the beam of Prob. 17.119, determine the...Ch. 17.3 - The plank CDEhas a mass of 15 kg and rests on a...Ch. 17.3 - Prob. 17.122PCh. 17.3 - A slender rod AB is released from rest in the...Ch. 17.3 - A slender rod AB is released from rest in the...Ch. 17.3 - Prob. 17.125PCh. 17.3 - A 2-kg solid sphere of radius r=40 mm is dropped...Ch. 17.3 - Member ABC has a mass of 2.4 kg and is attached to...Ch. 17.3 - Member ABC has a mass of 2.4 kg and is attached to...Ch. 17.3 - Sphere A of mass mA=2 kg and radius r=40 mm rolls...Ch. 17.3 - A large 3-lb sphere with a radius r=3 in. is...Ch. 17.3 - Prob. 17.131PCh. 17.3 - Sphere A of mass m and radius r rolls without...Ch. 17.3 - Prob. 17.133PCh. 17.3 - Prob. 17.134PCh. 17 - A uniform disk, initially at rest and of constant...Ch. 17 - Prob. 17.136RPCh. 17 - Prob. 17.137RPCh. 17 - You are asked to analyze a catcher for a small...Ch. 17 - A uniform slender rod is placed at corner B and is...Ch. 17 - Prob. 17.140RPCh. 17 - Prob. 17.141RPCh. 17 - Prob. 17.142RPCh. 17 - Prob. 17.143RPCh. 17 - A square block of mass m is falling with a...Ch. 17 - Prob. 17.145RPCh. 17 - A 1.8-lb javelin DE impacts a 10-lb slender rod...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- Jumping up before the elevator hits. After the cable snaps and the safety system fails, an elevator cab free-falls from a height of 34 m. During the collision at the bottom of the elevator shaft, a 90 kg passenger is stopped in 6.0 ms. (Assume that neither the passenger nor the cab rebounds.) (a) What is the magnitude of the impulse on the passenger during the collision? N's (b) What is the magnitude of the average force on the passenger during the collision? N Suppose the passenger were to jump upward with a speed of 6.5 m/s relative to the cab floor just before the cab hits the bottom of the shaft. Assume the same stopping time as above. (c) What is the magnitude of the impulse on the passenger during the collision? N•s (d) What is the magnitude of the average force on the passenger during the collision? Narrow_forwardA 35 g steel ball is held by a ceiling-mounted electromagnet 3.4 m above the floor. A compressed-air cannon sits on the floor, 5.0 m to one side of the point directly under the ball. When a button is pressed, the ball drops and, simultaneously, the cannon fires a 25 g plastic ball. The two balls collide 1.2 m above the floor. What was the launch speed of the plastic ball?arrow_forwardNote: Give a clear, step-by-step handwritten calculation (without any explanations), completed entirely without using AI. The solution should be of expert quality, as I will evaluate and rate it based on its precision and overall quality. Be sure to carefully double-check all work for accuracy before submission. Question: In Figure 5, a 0.1-kg projectile is launched into a 10-kg block, initially at rest with an uncompressed spring secured at both ends. Calculate the maximum horizontal displacement, X, of the spring and the resulting oscillation period of the block and the embedded projectile.arrow_forward
- Please show me the steps for a,b,c,darrow_forwardHi! Please help me answer Problem 1, Questions 4 and 5. Question 1-3 have been answered by another tutor. Problem 1, Questions 4 and 5. Problem 1, Questions 4 and 5. Problem 1, Questions 4 and 5.arrow_forwardOblique Impact To analyze an oblique impact using the conservation of momentum and coefficient of restitution. When an oblique impact occurs between two smooth particles, the particles move away from each other with velocity vectors that have unknown directions and unknown magnitudes. If the y axis is within the plane of contact and the x axis is the line of impact, the impulsive forces of deformation and restitution act only along the line of impact (the x axis). Momentum of the system is conserved along the line of impact (the x axis): ∑m(vx)1=∑m(vx)2 The coefficient of restitution, e, relates the relative-velocity components of the particles along the line of impact (the x axis): e=(vBx)2−(vAx)2(vAx)1−(vBx)1 The momenta of both particles A and B are conserved in the plane of contact (the y axis) because no impulse acts on either particle in this plane. Therefore, the y component of the velocities before and after the collisions remains unchanged: (vy)1=(vy)2 As shown,…arrow_forward
- Oblique Impact To analyze an oblique impact using the conservation of momentum and coefficient of restitution. When an oblique impact occurs between two smooth particles, the particles move away from each other with velocity vectors that have unknown directions and unknown magnitudes. If the y axis is within the plane of contact and the x axis is the line of impact, the impulsive forces of deformation and restitution act only along the line of impact (the x axis). Momentum of the system is conserved along the line of impact (the x axis): ∑m(vx)1=∑m(vx)2 The coefficient of restitution, e, relates the relative-velocity components of the particles along the line of impact (the x axis): e=(vBx)2−(vAx)2(vAx)1−(vBx)1 The momenta of both particles A and B are conserved in the plane of contact (the y axis) because no impulse acts on either particle in this plane. Therefore, the y component of the velocities before and after the collisions remains unchanged: Immediately after the collision,…arrow_forwardIf either a simple or a compound pendulum is used to determine experimentally the acceleration of gravity g, difficulties are encountered. In the case of the simple pendulum, the string is not truly weightless, while in the case of the compound pendulum, the exact location of the mass center is difficult to establish. In the case of a compound pendulum, the difficulty can be eliminated by using a reversible, or Kater, pendulum. Two knife edges A and B are placed so that they are obviously not at the same distance from the mass center G, and the distance l is measured with great precision. The position of a counterweight D is then adjusted so that the period of oscillation τ obtained is equal to that of a true simple pendulum of length l and that g 4π2 l/τ.arrow_forwardA small block (block 1) of mass m, attached to an ideal string of length L, is initially held so that the string is taut and horizontal at height Labove a frictionless table, as shown in Fig.2. A second small block (block 2) of mass 3mis placed on the table right under the point of attachment of the string holding block 1. The tabletop is at height 2L above the floor. Block 1 is then released from rest and collides elastically with block 2 at time t=0. The acceleration due to gravity has magnitude g and air resistance is negligible. a) Determine the horizontal distance traveled by block 2as a projectile. b)Determine the speed and direction of motion of block 1 right after the collision. c) Determine the maximum angular displacement (measured from the vertical direction) of block 1 after the collision.arrow_forward
- A small block (block 1) of mass m, attached to an ideal string of length L, is initially held so that the string is taut and horizontal at height Labove a frictionless table, as shown in Fig.2. A second small block (block 2) of mass 3mis placed on the table right under the point of attachment of the string holding block 1. The tabletop is at height 2L above the floor. Block 1 is then released from rest and collides elastically with block 2 at time t=0. The acceleration due to gravity has magnitude g and air resistance is negligible. a)Determine the time t2at which block 2 hits the floor. b) Determine the horizontal distance traveled by block 2as a projectile. c)Determine the speed and direction of motion of block 1 right after the collision.arrow_forward4. When only conservative forces are acting on a system, we can use the conservation of energy theorem to solve a problem. Recall from particle kinetics: T₁ + V₁ = T2 + V2, where V is potential energy (due to both gravitational and elastic forces: V₂ = Wyc and Ve= + ½ ks², respectively). The 30-kg rod is released from rest when 0 = 45°. The spring is unstretched when 0 = 45°. Using conservation of energy theorem, determine the angular velocity of the rod when 0 = 0°. B 1.5 m k = 300 N/m Aarrow_forwardChoose the correct answer of the following questions: 1. If no external impressed force acts on the system, the total momentum (G) of a system ... c. remains constant a. increases b. decreases d. none of the these 2. Which of the following cases momentum is conserved? (1 M) a. Perfectly elastic impact d. Momentum is always conserved c. Perfectly inelastic impact b. plastic impact with 0arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
Recommended textbooks for you
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
Power Transmission; Author: Terry Brown Mechanical Engineering;https://www.youtube.com/watch?v=YVm4LNVp1vA;License: Standard Youtube License