Vector Mechanics for Engineers: Dynamics
11th Edition
ISBN: 9780077687342
Author: Ferdinand P. Beer, E. Russell Johnston Jr., Phillip J. Cornwell, Brian Self
Publisher: McGraw-Hill Education
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 12.3, Problem 12.104P
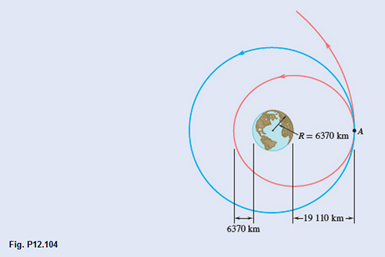
A satellite describes a circular orbit at an altitude of 19 110 km above the surface of the earth. Determine (a) the increase in speed required at point A for the satellite to achieve the escape velocity and enter a parabolic orbit, (b) the decrease in speed required at point A for the satellite to enter an elliptic orbit with a minimum altitude of 6370 km, (c) the eccentricity E of the elliptic orbit.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
How much energy per pound should be imparted to a satellite in order to place it in a circular orbit at an altitude of (a) 400 mi, (b) 4000 mi?
A satellite is in a circular earth orbit of radius min = 1.66R, where R is the radius of the earth. What is the minimum velocity boost Av
necessary to reach point B, which is a distance max = 3.94R from the center of the earth? At what point in the original circular orbit
should the velocity increment be added?
CO
Answer: Av = i
max
m/s
A satellite describes an elliptic orbit about a planet of mass M . The minimum and maximum values of the distance r from the satellite to the center of the planet are, respectively, r0 and r1. Use the principles of conservation of energy and conservation of angular momentum to derive the relationwhere h is the angular momentum per unit mass of the satellite and G is the constant of gravitation.
Chapter 12 Solutions
Vector Mechanics for Engineers: Dynamics
Ch. 12.1 - A 1000-Ib boulder B is resting on a 200-Ib...Ch. 12.1 - Marble A is placed in a hollow tube, and the tube...Ch. 12.1 - The two systems shown start from rest. On the...Ch. 12.1 - Prob. 12.CQ4PCh. 12.1 - People sit on a Ferris wheel at points A, B, C,...Ch. 12.1 - Crate A is gently placed with zero initial...Ch. 12.1 - Prob. 12.F2PCh. 12.1 - Objects A, B, and C have masses mA, mB, and...Ch. 12.1 - Blocks A and B have masses mAand mB, my...Ch. 12.1 - Blocks A and B have masses mAand mB, my...
Ch. 12.1 - A pilot of mass m flies a jet in a half-vertical...Ch. 12.1 - Wires AC and BC are attached to a sphere that...Ch. 12.1 - A collar of mass m is attached to a spring and...Ch. 12.1 - Four pins slide in four separate slots cut in a...Ch. 12.1 - At the instant shown, the length of the boom AB is...Ch. 12.1 - Prob. 12.F11PCh. 12.1 - Pin B has a mass m and slides along the slot in...Ch. 12.1 - Astronauts who landed on the moon during the...Ch. 12.1 - The value of g at any latitude o may be obtained...Ch. 12.1 - A 400-kg satellite has been placed in a circular...Ch. 12.1 - A spring scale A and a lever scale B having equal...Ch. 12.1 - In anticipation of a ling 7° upgrade, a bus driver...Ch. 12.1 - A 0.2-Ib model rocket is launched vertically from...Ch. 12.1 - A tugboat pulls a small barge through a harbor....Ch. 12.1 - Determine the maximum theoretical speed that may...Ch. 12.1 - If an automobile’s braking distance from 90km/h is...Ch. 12.1 - A mother and her child are skiing together, and...Ch. 12.1 - The coefficients of friction the load and the...Ch. 12.1 - A light train made up of two cars is traveling at...Ch. 12.1 - The two blocks shown are originally at rest....Ch. 12.1 - The two blocks shown are originally at rest....Ch. 12.1 - Each of the systems shown is initially at rest....Ch. 12.1 - Boxes A and B are at rest on a conveyor belt that...Ch. 12.1 - A 5000-1b truck is being used to lift a 1000-1b...Ch. 12.1 - Block A has a mass of 40 kg, and block B has a...Ch. 12.1 - Block A has a mass of 40 kg, and block B has a...Ch. 12.1 - Prob. 12.20PCh. 12.1 - Prob. 12.21PCh. 12.1 - To unload a bound stack of plywood from a truck;...Ch. 12.1 - To transport a series of bundles of shingles A to...Ch. 12.1 - Prob. 12.24PCh. 12.1 - Prob. 12.25PCh. 12.1 - Prob. 12.26PCh. 12.1 - A spring AB of constant k is attached to a support...Ch. 12.1 - Prob. 12.28PCh. 12.1 - Prob. 12.29PCh. 12.1 - An athlete pulls handle A to the left with a...Ch. 12.1 - A 10-Ib block B rests as shown on a 20-1b bracket...Ch. 12.1 - Prob. 12.32PCh. 12.1 - Knowing that k=0.30 , determine the acceleration...Ch. 12.1 - A 25-kg block A rests on an inclined surface, and...Ch. 12.1 - Block B of mass 10 kg rests as shown on the upper...Ch. 12.1 - A 450-g tetherball A is moving along a horizontal...Ch. 12.1 - During a hammer throwers practice swings. The...Ch. 12.1 - Prob. 12.38PCh. 12.1 - A single wire ACB passes through a ring at C...Ch. 12.1 - Two wires AC and BC are tied at C to a sphere that...Ch. 12.1 - A 1-kg sphere is at rest relative to parabolic...Ch. 12.1 - Prob. 12.42PCh. 12.1 - The 1.2-Ib flyballs of a centrifugal governor...Ch. 12.1 - A 130-ib wrecking ball B is attached to a...Ch. 12.1 - During a high-speed chase, a 2400-Ib sports car...Ch. 12.1 - An airline pilot climbs to a new flight level...Ch. 12.1 - The roller-coaster track shown is contained in a...Ch. 12.1 - A spherical-cap governor is fixed to a vertical...Ch. 12.1 - A series of small packages, each with a mass of...Ch. 12.1 - A 54-kg pilot flies a jet trainer in a...Ch. 12.1 - A carnival ride is designed to allow the general...Ch. 12.1 - Prob. 12.52PCh. 12.1 - Prob. 12.53PCh. 12.1 - Prob. 12.54PCh. 12.1 - A 3-kg block is at rest relative to a parabolic...Ch. 12.1 - A polisher is started so that the fleece along the...Ch. 12.1 - Prob. 12.57PCh. 12.1 - The carnival ride from Prob. 12.51 is modified so...Ch. 12.1 - Prob. 12.59PCh. 12.1 - Prob. 12.60PCh. 12.1 - Prob. 12.61PCh. 12.1 - Prob. 12.62PCh. 12.1 - Prob. 12.63PCh. 12.1 - A small 250-g collar C can slide on a semicircular...Ch. 12.1 - A small 250-g collar C can slide on a semicircular...Ch. 12.1 - An advanced spatial disorientation trainer allows...Ch. 12.1 - Prob. 12.67PCh. 12.1 - The 3-kg collar B slides on the frictionless arm...Ch. 12.1 - A 0.5-kg block B slides without friction inside a...Ch. 12.1 - Pin B weighs 4 oz and is free to slide in a...Ch. 12.1 - The two blocks are released from rest when r=0.8 m...Ch. 12.1 - Prob. 12.72PCh. 12.1 - Slider C has a weight of 0.5 Ib and may move in a...Ch. 12.2 - A particle of mass m is projected from point A...Ch. 12.2 - For the particle of Prob. 12.74, show (a) that the...Ch. 12.2 - Prob. 12.76PCh. 12.2 - For the particle of Prob. 12.76, determine the...Ch. 12.2 - Determine the mass of the earth knowing that the...Ch. 12.2 - Prob. 12.79PCh. 12.2 - Prob. 12.80PCh. 12.2 - Prob. 12.81PCh. 12.2 - The orbit of the planet Venus is nearly circular...Ch. 12.2 - A satellite is placed into a circular orbit about...Ch. 12.2 - The periodic time (see Prob. 12.83) of an earth...Ch. 12.2 - Prob. 12.85PCh. 12.2 - Prob. 12.86PCh. 12.2 - Prob. 12.87PCh. 12.2 - Prob. 12.88PCh. 12.2 - Prob. 12.89PCh. 12.2 - A 1 -kg collar can slide on a horizontal rod that...Ch. 12.2 - A 1-Ib ball A and a 2-Ib ball B are mounted on a...Ch. 12.2 - Two 2.6-Ib collars A and B can slide without...Ch. 12.2 - A small ball swings in a horizontal circle at the...Ch. 12.3 - A uniform crate C with mass m is being transported...Ch. 12.3 - A uniform crate C with mass m is being transported...Ch. 12.3 - A particle of mass m is projected from point A...Ch. 12.3 - A particle of mass m describes the logarithmic...Ch. 12.3 - Prob. 12.96PCh. 12.3 - Prob. 12.97PCh. 12.3 - Prob. 12.98PCh. 12.3 - It was observed that during the Galileo...Ch. 12.3 - Prob. 12.100PCh. 12.3 - Prob. 12.101PCh. 12.3 - Prob. 12.102PCh. 12.3 - Prob. 12.103PCh. 12.3 - A satellite describes a circular orbit at an...Ch. 12.3 - A space probe is to be placed in a circular orbit...Ch. 12.3 - Prob. 12.106PCh. 12.3 - Prob. 12.107PCh. 12.3 - Prob. 12.108PCh. 12.3 - Prob. 12.109PCh. 12.3 - Prob. 12.110PCh. 12.3 - Prob. 12.111PCh. 12.3 - Prob. 12.112PCh. 12.3 - Prob. 12.113PCh. 12.3 - Prob. 12.114PCh. 12.3 - Prob. 12.115PCh. 12.3 - Prob. 12.116PCh. 12.3 - Prob. 12.117PCh. 12.3 - A satellite describes an elliptic orbit about a...Ch. 12.3 - Prob. 12.119PCh. 12.3 - Prob. 12.120PCh. 12.3 - Show that the angular momentum per unit mass h of...Ch. 12 - In the braking test of a sports car, its velocity...Ch. 12 - A bucket is attached to a rope of length L=1.2 m...Ch. 12 - Prob. 12.124RPCh. 12 - Prob. 12.125RPCh. 12 - The roller-coaster track shown is contained in a...Ch. 12 - The parasailing system shown uses a winch to pull...Ch. 12 - Prob. 12.128RPCh. 12 - Telemetry technology is used to quantify kinematic...Ch. 12 - Prob. 12.130RPCh. 12 - Prob. 12.131RPCh. 12 - Prob. 12.132RPCh. 12 - Disk A rotates in a horizontal plane about a...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- A satellite is in a circular earth orbit of radius I'min = 1.67R, where R is the radius of the earth. What is the minimum velocity boost Av necessary to reach point B, which is a distance max = 2.68R from the center of the earth? At what point in the original circular orbit should the velocity increment be added? Answer: Av= i min max B m/sarrow_forwardDuring lecture we discussed that an elliptical orbit is not necessarily helpful to escapeEarth, and we said we would not investigate that further (but you are welcome on yourown).However, it is useful to investigate the radius of the “best” (ie, lowest Δv) circular parkingorbit. For this problem consider the following “steps” to Escape Earth:1. A Hohmann transfer from the surface to the parking orbit (i.e., 2 Δv’s).Assumptions:a. launch exactly from the equator with zero velocity relative to thegroundb. there is no atmosphere, mountains, obstacles, etc - the Δv canhappen in the tangential direction from the groundc. Simplify for now and use the Earth rotation = 1 revolution in 24hours2. A Δv from the parking orbit to escape Earth3. The target velocity is exactly vesc (i.e., there is no v∞ for a specificdestination, we just want to escape Earth)For all Δv’s you can ignore the direction, only consider magnitude.a. Develop an equation (or function in Matlab or a spreadsheet) which takes…arrow_forwardA space probe is to be placed in a circular orbit of 5600-mi radius about the planet Venus in a specified plane. As the probe reaches A, the point of its original trajectory closest to Venus, it is inserted in a first elliptic transfer orbit by reducing its speed by ΔvA. This orbit brings it to point B with a much reduced velocity. There the probe is inserted in a second transfer orbit located in the specified plane by changing the direction of its velocity and further reducing its speed by ΔvB. Finally, as the probe reaches point C, it is inserted in the desired circular orbit by reducing its speed by ΔvC. Knowing that the mass of Venus is 0.82 times the mass of the earth, that rA = 9.3 × 103 mi and rB = 190 × 103 mi, and that the probe approaches A on a parabolic trajectory, determine by how much the velocity of the probe should be reduced (a) at A, (b) at B, (c) at C.arrow_forward
- determine (a) the speed of the vehich as it approaches B on the elliptic path, (b) the amount by which its speed should be reduced as it approaches B to insert it into the smaller circular orbit.arrow_forwardWhile describing a circular orbit, 185 mi above the surface of the earth, a space shuttle ejects at point A an inertial upper stage (IUS) carrying a communications satellite to be placed in a geosynchronous orbit (see Prob. 13.87) at an altitude of 22,230 mi above the surface of the earth. Determine (a) the velocity of the IUS relative to the shuttle after its engine has been fired at A,( b) the increase in velocity required at B to place the satellite in its final orbit.Reference to Problem 13.87:arrow_forwardA space probe is to be placed in a circular orbit of radius 4o00 km about the planet Mars. As the probe reaches A, the point of its original trajectory closest to Mars, it is inserted into a first elliptic transfer orbit by reducing its speed. This orbit brings it to Point B with a much reduced velocity. There the probe is inserted into a second transfer orbit by further reducing its speed. Knowing that the mass of Mars is 0.1074 times the mass of the earth, that ra= 9004 km and rg= 180 004 km, and that the probe approaches A on a parabolic trajectory, determine the time needed for the space probe to travel from A to Bon its first transfer orbit. Approach trajectory Second transfer orbit В 4000 km First transfer orbit The time needed for the space probe to travel from A to B on its first transfer orbit is |h.arrow_forward
- I’m stuck on this problem. Please help with all parts especially starting with Newton’s law and sketching the situation. THANK YOUarrow_forwardA satellite used in a cellular telephone network has a mass of 2380 kg and is in a circular orbit at a height of 850 km above the surface of the earth. Part A What is the gravitational force Fgrav on the satellite? Take the gravitational constant to be G = 6.67x10-11 N-m2/kg2, the mass of the earth to be me = 5.97x1024 kg, and the radius of the Earth to be re = 6.38×106 marrow_forwardA spacecraft approaching the planet Saturn reaches point A with a velocity vA of magnitude 68.8 × 103 ft/s. It is to be placed in an elliptic orbit about Saturn so that it will be able to periodically examine Tethys, one of Saturn’s moons. Tethys is in a circular orbit of radius 183 × 103 mi about the center of Saturn, traveling at a speed of 37.2 × 103 ft/s. Determine (a) the decrease in speed required by the spacecraft at A to achieve the desired orbit, (b) the speed of the spacecraft when it reaches the orbit of Tethys at B.arrow_forward
- A satellite is in circular orbit around Earth at an altitude of three Earth radii above the surface. If the satellite uses onboard retro rockets to cut its speed to one half, at what distance from Earth can it establish a new stable circular orbit? Hint: vorbit =arrow_forward8.11 Estimate the total delta-v requirement for a Hohmann transfer from earth to Mercury, assuming a 150-km-altitude circular parking orbit at earth and a 150-km circular capture orbit at Mercury. Furthermore, assume that the planets have coplanar circular orbits with radii equal to the semimajor axes listed in Table A.1. {Ans.: 13.08 km/s}arrow_forwardDetermine the weight W of a satellite when it is in orbit 20.2(10^6)m above the surface of the earth if the satellite weighs 8450 N at the surface.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
Dynamics - Lesson 1: Introduction and Constant Acceleration Equations; Author: Jeff Hanson;https://www.youtube.com/watch?v=7aMiZ3b0Ieg;License: Standard YouTube License, CC-BY