Chapter 12.2, Problem 1PSA

a.

To determine

To calculate: The area of each lateral face of pyramid with triangular dimensions as 13 and 10.

Answer to Problem 1PSA

The area of each lateral face of pyramid is $60$.

Explanation of Solution

Given information:

A pyramid has triangular dimensions as 13 and 10.

Formula used:

The below theorem is used:

Pythagoras theorem states that “In a right angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides”.

  

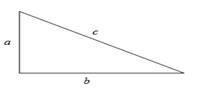
In right angle triangle,

  $a^2+b^2\text{= }{c}^2$

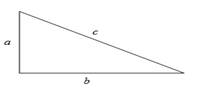
Area of triangle: $A=\frac{1}{2}\times b\times h$

b = base of triangle

h = height of triangle

Calculation:

  

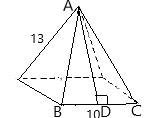
Draw altitude perpendicular to base.

The altitude AD can be calculated by applying Pythagoras Theorem.

In right angle triangle ADC , we get

  $\begin{array}{l}{\left(AD\right)}^2+{\left(DC\right)}^2={\left(AC\right)}^2\\ {\left(AD\right)}^2+{\left(5\right)}^2={\left(13\right)}^2\\ {\left(AD\right)}^2+25=169\\ {\left(AD\right)}^2=169-25\\ {\left(AD\right)}^2=144\\ AD=\sqrt{144}=12\end{array}$

The altitude drawn perpendicular divides the triangle face into two right triangles of $5-12-13$ family.

Lateral face is triangle.

Area of lateral face $=\frac{1}{2}\times 10\times 12$

Area of lateral face=60

b.

To determine

To find: The lateral area of pyramid with triangular dimensions as 13 and 10.

Answer to Problem 1PSA

The lateral area of pyramid is $240$ .

Explanation of Solution

Given information:

A pyramid has triangular dimensions as 13 and 10.

Formula used:

The below theorem is used:

Pythagoras theorem states that “In a right angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides”.

  

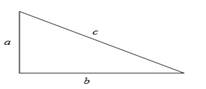
In right angle triangle,

  $a^2+b^2\text{= }{c}^2$

Area of triangle: $A=\frac{1}{2}\times b\times h$

b = base of triangle

h = height of triangle Lateral Area = Area of lateral face $\times$ Number of faces

Calculation:

  

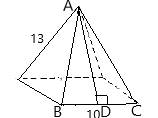
Draw altitude perpendicular to base.

The altitude AD can be calculated by applying Pythagoras Theorem.

In right angle triangle ADC , we get

  $\begin{array}{l}{\left(AD\right)}^2+{\left(DC\right)}^2={\left(AC\right)}^2\\ {\left(AD\right)}^2+{\left(5\right)}^2={\left(13\right)}^2\\ {\left(AD\right)}^2+25=169\\ {\left(AD\right)}^2=169-25\\ {\left(AD\right)}^2=144\\ AD=\sqrt{144}=12\end{array}$

The altitude drawn perpendicular divides the triangle face into two right triangles of $5-12-13$ family.

Lateral face is triangle.

Area of lateral face $=\frac{1}{2}\times 10\times 12$

Area of lateral face = 60

Lateral Area = Area of lateral face $\times$ Number of faces

Lateral Area = 60 $\times$ 4

Lateral Area = 240

c.

To determine

To calculate: The total area of pyramid with triangular dimensions as 13 and 10.

Answer to Problem 1PSA

The total area of pyramid is $340$ .

Explanation of Solution

Given information:

A pyramid has triangular dimensions as 13 and 10.

Formula used:

The below theorem is used:

Pythagoras theorem states that “In a right angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides”.

  

In right angle triangle,

  $a^2+b^2\text{= }{c}^2$

Area of triangle: $A=\frac{1}{2}\times b\times h$

b = base of triangle

h = height of triangle Area of square: $A=s^2$

s = side of square Lateral Area = Area of lateral face $\times$ Number of faces

Total area of pyramid = Area of square base + Lateral Area

Calculation:

  

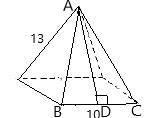
Draw altitude perpendicular to base.

The altitude AD can be calculated by applying Pythagoras Theorem.

In right angle triangle ADC , we get

  $\begin{array}{l}{\left(AD\right)}^2+{\left(DC\right)}^2={\left(AC\right)}^2\\ {\left(AD\right)}^2+{\left(5\right)}^2={\left(13\right)}^2\\ {\left(AD\right)}^2+25=169\\ {\left(AD\right)}^2=169-25\\ {\left(AD\right)}^2=144\\ AD=\sqrt{144}=12\end{array}$

The altitude drawn perpendicular divides the triangle face into two right triangles of $5-12-13$ family.

Lateral face is triangle.

Area of lateral face $=\frac{1}{2}\times 10\times 12$

Area of lateral face = 60

Lateral Area = Area of lateral face $\times$ Number of faces

Lateral Area = 60 $\times$ 4

Lateral Area = 240

Area of square base: $A={\left(10\right)}^2=100$

Total area of pyramid = Area of square base + Lateral Area

Total area of pyramid = 100 + 240

Total area of pyramid = 340