Chapter 1, Problem 14E

Consider a tapered bar of circular cross section. The length of the bar is 1 m, and the radius varies as $r\left(x\right)=0.050-0.04x$, where r and x are in meters. Assume Young’s modulus $=\text{1}00\text{MPa}$. Both ends of the bar are fixed, and $F=\text{1}0,000\text{N}$ is applied at the center. Determine the displacements, axial force distribution, and the wall reactions using four elements of equal length.

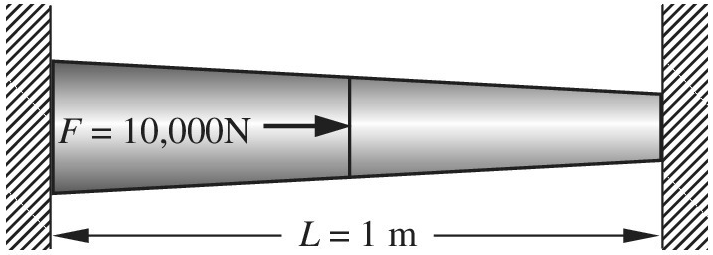
Hint: To approximate the area of cross section of a bar element, use the geometric mean of the end areas of the element, i.e., $A^{\left(e\right)}\sqrt{A_i{A}_j}=\pi r_i{r}_j$