Chapter 8, Problem 8.113P

A novel scheme for dissipating heat from the chips ofa multichip array involves machining coolant channelsin the ceramic substrate to which the chips areattached. The square chips ( $L_C=5\text{mm}$ ) are alignedabove each of the channels, with longitudinal andtransverse pitches of $S_L=S_T=20\text{mm}$ . Water flowsthrough the square cross section ( $\text{W }=\text{ 5 mm}$ ) of eachchannel with a mean velocity of $u_m=1\text{m}/\text{s}$ . and itsproperties may be approximated as $\rho =1000kg/m^3,c_p=4180\text{K/kg}\cdot \text{K,}\mu {\text{=855×10}}^{\text{-6}}\text{kg/s}\cdot \text{m,k}=0.610\text{W/m}\cdot \text{K}$ and $Pr=\text{ 5}.\text{8}$ . Symmetry in the transversedirection dictates the existence of equivalent conditionsfor each substrate section of length $L_S$ and width $S_T$ .

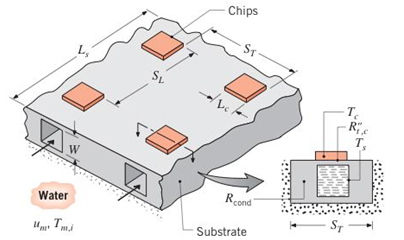
(a) Consider a substrate whose length in the flow direction is $L_S=200$ mm. thereby providing a total of $N_L=10$ chips attached in-line above each flow channel. To a good approximation, all the heat dissipated by the chips above a channel may be assumed to be transferred to the water flowing through the channel. If each chip dissipates 5 W. what is the temperature rise of the water passing through the channel?

(b) The chip-substrate contact resistance is $R_{i,c}^n=0.5\times {10}^{-4}{\text{m}}^2\cdot \text{K/W}$ , and the three-dimensional conduction resistance for the $L_S\times S_T$ substrate section is $R_{cond}=0.120\text{K/W}$ . If water enters the substrate at $\text{25}°\text{C}$ and is in hilly developed flow, estimate the temperature $T_c$ of the chips and the temperature $T_s$ of the substrate channel surface.