Chapter 8, Problem 5CT

To determine

The three remaining parts of the triangle below

  

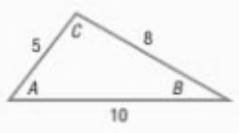
Answer to Problem 5CT

Solution:

Theangles of triangle are $A={52.41}^0,B={29.69}^0,$ and $C={97.9}^0.$

Explanation of Solution

Given information:

The figure of the triangle

  

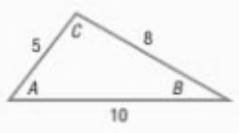
By applyingthe Law of cosines: The square of one side of a triangle equals the sum of squares of the other sides, minus twice their product times the cosine of their included angle.

To find angle $A$ :

  $\cos A=\frac{b^2+c^2-a^2}{2bc}$

  $\cos A=\frac{{\left(5\right)}^2+{\left(10\right)}^2-{\left(8\right)}^2}{2\cdot 5\cdot 10}=\frac{61}{100}.$

  $A={\cos }^{-1}\left(\frac{61}{100}\right)\approx {52.41}^0$ .

To find angle $B$ :

  $\cos B=\frac{a^2+c^2-b^2}{2ac}$

  $\cos B=\frac{{\left(8\right)}^2+{\left(10\right)}^2-{\left(5\right)}^2}{2\cdot 8\cdot 10}=\frac{139}{160}.$

  $B={\cos }^{-1}\left(\frac{139}{160}\right)\approx {29.69}^0$ .

Addition of angles of triangle $A,B,$ and $C$ is ${180}^0$ .

Now use angle $A$ and angle $B$ to find angle $C$ .

  $C={180}^0-A-B={180}^0-{52.41}^0-{29.69}^0={97.9}^0$

Therefore, the angles of the triangleare $A={52.41}^0,B={29.69}^0,$ and $C={97.9}^0.$