Chapter 8, Problem 16CT

To determine

The area of the entire region, rounded to two decimal places, shown in the

figure below which consists of an isosceles triangle $OAB$ and a semicircle.

  

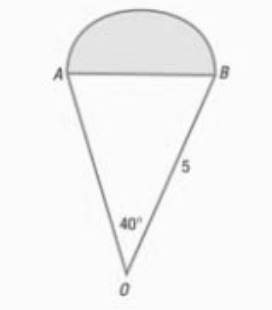
Answer to Problem 16CT

Solution:

The area of the entire region is $12.63$ square units.

Explanation of Solution

Given information:

Triangle $OAB$ is an isosceles triangle and the shaded sector is a semicircle as shown in the following figure:

  

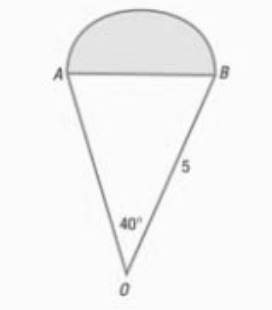
Draw a line on the diagram passing through point $O$, so that it is perpendicular to

the side $AB$, which meets at point $D$, such that it becomes the midpoint of the side $AB$ .

Hence, $AD=DB$

  

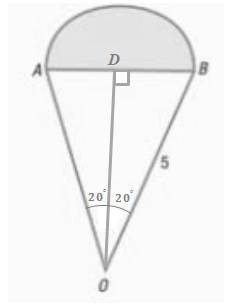
Consider the right angled triangle $ODB$, the length of side $BD$ is calculated by applying sine ratio.

  $\sin O=\frac{BD}{OB}$

By substituting the value of $OB=5$, and angle $O={20}^{\circ }$ in the sine ratio,

  $\sin {20}^{\circ }=\frac{BD}{5}$

  $BD=5\times \sin {20}^{\circ }$

  $BD=5\times 0.342020\approx 1.7101$

The length of side $BD$ is the radius of the semicircle.

The area of the semicircle is $A=\frac{1}{2}\pi r^2$

Substitute the value of $r=1.7101$ in the area formula of the semicircle.

  $A=\frac{1}{2}\pi {\left(1.7101\right)}^2$

  $\Rightarrow A=\frac{1}{2}\pi {\left(1.7101\right)}^2$

  $\Rightarrow A=\frac{1}{2}\pi \left(2.92444201\right)$

  $A=4.5937\approx 4.59$

Thus, the area of the shaded semicircle is $4.59$ square units.

As a triangle $OAB$ is a right angled triangle, so $OA=OB=5$

Use the formula for the area $K$ of a triangle $K=\frac{1}{2}bh$

The area of the isosceles triangle $OAB$ is $K=\frac{1}{2}\times OA\times OB\sin O$

By substituting the values of the sides of a triangle and the angle in the formula,

  $\Rightarrow K=\frac{1}{2}\times 5\times 5\sin {40}^{\circ }$

  $\Rightarrow K=12.5\times 0.64278761$

  $\Rightarrow K=8.0348\approx 8.035$

Thus, the area of the triangle $OAB$ is $8.035$ square units.

The area of the entire region is the shaded semicircle and the area of the isosceles triangle $OAB$

So, the total area is $4.59+8.035=12.625\approx 12.63$ square units.

Therefore, the area of the entire region is $12.63$ square units.