Chapter 6, Problem 6.35P

Interpretation Introduction

(a)

Interpretation:

The diameter of the tensile bar is 0.505in. From a Cu-Ni alloy, this tensile bar is machined to 2.00 in gage length. The following data is observed.

Load (lbs)	Gage length (in.)
0	2.00000
1000	2.00045
2000	2.00091
3000	2.00136
4000	2.0020
6000	2.020
8000	2.052
10,000	2.112
11,000	2.80 (maximum load)
9000	2.750 (fracture)

Gage length and diameter was 2.75 in. and 0.365 in. respectively after the fracture.

For the observed data engineering stress and strain, the curve is to be plotted. From the plot, 0.2% offset yield strength needs to be determined.

Concept Introduction:

Following formula will be used for the calculation-

  $\sigma (stress)=\frac{P}{A}$

  $A(area)=\frac{\pi d^2}{4}$

And

  $\epsilon (strain)=\frac{\delta l}{l}$

Answer to Problem 6.35P

Yield strength at 0.2 % offset is 28,000 psi.

Explanation of Solution

Diameter of bar = 0.505in

Therefore the area of cross-section of the bar is-

  $A(area)=\frac{\pi d^2}{4}$ ...... (1)

Where d is the diameter.

Put the value of d in (1):

  $A(area)=\frac{\pi {\left(0.505\right)}^2}{4}$

Area = 0.200296 in²

Stress is given as force per unit area. The mathematical expression is given below-

  $\sigma (stress)=\frac{P}{A}$ ...... (2)

Where s = stress

P= applied load

A = area

The strain is used to measure the amount of deformation caused by the applied load. The mathematical expression is given below-

  $\epsilon (strain)=\frac{\delta l}{l}$

Where, $\delta l=l_f-l_o$

So,

  $\epsilon (strain)=\frac{l_f-l_o}{l_o}$ ...... (3)

Where,

l_f = final length

l_o = initial length

Given value-

Length of bar or initial length = 2.00 in

  $\epsilon (strain)=\frac{l_f-2}{2}$

Tabulate form for stress and strain data-

  $$

Load (P) lb	Area in2	stress $\sigma (stress)=\frac{P}{A}$ psi	Gauge length ( lf) in.	Change in length ( $l_f-2$) in.	Strain   $\left(\frac{l_f-2}{2}\right)$
0	0.2002296	0	2.00000	0.00000	0
1,000	0.2002296	4992.61	2.00045	0.00045	0.000225
2,000	0.2002296	9985.22	2.00091	0.00091	0.000455
3,000	0.2002296	14977.83	2.00136	0.00136	0.00068
4,000	0.2002296	19970.44	2.0020	0.0020	0.0010
6,000	0.2002296	29955.66	2.020	0.020	0.01
8,000	0.2002296	39940.89	2.052	0.052	0.026
10,000	0.2002296	49926.11	2.112	0.112	0.056
11,000(max load)	0.2002296	54918.72	2.280	0.280	0.140
9,000(fracture)	0.2002296	44933.50	2.750	0.750	0.375

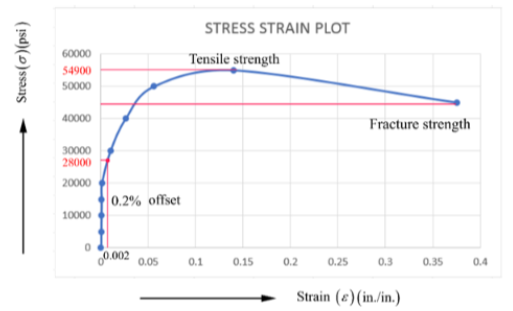
Stress-Strain curve based on above tabulated data-

  

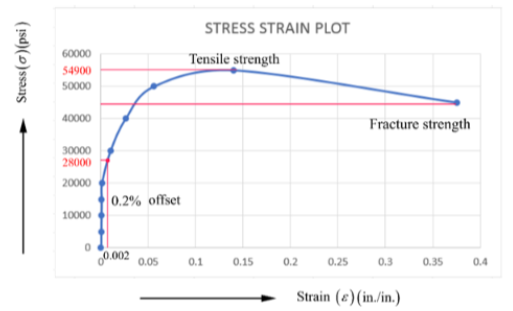
From the above plot 0.2% offset yield strength is calculated as follows-

For calculation draw a line which is parallel to the stress and strain and meets the curve at 0.2 % offset. The value of stress at 0.2% offset is called as yield strength which is 28,000 psi.

Yield strength at 0.2 % offset = 28,000 psi.

Interpretation Introduction

(b)

Interpretation:

The tensile strength of the bar needs to be calculated from observed data and engineering stress and strain curve.

Concept Introduction:

Following formula will be used for the calculation-

  $\sigma (stress)=\frac{P}{A}$

  $A(area)=\frac{\pi d^2}{4}$

And

  $\epsilon (strain)=\frac{\delta l}{l}$

Answer to Problem 6.35P

Tensile strength of the bar is 54900 psi from the stress-strain curve.

Explanation of Solution

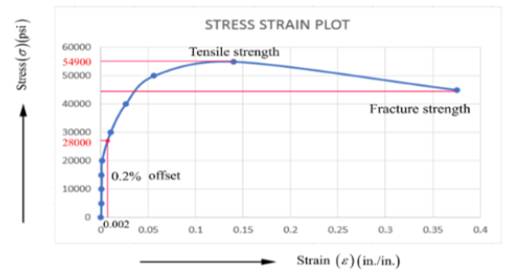
Stress-Strain curve based on above tabulated data of section (a)-

  

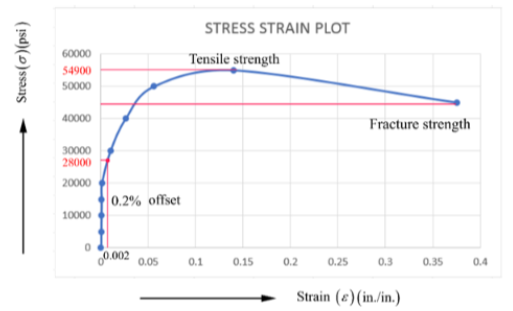
Tensile strength of the material is the ability of the material to withstand the pulling force.

From the above plot, the obtained tensile strength value is 54900 psi.

Tensile strength = 54900 psi.

Interpretation Introduction

(c)

Interpretation:

The modulus of elasticity of bar needs to be calculated from observed data and engineering stress and strain curve.

Concept Introduction:

Following formula will be used for the calculation-

  $\sigma (stress)=\frac{P}{A}$

  $A(area)=\frac{\pi d^2}{4}$

And

  $\epsilon (strain)=\frac{\delta l}{l}$

  $E\left(modulusofelasticity\right)=\frac{\sigma }{\epsilon }$

Answer to Problem 6.35P

Modulus of elasticity of bar is $21.94\times {10}^{6}psi$.

Explanation of Solution

Load (P) lb	Area in2	stress $\sigma (stress)=\frac{P}{A}$ psi	Gauge length ( lf) in.	Change in length ( $l_f-2$) in.	Strain   $\left(\frac{l_f-2}{2}\right)$
0	0.2002296	0	2.00000	0.00000	0
1,000	0.2002296	4992.61	2.00045	0.00045	0.000225
2,000	0.2002296	9985.22	2.00091	0.00091	0.000455
3,000	0.2002296	14977.83	2.00136	0.00136	0.00068
4,000	0.2002296	19970.44	2.0020	0.0020	0.0010
6,000	0.2002296	29955.66	2.020	0.020	0.01
8,000	0.2002296	39940.89	2.052	0.052	0.026
10,000	0.2002296	49926.11	2.112	0.112	0.056
11,000(max load)	0.2002296	54918.72	2.280	0.280	0.140
9,000(fracture)	0.2002296	44933.50	2.750	0.750	0.375

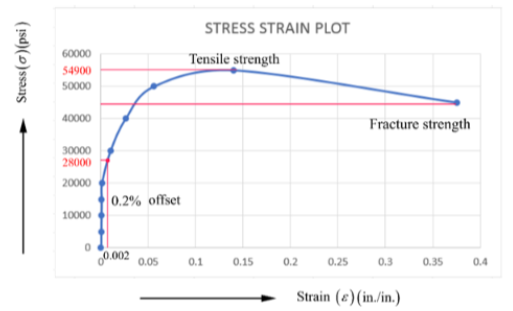
Stress-Strain curve based on tabulating data of section (a)-

  

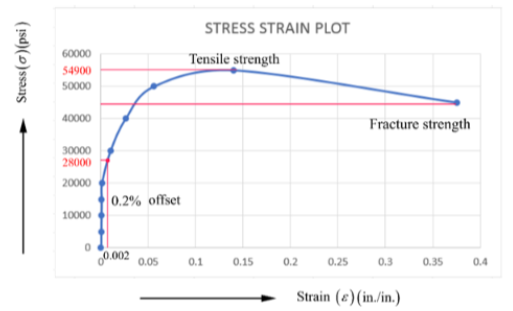
Modulus of elasticity is obtained by the Hooke's law. It is the ratio of stress and strain which is given as-

  $E=\frac{\sigma }{\epsilon }$ .........(1)

From the table-

s = 9985.22 psi

e = 0.000455

Put the above values in (1)

  $E=\frac{9985.22psi}{0.000455}$

  $E=21.94\times {10}^{6}psi$

Modulus of elasticity = $21.94\times {10}^{6}psi$.

Interpretation Introduction

(d)

Interpretation:

The percentage of elongation of the bar needs to be calculated from observed data and engineering stress and strain curve.

Concept Introduction:

Following formula will be used for calculation-

  $\sigma (stress)=\frac{P}{A}$

  $A(area)=\frac{\pi d^2}{4}$

And

  $\epsilon (strain)=\frac{\delta l}{l}$

  $\%elongation=\frac{\left(l_f-l_o\right)}{l_o}\times 100$

Answer to Problem 6.35P

The percentage of elongation of the bar by observed data and engineering stress and strain curve is 37.5%.

Explanation of Solution

Load (P) lb	Area in2	stress $\sigma (stress)=\frac{P}{A}$ psi	Gauge length ( lf) in.	Change in length ( $l_f-2$) in.	Strain   $\left(\frac{l_f-2}{2}\right)$
0	0.2002296	0	2.00000	0.00000	0
1,000	0.2002296	4992.61	2.00045	0.00045	0.000225
2,000	0.2002296	9985.22	2.00091	0.00091	0.000455
3,000	0.2002296	14977.83	2.00136	0.00136	0.00068
4,000	0.2002296	19970.44	2.0020	0.0020	0.0010
6,000	0.2002296	29955.66	2.020	0.020	0.01
8,000	0.2002296	39940.89	2.052	0.052	0.026
10,000	0.2002296	49926.11	2.112	0.112	0.056
11,000(max load)	0.2002296	54918.72	2.280	0.280	0.140
9,000(fracture)	0.2002296	44933.50	2.750	0.750	0.375

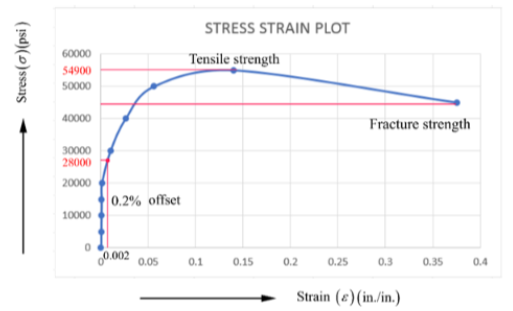
Stress-Strain curve on the basis of tabulating data of section (a)-

  

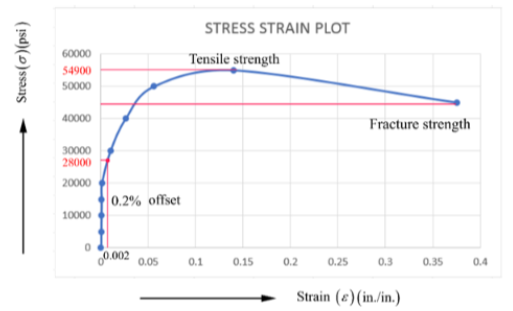
The percentage of elongation is the amount of deformation in the length of the bar.

It is calculated by using the following formula-

  $\%\text{ elongation}=\frac{\left(l_f-l_o\right)}{l_o}\times 100$ ............ (1)

From table- l_f ( final length) or fracture length = 2.75 in.

And given value −

l_o( initial length) = 2 in.

Put the above values in Equ (1)

  $\%elongation=\frac{\left(2.75-2\right)}{2}\times 100$

  $\%\text{ elongation}=37.5\%$

Interpretation Introduction

(e)

Interpretation:

The percentage of reduction in the area of the bar needs to be calculated from observed data and engineering stress and strain curve.

Concept Introduction:

Following formula will be used for the calculation-

  $\sigma (\text{stress)}=\frac{P}{A}$

  $A\text{(area})=\frac{\pi d^2}{4}$

And

  $\epsilon (\text{strain)}=\frac{\delta l}{l}$

  $\%\text{ reduction in area}=\frac{\left(A_o-A_f\right)}{A_o}\times 100$

  $A(\text{area})=\frac{\pi d^2}{4}$

Answer to Problem 6.35P

The percentage of reduction in the area of the bar by using observed data and engineering stress and strain curve is 47.76%.

Explanation of Solution

Load (P) lb	Area in2	stress $\sigma (stress)=\frac{P}{A}$ psi	Gauge length ( lf) in.	Change in length ( $l_f-2$) in.	Strain $\left(\frac{l_f-2}{2}\right)$
0	0.2002296	0	2.00000	0.00000	0
1,000	0.2002296	4992.61	2.00045	0.00045	0.000225
2,000	0.2002296	9985.22	2.00091	0.00091	0.000455
3,000	0.2002296	14977.83	2.00136	0.00136	0.00068
4,000	0.2002296	19970.44	2.0020	0.0020	0.0010
6,000	0.2002296	29955.66	2.020	0.020	0.01
8,000	0.2002296	39940.89	2.052	0.052	0.026
10,000	0.2002296	49926.11	2.112	0.112	0.056
11,000(max load)	0.2002296	54918.72	2.280	0.280	0.140
9,000(fracture)	0.2002296	44933.50	2.750	0.750	0.375

Stress-Strain curve on the basis of tabulating data of section (a)-

  

% reduction in the area of the bar is determined by using the following formula which is given as-

  $\%\text{ reduction in area}=\frac{\left(A_o-A_f\right)}{A_o}\times 100$

Where,

A_o = initial area

A_f = final area

  $A(area)=\frac{\pi d^2}{4}$

Where d is the diameter of the bar.

  $\%Are{a}_{\text{reduction}}=\frac{\left(\frac{\pi d_o^2}{4}-\frac{\pi d_f^2}{4}\right)}{\frac{\pi d_o^2}{4}}\times 100$ ......... Equ(1)

From observed table-

d_f = 0.365 in.

Given value-

d_o = 0.505 in.

Put the above values in Equ (1) :

  $\%Are{a}_{reduction}=\frac{\left(\frac{\pi \times {\left(0.505in\right)}^2}{4}-\frac{\pi \times {\left(0.365in\right)}^2}{4}\right)}{\frac{\pi \times {\left(0.505in\right)}^2}{4}}\times 100$

  $\%Are{a}_{reduction}=47.76\%$

Interpretation Introduction

(f)

Interpretation:

The engineering stress at the fracture needs to be calculated from observed data and engineering stress and strain curve.

Concept Introduction:

Following formula will be used for the calculation-

  $\sigma (stress)=\frac{P}{A}$

  $A(area)=\frac{\pi d^2}{4}$

And

  $\epsilon (strain)=\frac{\delta l}{l}$

Answer to Problem 6.35P

The engineering stress at the fracture is 44900 psi obtained from the plot.

Explanation of Solution

Stress-Strain curve on the basis of tabulating data of section (a)-

  

Engineering stress is defined as the force per unit area. The mathematical expression of engineering stress is given as-

  $\sigma (stress)=\frac{P}{A}$

From the plot of engineering stress and strain curve, the value of stress at the fracture is 44900 psi.

Interpretation Introduction

(g)

Interpretation:

The true stress at necking of bar is needs to be calculated from observed data and engineering stress and strain curve.

Concept Introduction:

Following formula will be used for the calculation-

  $\sigma (stress)=\frac{P}{A}$

  $A(area)=\frac{\pi d^2}{4}$

  $\epsilon (strain)=\frac{\delta l}{l}$

  $\epsilon =\frac{l-l_o}{l_o}$

The Relation between true stress and strain is-

  $\sigma =\frac{P}{A_O}(1+\epsilon )$

Answer to Problem 6.35P

Stress at the necking of bar is 61783 psi.

Explanation of Solution

Load (P) lb	Area in2	stress $\sigma (stress)=\frac{P}{A}$ psi	Gauge length ( lf) in.	Change in length ( $l_f-2$) in.	Strain $\left(\frac{l_f-2}{2}\right)$
0	0.2002296	0	2.00000	0.00000	0
1,000	0.2002296	4992.61	2.00045	0.00045	0.000225
2,000	0.2002296	9985.22	2.00091	0.00091	0.000455
3,000	0.2002296	14977.83	2.00136	0.00136	0.00068
4,000	0.2002296	19970.44	2.0020	0.0020	0.0010
6,000	0.2002296	29955.66	2.020	0.020	0.01
8,000	0.2002296	39940.89	2.052	0.052	0.026
10,000	0.2002296	49926.11	2.112	0.112	0.056
11,000(max load)	0.2002296	54918.72	2.280	0.280	0.140
9,000(fracture)	0.2002296	44933.50	2.750	0.750	0.375

Stress-Strain curve on the basis of tabulating data of section (a)-

  

Strain the maximum load is −

  $\epsilon =\frac{l-l_o}{l_o}$ ........... Equ(1)

l = guage length

l_o = initial length

From table-

l = 2.75 in.

Given value

l_o = 2.00 in.

Put the above values in Equ(1)

  $\epsilon =\frac{2.75-2}{2}$

  $\epsilon =0.375$

True stress at the necking is calculated by −

  $\sigma =\frac{P}{A_O}(1+\epsilon )$

  $A=\frac{\pi d_o^2}{4}$

  $\sigma =\frac{4P}{\pi d_o^2}(1+\epsilon )$ ............ Equ (2)

P = 9000 lbs (from table)

  $\epsilon =0.375$ (as, calculated)

d_o = 0.505 in.

Put the above values in Equ (2)$\sigma =\frac{4\times 9000}{\pi {\left(0.505\right)}^2}(1+0.375)$

  $\sigma =61783psi$

Stress at the necking = $\sigma =61783psi$

Interpretation Introduction

(h)

Interpretation:

The modulus of resilience of bar needs to be calculated from observed data and engineering stress and strain curve.

Concept Introduction:

Following formula will be used for the calculation-

  $\sigma (stress)=\frac{P}{A}$

  $A(area)=\frac{\pi d^2}{4}$

And

  $\epsilon (strain)=\frac{\delta l}{l}$

  $Modulusofresilience=\frac{1}{2}\times {\sigma }_y\times {\epsilon }_y$

Answer to Problem 6.35P

The modulus of resilience of bar is 10 psi.

Explanation of Solution

Load (P) lb	Area in2	stress $\sigma (stress)=\frac{P}{A}$ psi	Gauge length ( of) in.	Change in length ( $l_f-2$) in.	Strain $\left(\frac{l_f-2}{2}\right)$
0	0.2002296	0	2.00000	0.00000	0
1,000	0.2002296	4992.61	2.00045	0.00045	0.000225
2,000	0.2002296	9985.22	2.00091	0.00091	0.000455
3,000	0.2002296	14977.83	2.00136	0.00136	0.00068
4,000	0.2002296	19970.44	2.0020	0.0020	0.0010
6,000	0.2002296	29955.66	2.020	0.020	0.01
8,000	0.2002296	39940.89	2.052	0.052	0.026
10,000	0.2002296	49926.11	2.112	0.112	0.056
11,000(max load)	0.2002296	54918.72	2.280	0.280	0.140
9,000(fracture)	0.2002296	44933.50	2.750	0.750	0.375

Stress-Strain curve on the basis of tabulating data of section (a)-

  

The modulus of resilience can be explained as the amount of maximum energy absorbed by the material without any permanent deformation.

It is given as-

  $\text{Modulus of resilience}=\frac{1}{2}\times {\sigma }_y\times {\epsilon }_y$ ......... Eq (1)

Where,

s_y = yield stress

e_y = yield strain

From the plot-

s_y = 2000 psi

e_y = 0.001

Put above values in Eq (1)

  $\text{Modulus of resilience}=\frac{1}{2}\times 2000\times 0.001$

  $\text{Modulus of resilience}=10psi$