Chapter 6, Problem 6.37P

Interpretation Introduction

(a)

Interpretation:

The engineering stress-strain curve and true stress-strain curve should be plotted with the use of data provided of cold-rolled and annealed brass.

Concept Introduction:

Engineering stress is a term explained as a force or applied load on the given object's initial cross-sectional area and it is also known as nominal stress.

Engineering strain is a term representing the amount of deformation of the material in the direction of force applied divided by the initial material length.

True stress is the required amount of force that tends to deformation of the specimen.

True strain or reduction of area are defined as the length change of the specimen due to the applied force on it.

Answer to Problem 6.37P

Below graph represents the engineering stress-strain curve and true stress-strain curve given sample of cold-rolled and annealed brass.

  

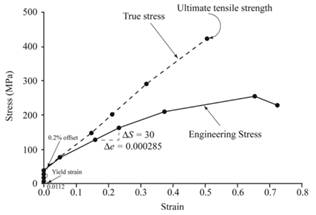
Explanation of Solution

The tabular data providing details about the load and length difference for given sample is as follows:

Table:

Load(N)	$\Delta l$ (mm)
0	0.00000
66	0.0112
177	0.0157
327	0.0199
462	0.0240
797	1.72
1350	5.55
1720	8.15
2220	13.07
2690	22.77 (maximum load)
2410	25.25 (fracture)

Let us calculate the engineering stress for the sample with the help of below formula:

$\begin{array}{l}S=\frac{F}{A_0}\\ S=\frac{66\text{N}}{10.5}\text{}{\text{(Putitng values of F=66 N and A}}_0=10.5m{m}^2)\\ S=6.29\text{}\text{MPa}\end{array}$

For determining the engineering strain of the given sample,

$\begin{array}{l}E=\frac{\Delta l}{l_0}\\ E=\frac{0.0112}{35}\text{ (}\Delta \text{l=0}{\text{.0112 mm and l}}_0=35mm)\\ E=0.00032\end{array}$

Now, find true strain as well as true stress with the help for below formulas:

1. True strain:

$\begin{array}{l}\epsilon =\ln \left(\frac{l_0+\Delta l}{l_0}\right)\\ \epsilon =\ln \left(\frac{35+0.0112}{35}\right)\\ \epsilon =\ln \left(1.00032\right)\\ \epsilon =0.00032\end{array}$

1. True stress:

$\begin{array}{l}{\sigma }_T=\frac{F}{A_0}\left(1+\epsilon \right)\\ {\sigma }_T=\frac{66}{10.5}\left(1+0.000320\right)\\ {\sigma }_T=6.29\left(1.00032\right)\\ {\sigma }_T=6.29\text{ MPa}\end{array}$

Tabulate the data with the use of engineering stress, engineering strain, true strain and true stress as below:

Load(N)	Displacement ( $\Delta l$) mm	Engineering stress (S) MPa	Engineering strain (e)	True strain ( $\epsilon$)	True stress( ${\sigma }_T$) MPa
0	0.00000	0	2	0	0
66	0.0112	6.29	0.00032	0.00032	6.29
177	0.0157	16.86	0.00044	0.00044	16.87
327	0.0199	31.13	0.00056	0.00056	31.16
462	0.0240	44	0.00068	0.00068	43
797	1.72	75.90	0.04914	0.04797	79.54
1350	5.55	129	0.15857	0.14718	148
1720	8.15	164	0.23285	0.20932	198
2220	13.07	211	0.37342	0.31732	279
2690	22.77	256	0.65056	0.50111	423
2410	25.25	230	0.72142	0.54315	355

Now, plot the true stress-strain curve and engineering stress-strain curve from the above gathered tabular data as below:

  

Interpretation Introduction

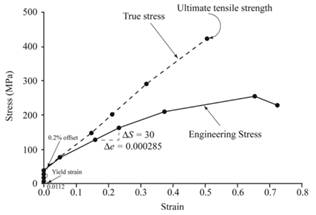
(b)

Interpretation:

With the help of plotted engineering stress-strain curve and true stress-strain curve, the explanation should be given for relative values of true stress-strain and engineering stress-strain.

Concept Introduction:

Engineering stress is a term explained as a force or applied load on the given object's initial cross-sectional area and it is also known as nominal stress.

Engineering strain is a term representing the amount of deformation of the material in the direction of force applied divided by the initial material length.

True stress is the required amount of force that tends to deformation of the specimen.

True strain or reduction of area and defined as the length change of the specimen due to the applied force on it.

Answer to Problem 6.37P

At point of elastic loading and necking, less value of engineering stress and true strain is obtained while high value of true stress and engineering strain is obtained.

Explanation of Solution

Below graph represents the engineering stress-strain curve and true stress-strain curve given sample of cold-rolled and annealed brass.

  

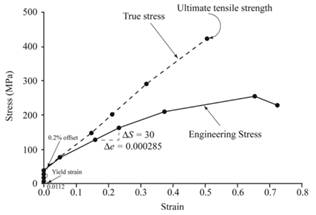
When there is a phase of elastic formation, the values of engineering stress-strain and true stress-strain are found closer to each other but after reviewing closely, one can justify that the values of true stress are higher than engineering stress values while true strain values are lower than engineering strain values. At the point of yielding phase, the differences of values become higher.

When tension test is performed, the cross-section area of sample getting reduced and there is an elongation of length determined by using instantaneous length changes. This result into high value of engineering strain as compared to true strain and low values of engineering stress compared to true stress prior to necking.

Interpretation Introduction

(c)

Interpretation:

The explanation should be given for the curve if prior known data was provided for true stress and strain at the point of necking.

Concept Introduction:

Modulus of elasticity is also known as coefficient of elasticity or elastic modulus and can be defined as the ratio of the stress in the given object body to the corresponding strain.

Answer to Problem 6.37P

If prior known data was provided for true stress and strain at the point of necking, one gets the continuously rising curve of true stress without indicating any highest point in this curve.

Explanation of Solution

The tabular data with the use of engineering stress, engineering strain, true strain and true stress is as below:

Load(N)	Displacement ( $\Delta l$) mm	Engineering stress (S) MPa	Engineering strain (e)	True strain ( $\epsilon$)	True stress( ${\sigma }_T$) MPa
0	0.00000	0	2	0	0
66	0.0112	6.29	0.00032	0.00032	6.29
177	0.0157	16.86	0.00044	0.00044	16.87
327	0.0199	31.13	0.00056	0.00056	31.16
462	0.0240	44	0.00068	0.00068	43
797	1.72	75.90	0.04914	0.04797	79.54
1350	5.55	129	0.15857	0.14718	148
1720	8.15	164	0.23285	0.20932	198
2220	13.07	211	0.37342	0.31732	279
2690	22.77	256	0.65056	0.50111	423
2410	25.25	230	0.72142	0.54315	355

The known value of true stress and strain may have not given us the curve of true stress-strain with the highest point and rather it would have shown continuous rise in the true stress.

Interpretation Introduction

(d)

Interpretation:

With the help of plotted engineering and true stress-strain curve, the 0.2 % offset yield strength should be calculated for the given data of cold-rolled and annealed brass.

Concept Introduction:

The maximum amount of elastic deformation which is bearable by any material is defined as yield strength.

Answer to Problem 6.37P

The yield strength for 0.2% offset is 45 MPa for a given sample of cold-rolled and annealed brass.

Explanation of Solution

The tabular data with the use of engineering stress, engineering strain, true strain and true stress is as below:

Load(N)	Displacement ( $\Delta l$) mm	Engineering stress (S) MPa	Engineering strain (e)	True strain ( $\epsilon$)	True stress( ${\sigma }_T$) MPa
0	0.00000	0	2	0	0
66	0.0112	6.29	0.00032	0.00032	6.29
177	0.0157	16.86	0.00044	0.00044	16.87
327	0.0199	31.13	0.00056	0.00056	31.16
462	0.0240	44	0.00068	0.00068	43
797	1.72	75.90	0.04914	0.04797	79.54
1350	5.55	129	0.15857	0.14718	148
1720	8.15	164	0.23285	0.20932	198
2220	13.07	211	0.37342	0.31732	279
2690	22.77	256	0.65056	0.50111	423
2410	25.25	230	0.72142	0.54315	355

Below graph represents the engineering stress-strain curve and true stress-strain curve given sample of cold-rolled and annealed brass.

  

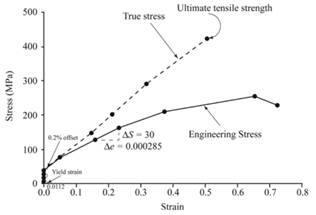
The above graph can provide the value of yield strength for 0.2% offset as 45 MPa.

Therefore, one can conclude that the given sample contains the yield strength for 0.2% offset as 45MPa.

Interpretation Introduction

(e)

Interpretation:

With the help of plotted engineering and true stress-strain curve, the tensile strength should be calculated for the given data of cold-rolled and annealed brass.

Concept Introduction:

The tensile strength can be defined as the measurement of maximum deformation which can be bearable by any material without undergoing necking condition.

Answer to Problem 6.37P

The tensile strength is 256.1MPa for a given sample of cold-rolled and annealed brass.

Explanation of Solution

The tabular data with the use of engineering stress, engineering strain, true strain and true stress is as below:

Load(N)	Displacement ( $\Delta l$) mm	Engineering stress (S) MPa	Engineering strain (e)	True strain ( $\epsilon$)	True stress( ${\sigma }_T$) MPa
0	0.00000	0	2	0	0
66	0.0112	6.29	0.00032	0.00032	6.29
177	0.0157	16.86	0.00044	0.00044	16.87
327	0.0199	31.13	0.00056	0.00056	31.16
462	0.0240	44	0.00068	0.00068	43
797	1.72	75.90	0.04914	0.04797	79.54
1350	5.55	129	0.15857	0.14718	148
1720	8.15	164	0.23285	0.20932	198
2220	13.07	211	0.37342	0.31732	279
2690	22.77	256	0.65056	0.50111	423
2410	25.25	230	0.72142	0.54315	355

Below graph represents the engineering stress-strain curve and true stress-strain curve given sample of cold-rolled and annealed brass.

  

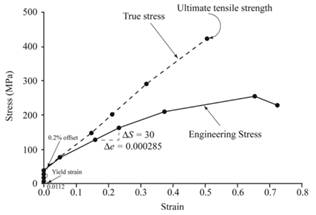
The tensile strength can be determined by use of below mentioned formula:

$\begin{array}{l}\text{ Tensile strength =}\frac{F_{\max imum\text{}\text{load}}}{A_0}\\ \text{ Tensile strength =}\frac{2690}{10.5}\\ \text{ Tensile strength =256}\text{.1}\text{}\text{MPa}\end{array}$

Therefore, one can conclude that the given sample of cold-rolled and annealed brass has the tensile strength of 256.1MPa.

Interpretation Introduction

(f)

Interpretation:

With the help of plotted engineering and true stress-strain curve, the value of modulus of elasticity should be calculated for the given data of cold-rolled and annealed brass.

Concept Introduction:

Modulus of elasticity is also known as coefficient of elasticity or elastic modulus and can be defined as the ratio of the stress in the given object body to the corresponding strain.

Answer to Problem 6.37P

The value of modulus of elasticity is 105.2GPafor a given sample of cold-rolled and annealed brass.

Explanation of Solution

The tabular data with the use of engineering stress, engineering strain, true strain and true stress is as below:

Load(N)	Displacement ( $\Delta l$) mm	Engineering stress (S) MPa	Engineering strain (e)	True strain ( $\epsilon$)	True stress( ${\sigma }_T$) MPa
0	0.00000	0	2	0	0
66	0.0112	6.29	0.00032	0.00032	6.29
177	0.0157	16.86	0.00044	0.00044	16.87
327	0.0199	31.13	0.00056	0.00056	31.16
462	0.0240	44	0.00068	0.00068	43
797	1.72	75.90	0.04914	0.04797	79.54
1350	5.55	129	0.15857	0.14718	148
1720	8.15	164	0.23285	0.20932	198
2220	13.07	211	0.37342	0.31732	279
2690	22.77	256	0.65056	0.50111	423
2410	25.25	230	0.72142	0.54315	355

Below graph represents the engineering stress-strain curve and true stress-strain curve given sample of cold-rolled and annealed brass.

  

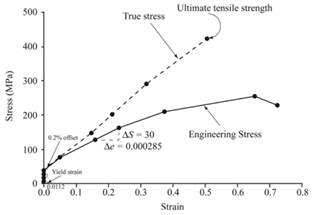
The modulus of elasticity can be determined by use of below mentioned formula:

$E=\frac{\Delta S}{\Delta e}\mathrm{......}(3)$

In above equation, $\Delta S$ is the stress related ordinate of slope and $\Delta e$ is strain related ordinate of slope. Putting values of $\Delta S$ and $\Delta e$ as 50 MPa and 0.0011 respectively in above equation (3).

$\begin{array}{l}E=\frac{\Delta S}{\Delta e}\\ E=\frac{30}{0.000285}\\ E=\left(\text{105,263MPa}\right)\text{}\left(\frac{1\text{}\text{GPa}}{1,000\text{ MPa}}\right)\\ E=105.2\text{ GPa}\end{array}$

Therefore, the value of modulus of elasticity for given cold-rolled and annealed brassas 105.2 GPa.