
Concept explainers
To find: the rate at which the length of the person’s shadow is changing when he is
Answer to Problem 29E
The rate at which the length of the person’s shadow is changing is
Explanation of Solution
Given information :
Streetlight
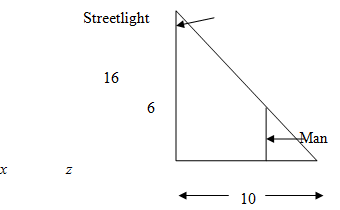
From the given data in the problem, we draw a diagram. The diagram looks like two triangles. When any two triangles are similar, the ratio between any two matching sides, two sides opposite an angle of the same degree is equal to the ratio of any two other matching sides. Therefore, two matching sides is
All variables are differentiable functions of t .
Calculation:
We have to calculate the rate at which the length of the person’s shadow is changing when he is
So,
Differentiate with respect to t .
Putting the value of
Hence, the rate at which the length of the person’s shadow is changing is
Chapter 5 Solutions
Calculus 2012 Student Edition (by Finney/Demana/Waits/Kennedy)
Additional Math Textbook Solutions
Elementary Statistics
Introductory Statistics
Elementary Statistics: Picturing the World (7th Edition)
Algebra and Trigonometry (6th Edition)
College Algebra (7th Edition)
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





