Chapter 5.4, Problem 65E

a.

To determine

To show: that the area of the triangle is:

  $A(x)=-f\text{'}(x){\left[x-\frac{f(x)}{f\text{'}(x)}\right]}^2,\text{where}y=f(x)$ is the function representing the upper half of the ellipse.

Explanation of Solution

Given information: Let P(x, a) and Q (-x, a ) be two points on the upper half of the ellipse.

  $\frac{x^2}{100}+\frac{{(y-5)}^2}{25}=1$ .

Centered at (0, 5). A triangle RST is formed by using the tangent lines to the ellipse at Q and P.

Calculation:

Let $x_0$ be some fixed value of x for points (x. a) and (-x, a) . P then has coordinates of ( $x_0$ ,a) . The slope of the tangent line passing through P is then f’ ( $x_0$ ). Substitute f’( $x_0$ ) and the point ( $x_0$ ,a) into the point-slope form of a line to write the equation of the tangent line.

  $y-y_1=m(x-x_1)$

  $y-a=f\text{'}(x_0)(x-x_0)$

The height h of the triangle is the y- intercept of the tangent line so substitute in x =0 into the tangent line equation to find the y-intercept. The height of the triangle is then;

  $h=a-x_{0}f\text{'}(x_0)$

  $△SRT$ is isosceles so the base of the triangle is 2 times the x-intercept of the tangent line. Substitute in y = 0 into the tangent line equation to find the x-intercept. The base of the triangle is then

  $\begin{array}{c}0-a=f\text{'}(x_0)(x-x_0)\\ -a=xf\text{'}(x_0)-x_{0}f\text{'}(x_0)\\ x=\frac{2(x_{0}f\text{'}(x_0)-a)}{f\text{'}(x_0)}\\ b=\frac{2(x_{0}f\text{'}(x_0)-a)}{f\text{'}(x_0)}\end{array}$

  $\begin{array}{c}A=\frac{1}{2}bh\\ =\frac{1}{2}\left(\frac{2(x_{0}f\text{'}(x_0)-a)}{f\text{'}(x_0)}\right)(a-x_{0}f\text{'}(x_0))\\ =\frac{(x_{0}f\text{'}(x_0)-a)}{f\text{'}(x_0)}.\frac{(x_{0}f\text{'}(x_0)-a)}{f\text{'}(x_0)}(-f\text{'}(x_0))\\ =-f\text{'}(x_0)\frac{{(x_{0}f\text{'}(x_0)-a)}^2}{f\text{'}(x_0)}\\ =-f\text{'}(x_0){\left(x_0-\frac{a}{f\text{'}(x_0)}\right)}^2\\ A(x)=-f\text{'}(x){\left(x-\frac{a}{f\text{'}(x)}\right)}^2\end{array}$

Hence proved.

b.

To determine

To find: the domain of A and draw the graph of A and how are the asymptotes of the graph related to the problem situation.

Answer to Problem 65E

  $\text{Domain}\{x\in R:-10<x<10,x\ne 0\}$ .

Asymptotes: x$=\pm 10$ .

Explanation of Solution

Given information: Let P(x, a) and Q (-x, a ) be two points on the upper half of the ellipse.

  $\frac{x^2}{100}+\frac{{(y-5)}^2}{25}=1$ .

Centered at (0, 5). A triangle RST is formed by using the tangent lines to the ellipse at Q and P.

Calculation:

  $\begin{array}{c}\frac{x^2}{100}+\frac{{(y-5)}^2}{25}=1\\ \frac{{(y-5)}^2}{25}=1-\frac{x^2}{100}\\ $ {(y-5)}^2=25-\frac{x^2}{4}$y-5=\sqrt{25-\frac{x^2}{4}}\\ y=5+\sqrt{25-\frac{x^2}{4}}\\ f(x)=5+\sqrt{25-\frac{x^2}{4}}\\ f\text{'}(x)=\frac{1}{2}{\left(25-\frac{x^2}{4}\right)}^{-\frac{1}{2}}(-\frac{x}{2})\\ f\text{'}(x)=-\frac{x}{4\sqrt{25-\frac{x^2}{4}}}\end{array}$

To graph, plug in $Y_1=5+\sqrt{25-\frac{x^2}{4}},Y_2=-\frac{x}{4\sqrt{25-\frac{x^2}{4}}}\text{and}{Y}_3=-Y_2{(x-\frac{Y_1}{Y_2})}^2$ into graphing calculator.

  

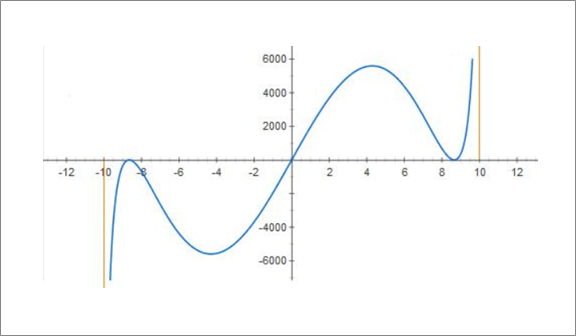
The domain of A(x) is the intersection of the domains of the f(x) and f’(x) for which $f\text{'}(x)\ne 0$ . So the domain of A is;

  $\text{Domain}\{x\in R:-10<x<10,x\ne 0\}$ .

There are vertical asymptotes x$=\pm 10$ at the edges of the domain of A(x).The definition of A(x) can be extended to include x = 0.

c.

To determine

To find: the height of the triangle with minimum area and how is it related to the y-coordinates of the center of the ellipse.

Answer to Problem 65E

Height of 15, its 3 times the y-coordinate of the center.

Explanation of Solution

Calculation:

Plugged in $Y_1=5+\sqrt{25-\frac{x^2}{4}}=f(x),Y_2=-\frac{x}{4\sqrt{25-\frac{x^2}{4}}}=f\text{'}(x)\text{and}{Y}_3=-Y_2{(x-\frac{Y_1}{Y_2})}^2=A(x)$ and then found the graph of A(x). Use the minimum feature of graphing calculator to find the minimum of A(x). This gives a minimum at the point (8.66. 259.808). The minimum area then occurs when x=8.66.

To find the height , use $h=a=x_{0}f\text{'}(x_0)=f(x)-xf\text{'}(x)$ found in part (a).

First find the values of f(x) and f’(x) at x =8.66 using calculator.

  $\begin{array}{c}h=f(x)-xf\text{'}(x)\\ =f(8.66)-8.66f\text{'}(8.66)\\ =7.50-8.66(-0.866)\\ =7.50+7.50\\ =15\end{array}$

The height of the triangle with minimum area is then 15. Since the y- coordinate of the center of the ellipse is 5, this is 3 times the y- coordinates.

Therefore, height of 15 , its 3 times the y-coordinate of the center.

d.

To determine

To repeat: parts (a) − (c ) for the ellipse

  $\frac{x^2}{C^2}+\frac{{(y-B)}^2}{B^2}=1$

Centered (0, B) and show that the triangle has minimum area when its height is 3B.

Answer to Problem 65E

Height of the triangle is 3b which is three times the y − coordinate of the center of the ellipse.

Explanation of Solution

Calculation:

  $\begin{array}{l}A(x)=-f\text{'}(x){\left(x-\frac{a}{f\text{'}(x)}\right)}^2\\ $ A\text{'}(x)={(x-\frac{f(x)}{f\text{'}(x)})}^2.(-f\text{'}\text{'}(x))+(-f\text{'}(x).2(x-\frac{f(x)}{f\text{'}(x)}).(1-\frac{{(f\text{'}(x))}^2-f(x).f\text{'}\text{'}(x))}{{(f\text{'}(x))}^2})$f(x)=\frac{b.\sqrt{c^2-x^2}+b.c}{c}\\ f\text{'}(x)=-\frac{b.x}{c.{(c^2-x^2)}^{\frac{3}{2}}}\\ f\text{'}\text{'}(x)=-\frac{b.c}{{(c^2-x^2)}^{\frac{3}{2}}}\\ A\text{'}(x)=0;x=-\frac{c.\sqrt{3}}{2},\frac{c.\sqrt{3}}{2}\\ y=f\text{'}(x).(-x)+f(x)\end{array}$

The height of the triangle can be found from the equation of the line tangent to point

P. y = height.

Therefore, height of the triangle is 3b which is three times the y − coordinate of the center of the ellipse.