Chapter 3.10, Problem 150P

A hollow cylindrical shaft of length L, mean radius c_m, and uniform thickness t is subjected to a torque of magnitude T. Consider, on the one hand, the values of the average shearing stress τ_ave and the angle of twist ϕ obtained from the elastic torsion formulas developed in Secs. 3.1C and 3.2 and, on the other hand, the corresponding values obtained from the formulas developed in Sec. 3.10 for thin-walled shafts, (a) Show that the relative error introduced by using the thin-walled-shaft formulas rather than the elastic torsion formulas is the same for τ_ave and ϕ and that the relative error is positive and proportional to the ratio t/c_m·(b) Compare the percent error corresponding to values of the ratio t/c_m of 0.1, 0.2, and 0.4.

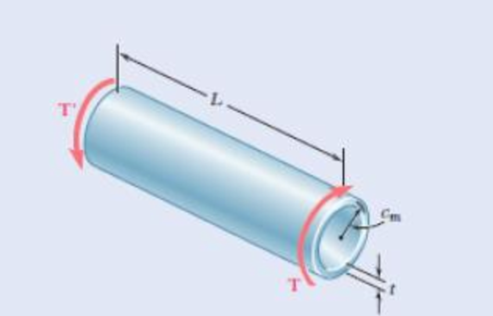
Fig. P3.150

To determine

Show that the relative error introduced by using the thin walled shaft formulas rather than the elastic torsion formulas is the same for ${\tau }_{ave}$ and $\varphi$ and that the relative error is positive and proportional to the ratio $\left(\raisebox{1ex}{$t$}\!\left/ \!\raisebox{-1ex}{$c_m$}\right.\right)$.

Answer to Problem 150P

The ratio $\left(\raisebox{1ex}{${\tau }_{ave}$}\!\left/ \!\raisebox{-1ex}{${\tau }_m$}\right.\right)$ is $\underset{\_}{1+\frac{1}{4}\frac{t^2}{c_m^2}}$.

The ratio $\left(\raisebox{1ex}{${\varphi }_2$}\!\left/ \!\raisebox{-1ex}{${\varphi }_1$}\right.\right)$ is $\underset{\_}{1+\frac{1}{4}\frac{t^2}{c_m^2}}$.

Explanation of Solution

Given information:

The hollow cylindrical shaft of length is (L).

The uniform thickness of the hollow cylinder is (t).

The mean radius of the hollow cylinder is $\left(c_m\right)$.

The magnitude of torque is (T).

Calculation:

Writ the equation for outer radius $\left(c_1\right)$:

$\left(c_1\right)=c_m+\frac{t}{2}$

Writ the equation for inner radius $\left(c_2\right)$:

$\left(c_2\right)=c_m-\frac{t}{2}$

Calculate the polar moment of inertia (J) using the relation:

$\begin{array}{c}J=\frac{\pi }{2}\left(c_2^4-c_1^4\right)\\ =\frac{\pi }{2}\left(c_2^2+c_1^2\right)\left(c_2+c_1\right)\left(c_2-c_1\right)\end{array}$

Substitute $c_m+\frac{t}{2}$ for $c_1$ and $c_m-\frac{t}{2}$ for $c_2$.

$\begin{array}{c}J=\frac{\pi }{2}\left({\left(c_m-\frac{t}{2}\right)}_{}^2+{\left(c_m+\frac{t}{2}\right)}_{}^2\right)\left(\left(c_m-\frac{t}{2}\right)+\left(c_m+\frac{t}{2}\right)\right)\left(\left(c_m-\frac{t}{2}\right)-\left(c_m+\frac{t}{2}\right)\right)\\ =\frac{\pi }{2}\left(c_m^2+c_m^{}t+\frac{1}{4}{t}^2+c_m^2-c_{m}t+\frac{1}{4}{t}^2\right)\left(2c_m\right)t\\ =2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)c_{m}t\end{array}$

Calculate the maximum shearing stress ${\tau }_m$ using the relation:

${\tau }_m=\frac{T{c}_m}{J}$

Substitute $2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)c_{m}t$ for J.

$\begin{array}{c}{\tau }_m=\frac{T{c}_m}{2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)c_{m}t}\\ =\frac{T}{2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)t}\end{array}$

Calculate the angle twist $\left({\varphi }_1\right)$ using the relation:

${\varphi }_1=\frac{TL}{JG}$

Here, G is rigidity modulus.

Substitute $2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)c_{m}t$ for J.

${\varphi }_1=\frac{TL}{2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)c_{m}tG}$

Write the expression to calculate the area bounded by centerline $\left(a\right)$.

$a=\pi c_m{}^2$

Calculate the shearing stress at tube ‘a’ $\left({\tau }_{ave}\right)$ using the relation:

${\tau }_{ave}=\frac{T}{2ta}$

Substitute $\pi c_m{}^2$ for $a$.

$\begin{array}{c}{\tau }_{ave}=\frac{T}{2\times t\times \pi c_m{}^2}\\ =\frac{T}{2\pi t{c}_m{}^2}\end{array}$

Calculate the angle twist $\left({\varphi }_2\right)$ using the elastic torsion formula:

${\varphi }_2=\frac{TL}{4a^{2}G}{\displaystyle \oint \frac{ds}{t}}$

Substitute $\pi c_m{}^2$ for a.

$\begin{array}{c}{\varphi }_2=\frac{TL}{4{\left(\pi c_m{}^2\right)}^{2}G}{\displaystyle \oint \frac{ds}{t}}\\ =\frac{TL}{4{\left(\pi c_m{}^2\right)}^{2}G}\left(\frac{2\pi c_m}{t}\right)\\ =\frac{TL}{2\pi c_m^{3}tG}\end{array}$

Calculate the ratio $\left(\frac{{\tau }_{ave}}{{\tau }_m}\right)$:

Substitute $\frac{T}{2\pi t{c}_m{}^2}$ for ${\tau }_{ave}$ and $\frac{T}{2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)t}$ for ${\tau }_m$.

$\begin{array}{c}\left(\frac{{\tau }_{ave}}{{\tau }_m}\right)=\frac{\left(\frac{T}{2\pi t{c}_m{}^2}\right)}{\left(\frac{T}{2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)t}\right)}\\ =\frac{T}{2\pi t{c}_m{}^2}\times \frac{2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)t}{T}\\ =1+\frac{1}{4}\frac{t^2}{c_m^2}\end{array}$

Calculate the ratio $\left(\frac{{\varphi }_2}{{\varphi }_1}\right)$:

Substitute $\frac{TL}{2\pi c_m^{3}tG}$ for ${\varphi }_2$ and $\frac{TL}{2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)c_{m}tG}$ for ${\varphi }_1$.

$\begin{array}{c}\left(\frac{{\varphi }_2}{{\varphi }_1}\right)=\frac{\left(\frac{TL}{2\pi c_m^{3}tG}\right)}{\left(\frac{TL}{2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)c_{m}tG}\right)}\\ =\frac{TL}{2\pi c_m^{3}tG}\times \frac{2\pi \left(c_m^2+\frac{1}{4}{t}^2\right)c_{m}tG}{TL}\\ =1+\frac{1}{4}\frac{t^2}{c_m}\end{array}$

Thus, the ratio $\left(\raisebox{1ex}{${\tau }_{ave}$}\!\left/ \!\raisebox{-1ex}{${\tau }_m$}\right.\right)$ is $\underset{\_}{1+\frac{1}{4}\frac{t^2}{c_m^2}}$.

Thus, the ratio $\left(\raisebox{1ex}{${\varphi }_2$}\!\left/ \!\raisebox{-1ex}{${\varphi }_1$}\right.\right)$ is $\underset{\_}{1+\frac{1}{4}\frac{t^2}{c_m^2}}$.

To determine

Compare the percent error corresponding to value of the ratio $\left(\raisebox{1ex}{$t$}\!\left/ \!\raisebox{-1ex}{$c_m$}\right.\right)$ of 0.1,0.2 and 0.4.

Answer to Problem 150P

The ratio $\left(\raisebox{1ex}{$t$}\!\left/ \!\raisebox{-1ex}{$c_m$}\right.\right)$ of 0.1, 0.2 and 0.4 are $\underset{\_}{0.25\%,1.0\%}$, and $\underset{\_}{4.0\%}$ respectively.

Explanation of Solution

Calculation:

Calculate the percent error corresponding to value of the ratio $\left(\raisebox{1ex}{$t$}\!\left/ \!\raisebox{-1ex}{$c_m$}\right.\right)$ of 0.1,0.2 and 0.4:

$\left(\frac{{\tau }_{ave}}{{\tau }_m}\right)=\left(\frac{{\varphi }_2}{{\varphi }_1}\right)$

Substitute $1+\frac{1}{4}\frac{t^2}{c_m^2}$ for $\frac{{\tau }_{ave}}{{\tau }_m}$ and $1+\frac{1}{4}\frac{t^2}{c_m^2}$ for $\frac{{\varphi }_2}{{\varphi }_1}$.

$\begin{array}{l}\left(\frac{{\tau }_{ave}}{{\tau }_m}-1\right)=\left(\frac{{\varphi }_2}{{\varphi }_1}-1\right)=\frac{1}{4}\frac{t^2}{c_m^2}\\ \end{array}$ (1).

Calculate the ratio for value 0.1 using the Equation (1).

Substitute 0.1 for $\left(\raisebox{1ex}{$t$}\!\left/ \!\raisebox{-1ex}{$c_m$}\right.\right)$.

$\begin{array}{c}\frac{1}{4}\frac{t^2}{c_m^2}=\frac{1}{4}{\left(0.1\right)}^2\\ =0.0025\\ =0.0025\times 100\%\\ =0.25\%\end{array}$

Calculate the ratio for value 0.2 using the Equation (1).

Substitute 0.2 for $\left(\raisebox{1ex}{$t$}\!\left/ \!\raisebox{-1ex}{$c_m$}\right.\right)$.

$\begin{array}{c}\frac{1}{4}\frac{t^2}{c_m^2}=\frac{1}{4}{\left(0.2\right)}^2\\ =0.01\\ =0.01\times 100\%\\ =1.0\%\end{array}$

Calculate the ratio for value 0.3 using the Equation (1).

Substitute 0.3 for $\left(\raisebox{1ex}{$t$}\!\left/ \!\raisebox{-1ex}{$c_m$}\right.\right)$.

$\begin{array}{c}\frac{1}{4}\frac{t^2}{c_m^2}=\frac{1}{4}{\left(0.3\right)}^2\\ =0.04\\ =0.04\times 100\%\\ =4.0\%\end{array}$

Thus, the ratio $\left(\raisebox{1ex}{$t$}\!\left/ \!\raisebox{-1ex}{$c_m$}\right.\right)$ of 0.1, 0.2 and 0.4 are $\underset{\_}{0.25\%,1.0\%}$, and $\underset{\_}{4.0\%}$ respectively.