Chapter 20, Problem 42P

(a)

To determine

The magnitude and direction of the current in the lower wire.

Answer to Problem 42P

The current in the lower wire is $6700\text{ A}$ in right direction.

Explanation of Solution

Given:

The given figure is shown below.

  

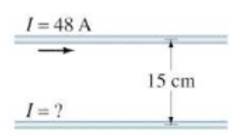
Current carried by upper horizontal wire is $48\text{ A}$ and another wire 15 cm below has a diameter of 2.5 mm.

Formula used:

The magnetic force is given by the formula

  ${\text{F}}_2=\frac{{\mu }_0}{2\pi }\frac{{\text{I}}_1{\text{I}}_2}{{\text{d}}_2}{\text{l}}_2$

Calculation:

Using the right-hand rule, the magnetic force exerted on the lower wire is in an upwards direction. Now, since every force must be balanced. Thus, the magnetic force exerted upwards must be balanced by the gravitational force experienced downwards. Considering the length of the lower copper wire as $\text{L}$

  $\begin{array}{l}{\text{F}}_{\text{g}}={\text{F}}_{\text{B}}\\ {\text{m}}_{\text{Cu}}\text{g}=\frac{{\mu }_0}{2\pi }\frac{{\text{I}}_{\text{top}}{\text{I}}_{\text{Cu}}}{\text{d}}{\text{L}}_{\text{Cu}}\\ {\text{I}}_{\text{Cu}}=\frac{2\pi {\text{dm}}_{\text{Cu}}\text{g}}{{\mu }_0{\text{I}}_{\text{top}}{\text{L}}_{\text{Cu}}}=\frac{2\pi \text{d}{\rho }_{\text{Cu}}{\text{L}}_{\text{Cu}}\pi {\text{r}}^2\text{g}}{{\mu }_0{\text{I}}_{\text{top}}{\text{L}}_{\text{Cu}}}\\ {\text{I}}_{\text{Cu}}=\frac{2\pi (0.15\text{ m})(8900{\text{ kg/m}}^3)\pi {(1.25\times {10}^{-3}\text{ m})}^2(9.8{\text{ m/s}}^2)}{(4\pi \times {10}^{-7}\text{ T}·\text{m/A})(48\text{ A})}\\ {\text{I}}_{\text{Cu}}=6700\text{ A}\end{array}$

Conclusion:

Thus, for the magnetic force to be exerted upwards, the current must be in the right direction and the magnitude of the current in the lower wire is $6700\text{ A}$ .

(b)

To determine

To identify: Whether the lower wire in stable equilibrium.

Answer to Problem 42P

The lower wire is not in a stable equilibrium.

Explanation of Solution

Given:

The given figure is shown below.

  

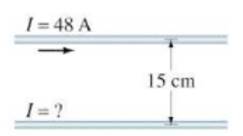
Current carried by upper horizontal wire is $48\text{ A}$ and another wire 15 cm below has a diameter of 2.5 mm.

Formula used:

The magnetic force is given by the formula

  ${\text{F}}_2=\frac{{\mu }_0}{2\pi }\frac{{\text{I}}_1{\text{I}}_2}{{\text{d}}_2}{\text{l}}_2$

Calculation:

To maintain stable equilibrium, all the forces exerted on any object must be balanced by the counter forces exerted by it.

Here, the magnetic force exerted on the copper wire is balanced by the gravitational force downwards. If wire moves a little bit farther from the top wire then the magnetic force weakens and the gravitational force supersedes. Thus, it will keep moving downwards, and farther it goes away from the top wire. Hence, the copper wire will not be in stable equilibrium.

Conclusion:

The lower Copper wire is not in a stable equilibrium.

(c)

To determine

The magnitude and direction of the current in the upper wire.

To identify: Whether the upper wire is in stable equilibrium or not.

Answer to Problem 42P

The current in the copper wire is $6700\text{ A}$ in the left direction. The upper wire is in stable equilibrium.

Explanation of Solution

Given:

Current carried by horizontal wire is $48\text{ A}$ and another wire 15 cm above has a diameter of 2.5 mm.

Formula used:

The magnetic force is given by the formula

  ${\text{F}}_2=\frac{{\mu }_0}{2\pi }\frac{{\text{I}}_1{\text{I}}_2}{{\text{d}}_2}{\text{l}}_2$

Calculation:

Even if the wire is displaced in the opposite direction i.e. in the upward direction. The magnetic force exerted on it will be upwards and with the same magnitude of $6700\text{ A}$ , using the same calculation above. But, this time current will be in the opposite direction i.e. in the left direction.

To maintain stable equilibrium, all the forces exerted on any object must be balanced by the counter forces exerted by it. Here, the magnetic force exerted on the Copper wire is balanced by the gravitational force downwards. If wire moves a little bit upwards then the magnetic force decreases and the gravitational force supersedes and it will bring the wire in the original position.

If distance between both the wires decreases then magnetic force on the Copper wire increases and it will tend to move it back to its original position.

Thus, no matter, how the distance varies between both the wires. Copper wire will remain in stable equilibrium.

Conclusion:

Thus, for the magnetic force to be exerted upwards, the current must be in the left direction and the magnitude of the current in the upper wire is $6700\text{ A}$ . The upper Copper wire remains in stable equilibrium.