Chapter 14.1, Problem 37E

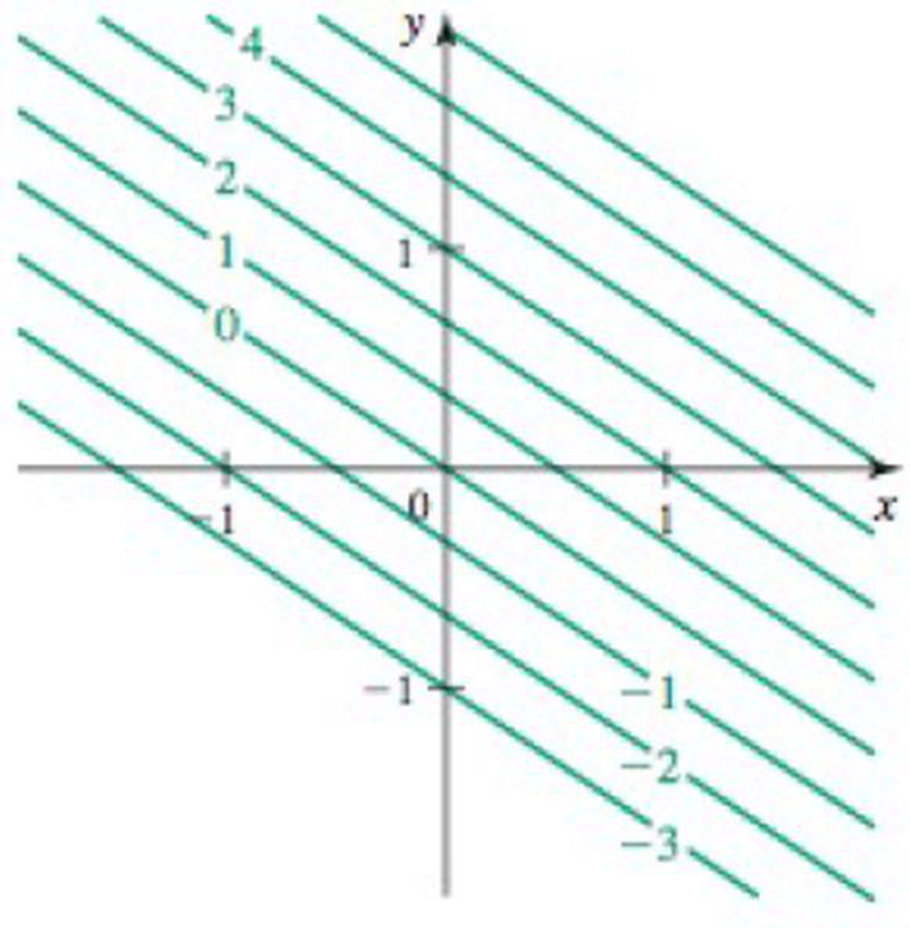
Equipotential curves Consider the following potential functions and graphs of their equipotential curves.

a.    Find the associated gradient field F = ▿ϕ.

b.    Show that the vector field is orthogonal to the equipotential curve at the point (1, 1). Illustrate this result on the figure.

c.    Show that the vector field is orthogonal to the equipotential curve at all points (x, y).

d.    Sketch two flow curves representing F that are everywhere orthogonal to the equipotential curves.

37.    ϕ (x, y) = 2x +3y