Chapter 11.2, Problem 91AYU

Make up a system of three linear equations containing three variables that has:

a. No solution.

b. Exactly one solution.

c. Infinitely many solutions.

Give the three systems to a friend to solve and critique.

To determine

To find: Give the three systems to a friend to solve and critique.

Answer to Problem 91AYU

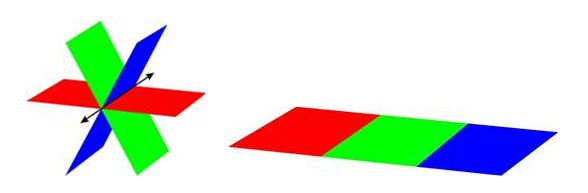
Case 1: There is one solution. In order for three equations with three variables to have one solution, the planes must intersect in a single point.

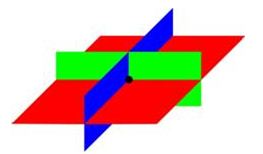
Case 2: There is no solution. The three planes do not have any points in common. (Note that two of the equations may have points in common with each other, but not all three). Below are examples of some of the ways this can happen.

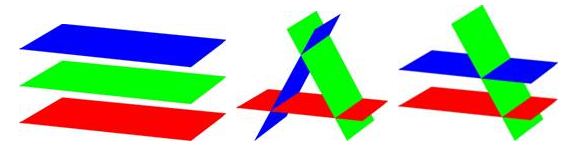
Case 3: There are an infinite number of solutions. This occurs when the three planes intersect in a line. And this can also occur when the three equations graph as the same plane.

Let’s start by looking at Case 1, where the system has a unique (one) solution. This is the case that you are usually most interested in.

Here is a system of linear equations. There are three variables and three equations.

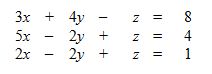
You know how to solve a system with two equations and two variables. For the first step, use the elimination method to remove one of the variables. In this case, $z$ can be eliminated by adding the first and second equations.

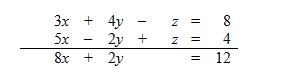
To solve the system, though, you need two equations using two variables. Adding the first and third equations in the original system will also give an equation with $x$ and $y$ but not $z$.

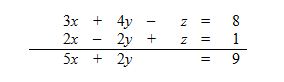
Now you have a system of two equations and two variables.

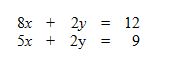
Solve the system using elimination again. In this case, you can eliminate $y$ by adding the opposite of the second equation:

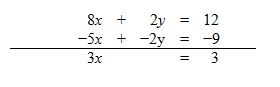
Solve the resulting equation for the remaining variable.

$\begin{array}{r}\hfill 3x =3\\ \hfill x =1\end{array}$

Now you use one of the equations in the two-variable system to find $y$.

$\begin{array}{r}\hfill 5x +2y =9\\ \hfill 5\left(1\right)+2y =9\\ \hfill 5+2y =9\\ \hfill 2y =4\\ \hfill y =2\end{array}$

Finally, use any equation from the first system, along with the values already found, to solve for the last variable.

$\begin{array}{r}\hfill 2x –2y + z =1\\ \hfill 2\left(1\right)–2\left(2\right)+ z =1\\ \hfill 2–4+ z =1\\ \hfill -2+ z =1\\ \hfill z =3\end{array}$

Explanation of Solution

Given:

Make up a system of three linear equations containing three variables.

a. No solution.

b. Exactly one solution.

c. Infinitely many solutions.

Calculation:

Just as when solving a system of two equations, there are three possible outcomes for the solution of a system of three variables. Let’s look at this visually, although you will not be graphing these equations.

Case 1: There is one solution. In order for three equations with three variables to have one solution, the planes must intersect in a single point.

Case 2: There is no solution. The three planes do not have any points in common. (Note that two of the equations may have points in common with each other, but not all three). Below are examples of some of the ways this can happen.

Case 3: There are an infinite number of solutions. This occurs when the three planes intersect in a line. And this can also occur when the three equations graph as the same plane.

Let’s start by looking at Case 1, where the system has a unique (one) solution. This is the case that you are usually most interested in.

Here is a system of linear equations. There are three variables and three equations.

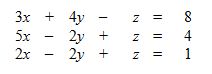
You know how to solve a system with two equations and two variables. For the first step, use the elimination method to remove one of the variables. In this case, $z$ can be eliminated by adding the first and second equations.

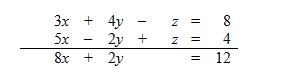
To solve the system, though, you need two equations using two variables. Adding the first and third equations in the original system will also give an equation with $x$ and $y$ but not $z$.

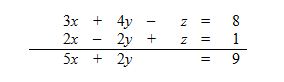
Now you have a system of two equations and two variables.

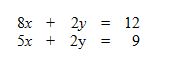
Solve the system using elimination again. In this case, you can eliminate $y$ by adding the opposite of the second equation:

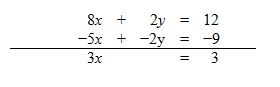
Solve the resulting equation for the remaining variable.

$\begin{array}{r}\hfill 3x =3\\ \hfill x =1\end{array}$

Now you use one of the equations in the two-variable system to find $y$.

$\begin{array}{r}\hfill 5x +2y =9\\ \hfill 5\left(1\right)+2y =9\\ \hfill 5+2y =9\\ \hfill 2y =4\\ \hfill y =2\end{array}$

Finally, use any equation from the first system, along with the values already found, to solve for the last variable.

$\begin{array}{r}\hfill 2x –2y + z =1\\ \hfill 2\left(1\right)–2\left(2\right)+ z =1\\ \hfill 2–4+ z =1\\ \hfill -2+ z =1\\ \hfill z =3\end{array}$