Chapter 1.3, Problem 76E

To determine

To find: the function is even or odd or neither using graph.

Answer to Problem 76E

Neither even nor odd

Explanation of Solution

Given information:

Given Function is $g(t)=\sqrt[3]{(t-1)}$

Concept Used:-

Odd function: - A function f(x) is said to be even if graph of function is symmetric about origin.

For example: -

  

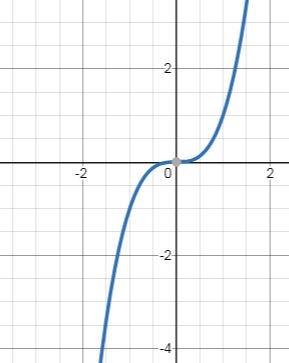
Even function: - A function f(x) is said to be even if graph of function is symmetric about y-axis.

For example:-

  

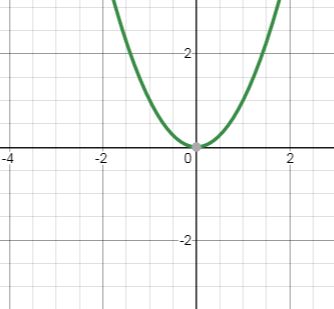
Calculation:-

Drawing graph of function

  

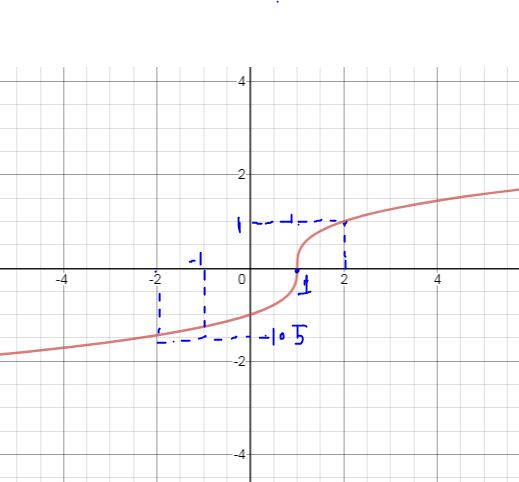
Symmetricity about Y-axis: - If graph is symmetric about y-axis if (x, y) is on graph then (-x, y) is also on graph for all x in domain.

Since, (2, 1) is on graph but (-2, 1) (as at x = -2, g (t) = -1.5) is not on graph.

So, using graph we can see (x, y) is on graph but (-x, y) is not on graph.

And function is not symmetric about y-axis.

Symmetricity about origin: - If graph is symmetric about origin if (x, y) is on graph then (-x, -y) is also on graph for all x in domain.

But using graph we can see (1, 0) is on graph but (-1, 0) is not on graph.

That is, if (x, y) is on graph then (-x, -y) is not on graph for all values of x in domain.

So, function is not symmetric about origin.

Therefore, the given function is neither symmetric about y-axis nor about origin. So, it is neither even nor odd.