Chapter 1.2, Problem 78E

To determine

To obtain: the area of the rectangle as a function of the given variable.

Also to find its domain.

Answer to Problem 78E

The area of the rectangle is given by $A=2x\sqrt{36-x^2}$.

The domain of the function is $0<x<6$.

Explanation of Solution

Given Information:

A rectangle is bounded by the x -axis and the semicircle with equation $y=\sqrt{36-x^2}$ .

Formula Used (1)The area of a rectangle with length l and width w is given by,

  $A=lw$

(2) The domain of any function f (x ) is all real values of x for which the function can be defined.

Calculation

Here, the semicircle and the rectangle intersect at the point (x, y ) as shown in figure below.

  

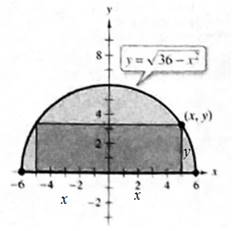
From the figure it can be seen that the length of the rectangle is 2x and the width of the rectangle is given by y . Therefore, the area of the rectangle can be expressed as,

  $A=2x\times y$

Now, considering the equation of the semicircle, $y=\sqrt{36-x^2}$ , the area of the rectangle can be rewritten fully in terms of x as,

  $\begin{array}{c}A=2x\times \sqrt{36-x^2}\\ =2x\sqrt{36-x^2}\end{array}$

The domain of the function representing the area of the rectangle $A=2x\sqrt{36-x^2}$ is given by all those real values of x which makes the function definable. The function is area here which places a restriction on the values of x to be real. Here, the value of x inside the square root should be less than or equal to 6 to ensure that the function has a real value. For x =0 and x =6 the rectangle ceases to exist. So it is not considered. Thus, the domain of the function representing the area A is given by $0<x<6$ .