9th Edition

ISBN: 9780321716835

Author: Michael Sullivan

Publisher: Addison Wesley

Concept explainers

In mathematics, traditional optimization problems are typically expressed in terms of minimization. When we talk about minimizing or maximizing a function, we refer to the maximum and minimum possible values of that function. This can be expressed in ter…

Maxima and Minima

The extreme points of a function are the maximum and the minimum points of the function. A maximum is attained when the function takes the maximum value and a minimum is attained when the function takes the minimum value.

Derivatives

A derivative means a change. Geometrically it can be represented as a line with some steepness. Imagine climbing a mountain which is very steep and 500 meters high. Is it easier to climb? Definitely not! Suppose walking on the road for 500 meters. Which …

Concavity

In calculus, concavity is a descriptor of mathematics that tells about the shape of the graph. It is the parameter that helps to estimate the maximum and minimum value of any of the functions and the concave nature using the graphical method. We use the …

Videos

Textbook Question

Chapter 7, Problem 11CR

Consider the function

$f ( x ) = 2 x 5 − x 4 − 4 x 3 + 2 x 2 + 2 x − 1$

Find the real zeros and their multiplicity.

Find the intercepts.

Find the power function that the graph of $f$ resembles for large $| x | .$

Graph $f$ using a graphing utility.

Approximate the turning points, if any exist.

Use the information obtained in parts $( a ) − ( e )$ to graph $f$ by hand.

Identify the intervals on which $f$ is increasing, decreasing, or constant.

(a)

Expert Solution

To determine

The real zeros of $f(x)=2x5−x4−4x3+2x2+2x−1$ and their multiplicity.

Answer to Problem 11CR

Solution:

The real zeros of $f(x)=2x5−x4−4x3+2x2+2x−1$ are $−1,12,1$ .

$−1$ and $1$ are the zero of the function with multiplicity $2$ whereas $12$ is the zero of the function with multiplicity $1$ .

Explanation of Solution

Given information:

The function, $f(x)=2x5−x4−4x3+2x2+2x−1$

Explanation:

Consider the function $f(x)=2x5−x4−4x3+2x2+2x−1$ .

By rational root theorem,

The divisors of the constant term are $p=±1$ .

The divisors of the leading coefficient are $q=±1, ±2$ .

Then, possible rational zeros of the polynomial are, $pq=±1, ±12$ .

Now, test $x=−1$ using synthetic division.

$−1 2 −1 −4 2 2 −1 −2 3 1 −3 1_ 2 −3 −1 3 −1 0$

Here, since the remainder is $0$ , $x=−1$ is a zero of $f$ .

After taking $x+1$ as a factor,

$2x5−x4−4x3+2x2+2x−1=(x+1)(2x4−3x3−x2+3x−1)$

Then, the depressed equation is $2x4−3x3−x2+3x−1$ .

$2x4−3x3−x2+3x−1$

$=x4+x4−3x3−x2+3x−1$

$=x4−x2+x4−1−3x3+3x$

$=x2(x2−1)+(x2−1)(x2+1)−3x(x2−1)$

$=(x2−1)(x2+x2+1−3x)$

$=(x2−1)(2x2+1−3x)$

$=(x+1)(x−1)(2x2+1−3x)$ .

By quadratic formula, the zeros of the quadratic equation $2x2+1−3x$ ; $x=−b±b2−4ac2a$ .

$x=−(−3)±9−84=3±14=$

$⇒x=1 or x=12$

The factors of $2x2+1−3x$ are $(x−1) and (x−12)$ .

$2x5−x4−4x3+2x2+2x−1=(x+1)(x+1)(x−1)(2x2+1−3x)=(x+1)(x+1)(x−1)(x−1)(x−12)$

The factor form of $f(x)$ is.

$2x5−x4−4x3+2x2+2x−1=(x+1)2(x−1)2(x−12)$

Hence, the real zeros of $f(x)=2x5−x4−4x3+2x2+2x−1$ are $−1,12,1$ and the factor form is $(x+1)2(x−1)2(x−12)$ .

$−1$ is the zero of the function with multiplicity $2$ since the exponent of the factor $(x+1)2$ is $2$ .

$12$ is the zero of the function with multiplicity $1$ since the exponent of the factor $x−12$

is $1$ .

$1$ is the zero of the function with multiplicity $2$ since the exponent of the factor $(x−1)2$ is $2$ .

(b)

Expert Solution

To determine

The $x−$ intercept and $y$ -intercepts of the function, $f(x)=2x5−x4−4x3+2x2+2x−1$ .

Answer to Problem 11CR

Solution:

The $x−$ intercepts of the function are $(0, −1),( 0, 12)$ and, $(0, 1)$ and the $y−$ intercept of the polynomial function is $(−1, 0)$ .

Explanation of Solution

Given information:

The function $f(x)=2x5−x4−4x3+2x2+2x−1$ .

Explanation:

To find $y−$ intercepts substitute $x=0$ in the function $f(x)=2x5−x4−4x3+2x2+2x−1$ ,

$f(0)=2(0)5−(0)4−4(0)3+2(0)2+2(0)−1=−1$

Thus the $y−$ intercept of the polynomial function is $(−1, 0)$ .

Now to find $x−$ intercept of the function substitute $f(x)=0$ in the function.

$f(x)=2x5−x4−4x3+2x2+2x−1$ , it gives

$0=2x5−x4−4x3+2x2+2x−1$

$⇒(x+1)2(x−1)2(x−12)=0$

$⇒ x+1=0$ or, $x−1=0$ or $x−12=0$

$⇒x=−1$ or, $x=1$ or $x=12$

Hence the $x−$ intercepts of the function are $(0, −1),( 0, 12)$ and $(0, 1)$ .

(c)

Expert Solution

To determine

The power function that the graph of $f(x)=2x5−x4−4x3+2x2+2x−1$ resembles for large values of $|x|$ .

Answer to Problem 11CR

Solution:

Thegraph of the function $f(x)=2x5−x4−4x3+2x2+2x−1$ resembles like $y=2x5$ for large values of $|x|$ .

Explanation of Solution

Given information:

The function $f(x)=2x5−x4−4x3+2x2+2x−1$ .

The polynomial function is $f(x)=2x5−x4−4x3+2x2+2x−1$ .

Here the degree of the polynomial function $f(x)$ is $5$ .

The graph of the function $f(x)=2x5−x4−4x3+2x2+2x−1$ behaves like $y=2x5$ for large values of $|x|$ .

(d)

Expert Solution

To determine

To graph: The function $f(x)=2x5−x4−4x3+2x2+2x−1$ using a graphing utility.

Explanation of Solution

Given information:

The function $f(x)=2x5−x4−4x3+2x2+2x−1$ .

Graph:

Use the steps below to graph the function using a graphing calculator.

Step I: Press the ON key.

Step II: Now, press [Y=]. Input the right hand side of the function $y=2x5−x4−4x3+2x2+2x−1$ in $Y1$

Step III: Press [WINDOW] key and set the viewing window as below,

$Xmin= −2Xmax= 2 X scl= 1 Ymin= −2 Ymax= 1 Y scl= 1$ .

Step IV: Then hit [Graph] key to view the graph.

The graph of the function is as follows:

Precalculus, Chapter 7, Problem 11CR , additional homework tip 1

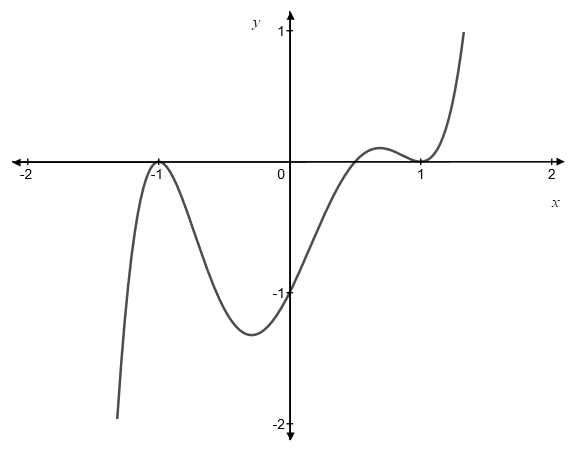
Interpretation:

The graph of the function $f(x)$ crosses the $x−$ axis at $x=12$ since the multiplicity of the $12$ is $1$ that is odd multiplicity.

(e)

Expert Solution

To determine

The approximation of the turning points, if exists, of the function $f(x)=2x5−x4−4x3+2x2+2x−1$ .

Answer to Problem 11CR

Solution:

The turning points of $f(x)=2x5−x4−4x3+2x2+2x−1$ are $(−0.29, −1.325),(1,0)$ , $(0.69, 0.104),(−1,0)$ .

Explanation of Solution

Given information:

The function $f(x)=2x5−x4−4x3+2x2+2x−1$ .

Explanation:

Let, the function $f(x)=2x5−x4−4x3+2x2+2x−1$ .

The maximum number of real zeros is the degree of the polynomial.

Here, the degree of $f(x)=2x5−x4−4x3+2x2+2x−1$ is $5$ .

Since the polynomial function $f(x)=2x5−x4−4x3+2x2+2x−1$ has degree $5$ so the maximum number of the turning points on the graph of the function $f(x)$ is $5−1=4$ .

For the approximation of the turning points find out the maxima and minima using a graphing calculator.

To graph the function $f(x)=2x5−x4−4x3+2x2+2x−1$ using graphing utility use the below steps.

Step I: Press the ON key.

Step II: Now, press [Y=]. Input the right hand side of the function $y=2x5−x4−4x3+2x2+2x−1$ in $Y1$

Step III: Press [WINDOW] key and set the viewing window as below,

$Xmin= −2Xmax= 2 X scl= 1 Ymin= −2 Ymax= 1 Y scl= 1$ .

Step IV: Then hit [Graph] key to view the graph.

The graph of the function is as follows:

Precalculus, Chapter 7, Problem 11CR , additional homework tip 2

To find local maximum and local minimum on the graph using graphing utility use below steps,

Step IV: Press [2ND] [TRACE] to access the calculate menu

Step V: press [MAXIMUM] and press [ENTER].

Step VI: Set left bound by using the left and right arrow. Click [ENTER].

Step VII: Set right bound by using the left and right arrow. Click [ENTER].

Step VIII: Click [Enter] button twice.

It will give the maximum value $x=0.69,y=0.104$

It will give the maximum value $x=−1,y=0$

Thus, the function have its local maximum value at $(0.69, 0.104),(−1,0)$ .

To find local minimum value use below steps.

Step IX: Press [2ND] [TRACE] to access the calculate menu

Step X: press [MINIMUM] and press [ENTER].

Step XI: Set left bound by using the left and right arrow. Click [ENTER].

Step XII: Set right bound by using the left and right arrow. Click [ENTER].

Step XIII: Click [Enter] button twice.

It will give the minimum value $x=−0.29,y=−1.325$ .

It will give the minimum value $x=1,y=0$ .

Thus, the function has its local minimum value at $(−0.29, −1.325),(1,0)$ .

Therefore, the turning points are $(−0.29, −1.325),(1,0)$ , $(0.69, 0.104),(−1,0)$ .

(f)

Expert Solution

To determine

To graph: The function $f(x)=2x5−x4−4x3+2x2+2x−1$ .

Explanation of Solution

Given information:

The function $f(x)=2x5−x4−4x3+2x2+2x−1$

Graph:

The polynomial function is $f(x)=2x5−x4−4x3+2x2+2x−1$ .

From all the above parts, the analysis of the function $f(x)=2x5−x4−4x3+2x2+2x−1$ are stated below:

The graph of the function $f(x)=2x5−x4−4x3+2x2+2x−1$ behaves like $y=2x5$ for large values of $|x|$ .

Thezeros of the function are $−5,−12$ and $3$

The $x−$ intercepts of the function are $−1,12$ and $1$ and the $y−$ intercept of the polynomial function is $−1$ .

The graph of the function $f(x)$ crosses the $x−$ axis at $x=12$ since the multiplicity of the $12$ is $1$ that is odd multiplicity and also the graph of function $f(x)$ touches the $x−$ axis at $x=1,x=−1$ since the multiplicity of the $−1, 1$ are $2$ which is even.

Here the degree of the polynomial function $f(x)$ is $5$ , the maximum number of tuning points are $5−1=4$ which are at $x=−1,x=0.29,x=0.69,x=1$ .

Using all this information, the graph will look alike:

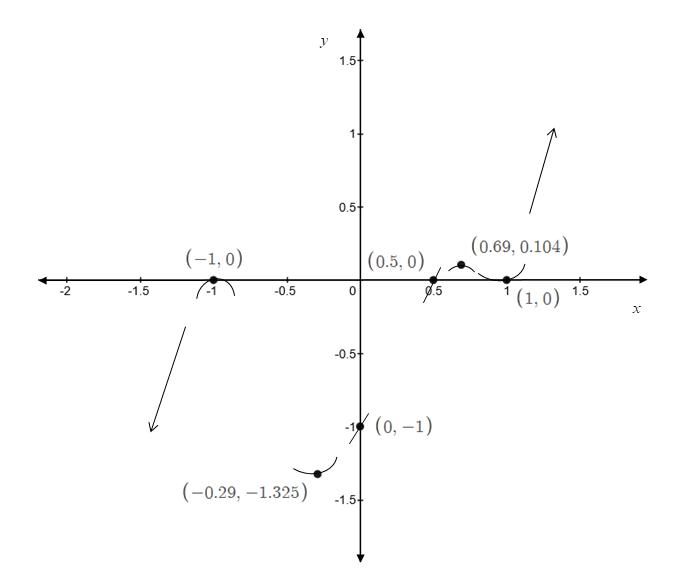
Precalculus, Chapter 7, Problem 11CR , additional homework tip 3

Now find additional points on the graph on each side of $x−$ intercept as follows

For $x=−1.1$ the value of $f(x)$ at $x=−1.1$ is $f(−1.1)=2(−1.1)5−(−1.1)4−4(−1.1)3+2(−1.1)2+2(−1.1)−1=−0.1411$

For $x=−0.5$ the value of $f(x)$ at $x=−0.5$ is $f(−0.5)=2(−0.5)5−(−0.5)4−4(−0.5)3+2(−0.5)2+2(−0.5)−1=−1.125$

For $x=−0.25$ the value of $f(x)$ at $x=−0.25$ is $f(−0.25)=2(−0.25)5−(−0.25)4−4(−0.25)3+2(−0.25)2+2(−0.25)−1=−1.3183$

For $x=0.25$ the value of $f(x)$ at $x=0.25$ is $f(0.25)=2(0.25)5−(0.25)4−4(0.25)3+2(0.25)2+2(0.25)−1=−0.4395$

For $x=0.55$ the value of $f(x)$ at $x=0.55$ is $f(0.55)=2(0.55)5−(0.55)4−4(0.55)3+2(0.55)2+2(0.55)−1=0.0487$

For $x=0.75$ the value of $f(x)$ at $x=0.75$ is $f(0.25)=2(0.25)5−(0.75)4−4(0.75)3+2(0.75)2+2(0.75)−1=0.0957$

For $x=1.1$ the value of $f(x)$ at $x=1.1$ is $f(1.1)=2(1.1)5−(1.1)4−4(1.1)3+2(1.1)2+2(1.1)−1=0.0529$

Now plot all these coordinates $(−1.1, −0.1411), (−0.5, 1.125), (−0.25, −1.3183), (0.25, −0.4395),(0.55,0.0487),(0.75,0.0957),(1.1,0.0529)$ on the graph and join them.

Therefore, the graph of the function is as follows:

Precalculus, Chapter 7, Problem 11CR , additional homework tip 4

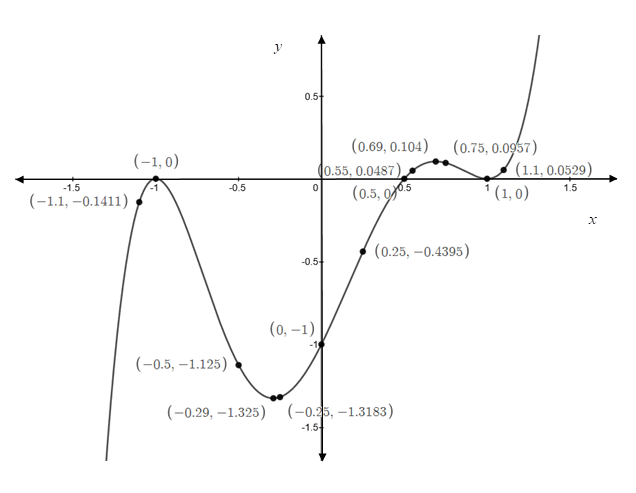
Interpretation:

The graph of the function $f(x)=2x5−x4−4x3+2x2+2x−1$ behaves like $y=2x5$ for large values of $|x|$ .

Thezeros of the function are $−5,−12$ and $3$ .

The $x−$ intercepts of the function are $−1,12$ and, $1$ and the $y−$ intercept of the polynomial function is $−1$ .

Here the degree of the polynomial function $f(x)$ is $5$ , the maximum number of tuning points are $5−1=4$ which are at $x=−1,x=0.29,x=0.69,x=1$ .

(g)

Expert Solution

To determine

The intervals where the function $f(x)=2x5−x4−4x3+2x2+2x−1$ is increasing, or decreasing or constant.

Answer to Problem 11CR

Solution:

The function $f(x)=2x5−x4−4x3+2x2+2x−1$ is increasing in the interval $(−∞,−1),(−0.29,0.69),(1,∞)$ and decreasing in the intervals $(−1,−0.29),(0.69,1)$ and nowhere constant.

Explanation of Solution

Given information:

The function, $f(x)=2x5−x4−4x3+2x2+2x−1$ .

The polynomial function is $f(x)=2x5−x4−4x3+2x2+2x−1$ .

From parts (d),(e),(f) the graph of the function $f(x)=2x5−x4−4x3+2x2+2x−1$ is stated below:

Precalculus, Chapter 7, Problem 11CR , additional homework tip 5

Here the degree of the polynomial function $f(x)$ is $5$ , the maximum number of tuning points are $5−1=4$ which are at $x=−1,x=0.29,x=0.69,x=1$ .

From the graph, it is clearly evident the graph is increasing in the interval $(−∞,−1),(−0.29,0.69),(1,∞)$ and decreasing in the intervals $(−1,−0.29),(0.69,1)$ and nowhere constant.

Chapter 7, Problem 10CR

Chapter 7, Problem 12CR

Chapter 7 Solutions

Precalculus

Ch. 7.1 - Prob. 1AYU Ch. 7.1 - Prob. 2AYU Ch. 7.1 - Prob. 3AYU Ch. 7.1 - Prob. 4AYU Ch. 7.1 - Prob. 5AYU Ch. 7.1 - Prob. 6AYU Ch. 7.1 - y= sin 1 x means _____, where 1x1 and 2 y 2 .Ch. 7.1 - Prob. 8AYU Ch. 7.1 - Prob. 9AYU Ch. 7.1 - Prob. 10AYU

Additional Math Textbook Solutions

Find more solutions based on key concepts

Shell method Let R be the region bounded by the following curves. Use the shell method to find the volume of th...

Calculus: Early Transcendentals (2nd Edition)

The sequence 2,3,4,5,….. in symbols and words

Pre-Algebra Student Edition

In Exercises 11-20, express each decimal as a percent. 11. 0.59

Thinking Mathematically (6th Edition)

The following set of data is from sample of n=5: a. Compute the mean, median, and mode. b. Compute the range, v...

Basic Business Statistics, Student Value Edition

Fill in each blanks so that the resulting statement is true. Any set of ordered pairs is called a/an _______. T...

College Algebra (7th Edition)

In Exercises 25–28, use the confidence interval to find the margin of error and the sample mean. 25. (12.0, 14....

Elementary Statistics: Picturing the World (7th Edition)

Knowledge Booster

$Calculus$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.