
Concept explainers
A Rotating Beacon Suppose that a fire truck is parked in front of a building as shown in the figure.
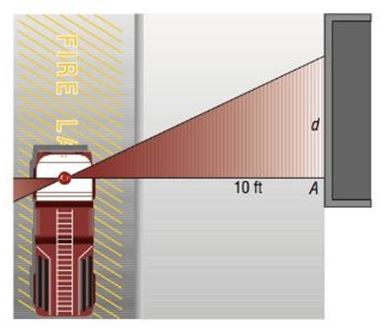
The beacon light on top of the fire truck is located 10 feet from the wall and has a light on each side. If the beacon light rotates 1 revolution every 2 seconds, then a model for determining the distance , in feet, that the beacon of light is from point A on the wall after seconds is given by
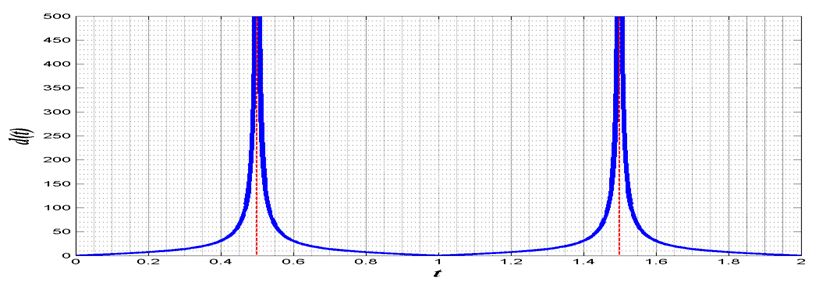
(a) Graph for .
(b) For what values of is the function undefined? Explain what this means in terms of the beam of light on the wall.
(c) Fill in the following table.
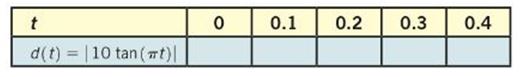
(d) Compute , and so on, for each consecutive value of . These are called first differences.
(e) Interpret the first differences found in part . What is happening to the speed of the beam of light as increases?
To find:
a. Graph for .
Answer to Problem 52AYU
Solution:
a.
Explanation of Solution
Given:
The function .
Calculation:
a. Graphing the given expression in either a graphing calculator, or using the method outlined in the solutions and using the vertical reflection operation needed to reflect the negative halves of the tangent into the positive side of the y-axis for the absolute value operation. We obtain the following graph.

To find:
b. For what values of t is the function undefined? Explain what this means in terms of the beam of light o the wall.
Answer to Problem 52AYU
Solution:
b.
Explanation of Solution
Given:
The function .
Calculation:
b. since , this function is undefined when for integer . in the interval provided the undefined values are going to be:
, from this list we choose the first two, since the third is outside the range given.
In terms of the light these times correspond to the times when the light beam is parallel to the wall, where it intersect the wall at infinity, which makes for either time point. Assuming the light is rotating counterclockwise in the figure given, and noting that for , which corresponds to time when the light is pointing straight at the wall at point , then corresponding to the time when the light is pointing directly in the front of truck parallel to the wall. Then one second after that (the time it takes that same beam to traverse the other half of the rotation)the beam is pointing directly behind the truck, parallel to the wall.
To find:
c. Fill in the following table.
Answer to Problem 52AYU
Solution:
c.
Explanation of Solution
Given:
The function .
Calculation:
c.
To find:
d. Compute and so on, for each consecutive value of .
Answer to Problem 52AYU
Solution:
d.
Explanation of Solution
Given:
The function .
Calculation:
d.
To find:
e. Interpret the first differences found in part d. What is happening to the speed of the beam of light as increases?
Answer to Problem 52AYU
Solution:
e. The results in part d are like an approximation to the velocity of the light beam along width of the wall. We see that as the beam travels further away from point , the of the beam increases, and is in fact nonlinear in its variation (since for equal increments in time the differences in these first differences is not uniform). In fact as we approach the point where the light detaches from the wall at infinity the velocity increases all the way to infinity, as told by the converging upon the vertical line of the asymptote.
Explanation of Solution
Given:
The function .
Calculation:
e. The results in part d are like an approximation to the velocity of the light beam along width of the wall. We see that as the beam travels further away from point , the of the beam increases, and is in fact nonlinear in its variation (since for equal increments in time the differences in these first differences is not uniform). In fact as we approach the point where the light detaches from the wall at infinity the velocity increases all the way to infinity, as told by the converging upon the vertical line of the asymptote.
Chapter 6 Solutions
Precalculus
Additional Math Textbook Solutions
Calculus: Early Transcendentals (2nd Edition)
Basic Business Statistics, Student Value Edition
Elementary Statistics: Picturing the World (7th Edition)
University Calculus: Early Transcendentals (4th Edition)
Pre-Algebra Student Edition
College Algebra with Modeling & Visualization (5th Edition)
- 8–23. Sketching vector fields Sketch the following vector fieldsarrow_forward25-30. Normal and tangential components For the vector field F and curve C, complete the following: a. Determine the points (if any) along the curve C at which the vector field F is tangent to C. b. Determine the points (if any) along the curve C at which the vector field F is normal to C. c. Sketch C and a few representative vectors of F on C. 25. F = (2½³, 0); c = {(x, y); y − x² = 1} 26. F = x (23 - 212) ; C = {(x, y); y = x² = 1}) , 2 27. F(x, y); C = {(x, y): x² + y² = 4} 28. F = (y, x); C = {(x, y): x² + y² = 1} 29. F = (x, y); C = 30. F = (y, x); C = {(x, y): x = 1} {(x, y): x² + y² = 1}arrow_forward٣/١ B msl kd 180 Ka, Sin (1) I sin () sin(30) Sin (30) اذا ميريد شرح الكتب بس 0 بالفراغ 3) Cos (30) 0.866 4) Rotating 5) Synchronous speed, 120 x 50 G 5005 1000 s = 1000-950 Copper bosses 5kW Rotor input 5 0.05 : loo kw 6) 1 /0001 ined sove in peaper I need a detailed solution on paper please وه اذا ميريد شرح الكتب فقط ١٥٠ DC 7) rotor a ' (y+xlny + xe*)dx + (xsiny + xlnx + dy = 0. Q1// Find the solution of: ( 357arrow_forward
- ۳/۱ R₂ = X2 2) slots per pole per phase 3/31 B. 180 msl Kas Sin (I) 1sin() sin(30) Sin (30) اذا ميريد شرح الكتب بس 0 بالفراغ 3) Cos (30): 0.866 4) Rotating 5) Synchronous speeds 120×50 looo G 1000-950 1000 Copper losses 5kw Rotor input 5 loo kw 0.05 6) 1 اذا ميريد شرح الكتب فقط look 7) rotor DC ined sove in peaper I need a detailed solution on paper please 0 64 Find the general solution of the following equations: QI//y(4)-16y= 0. Find the general solution of the following equations: Q2ll yll-4y/ +13y=esinx.arrow_forwardR₂ = X2 2) slots per pole per phase = 3/31 B-180 60 msl kd Kas Sin () 2 I sin (6) sin(30) Sin (30) اذا مريد شرح الكتب بس 0 بالفراغ 3 Cos (30) 0.866 4) Rotating ined sove in peaper 5) Synchronous speed s 120×50 6 s = 1000-950 1000 Copper losses 5kw Rotor input 5 0.05 6) 1 loo kw اذا ميريد شرح الكتب فقط Look 7) rotov DC I need a detailed solution on paper please 0 64 Solve the following equations: 0 Q1// Find the solution of: ( y • with y(0) = 1. dx x²+y²arrow_forwardR₂ = X2 2) slots per pole per phase = 3/3 1 B-180-60 msl Ka Sin (1) Isin () sin(30) Sin (30) اذا ميريد شرح الكتب بس 0 بالفراغ 3) Cos (30) 0.866 4) Rotating 5) Synchronous speed, 120 x 50 s = 1000-950 1000 Copper losses 5kw Rotor input 5 6) 1 0.05 G 50105 loo kw اذا ميريد شرح الكتب فقط look 7) rotov DC ined sove in peaper I need a detailed solution on paper please 064 2- A hot ball (D=15 cm ) is cooled by forced air T.-30°C, the rate of heat transfer from the ball is 460.86 W. Take for the air -0.025 Wim °C and Nu=144.89, find the ball surface temperature a) 300 °C 16 b) 327 °C c) 376 °C d) None か = 750 01arrow_forward
- Don't do 14. Please solve 19arrow_forwardPlease solve 14 and 15arrow_forward1. Consider the following system of equations: x13x2 + 4x3 - 5x4 = 7 -2x13x2 + x3 - 6x4 = 7 x16x213x3 - 21x4 = 28 a) Solve the system. Write your solution in parametric and vector form. b) What is a geometric description of the solution. 7 c) Is v = 7 in the span of the set S= [28. 1 HE 3 -5 3 ·6 ? If it is, write v 6 as a linear combination of the vectors in S. Justify. d) How many solutions are there to the associated homogeneous system for the system above? Justify. e) Let A be the coefficient matrix from the system above. Find the set of all solutions to Ax = 0. f) Is there a solution to Ax=b for all b in R³? Justify.arrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





