Geometry For Enjoyment And Challenge
91st Edition
ISBN: 9780866099653
Author: Richard Rhoad, George Milauskas, Robert Whipple
Publisher: McDougal Littell
expand_more
expand_more
format_list_bulleted
Videos
Question
Chapter 5.4, Problem 2PSA
a.
To determine
To find:The statement opposite sides of a kiteKITEare congruent is true.
a.
Expert Solution
Answer to Problem 2PSA
The statementopposite sides are congruent is false.
Explanation of Solution
Given information:
A KITE with sides KI, IT, TE and KE.
Diagonals ofkiteKITEare KTand IE.
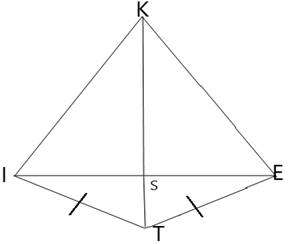
Two disjoints pairs of consecutive sides are congruent.
b.
To determine
To find: The statement opposite sides of a kite KITEare parallel is true.
b.
Expert Solution
Answer to Problem 2PSA
The statement opposite sides are parallel is false.
Explanation of Solution
Given information:
A KITE with sides KI, IT, TE and KE.
Diagonals of kite KITEare KT and IE.
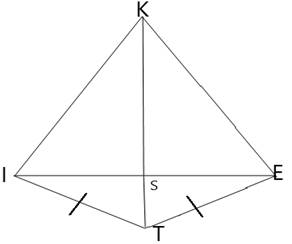
A kite has got two pairs of sides next to each other that have equal length but none of the sides are parallel.
c.
To determine
To find:The statement diagonals KTand IE of a kite KITE bisect the
c.
Expert Solution
Answer to Problem 2PSA
The statement diagonals KT and IEbisect the angles is true.
Explanation of Solution
Given information:
A KITE with sides KI, IT, TE and KE.
Diagonals of kite KITEare KT and IE.
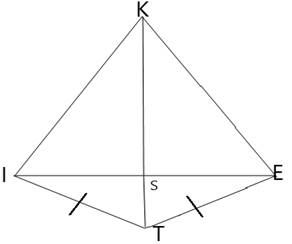
If a quadrilateral is kite, it has one diagonal that bisects a pair of opposite angles.
d.
To determine
To find:The statement diagonals KT and IE of a kite KITEbisect each other is true.
d.
Expert Solution
Answer to Problem 2PSA
The statement diagonals KTand IE bisect each other is true.
Explanation of Solution
Given information:
A KITE with sides KI, IT, TE and KE.
Diagonals of kite KITEare KT and IE.
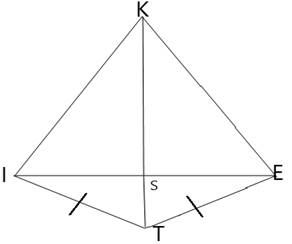
If a quadrilateral is kite, it has one diagonal that bisects the other diagonal.
Diagonals KT and IE bisect each other at point S.
e.
To determine
To find:The statement diagonals KT and IE of a kite KITEare congruent is true.
e.
Expert Solution
Answer to Problem 2PSA
The statement diagonals KT and IEare congruent is false.
Explanation of Solution
Given information:
A KITE with sides KI, IT, TE and KE.
Diagonals of kite KITEare KT and IE.
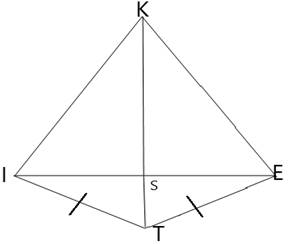
The consecutive sides are congruent but the diagonals are not congruent.
Diagonals KT and IEare not congruent.
f.
To determine
To find:The statement diagonals KT and IE of a kite KITE are perpendicular is true.
f.
Expert Solution
Answer to Problem 2PSA
The statement diagonals KT and IE are perpendicular is true.
Explanation of Solution
Given information:
A KITE with sides KI, IT, TE and KE.
Diagonals of kite KITEare KT and IE.
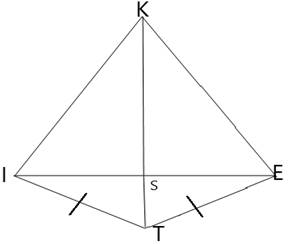
The intersection of the diagonals of a kite form right angles. This means that they are perpendicular. The longer diagonal of a kite bisects the shorter one. This means that the longer diagonal cuts the shorter one in half.
Chapter 5 Solutions
Geometry For Enjoyment And Challenge
Ch. 5.1 - Prob. 1PSACh. 5.1 - Prob. 2PSACh. 5.1 - Prob. 3PSACh. 5.1 - Prob. 4PSACh. 5.1 - Prob. 5PSACh. 5.1 - Prob. 6PSACh. 5.1 - Prob. 7PSACh. 5.1 - Prob. 8PSACh. 5.1 - Prob. 9PSACh. 5.1 - Prob. 10PSB
Ch. 5.1 - Prob. 11PSBCh. 5.1 - Prob. 12PSBCh. 5.1 - Prob. 13PSBCh. 5.1 - Prob. 14PSCCh. 5.1 - Prob. 15PSCCh. 5.2 - Prob. 1PSACh. 5.2 - Prob. 2PSACh. 5.2 - Prob. 3PSACh. 5.2 - Prob. 4PSACh. 5.2 - Prob. 5PSACh. 5.2 - Prob. 6PSACh. 5.2 - Prob. 7PSACh. 5.2 - Prob. 8PSACh. 5.2 - Prob. 9PSACh. 5.2 - Prob. 10PSACh. 5.2 - Prob. 11PSACh. 5.2 - Prob. 12PSACh. 5.2 - Prob. 13PSACh. 5.2 - Prob. 14PSACh. 5.2 - Prob. 15PSACh. 5.2 - Prob. 16PSACh. 5.2 - Prob. 17PSACh. 5.2 - Prob. 18PSACh. 5.2 - Prob. 19PSACh. 5.2 - Prob. 20PSACh. 5.2 - Prob. 21PSACh. 5.2 - Prob. 22PSBCh. 5.2 - Prob. 23PSBCh. 5.2 - Prob. 24PSBCh. 5.2 - Prob. 25PSCCh. 5.2 - Prob. 26PSCCh. 5.2 - Prob. 27PSCCh. 5.2 - Prob. 28PSCCh. 5.3 - Prob. 1PSACh. 5.3 - Prob. 2PSACh. 5.3 - Prob. 3PSACh. 5.3 - Prob. 4PSACh. 5.3 - Prob. 5PSACh. 5.3 - Prob. 6PSACh. 5.3 - Prob. 7PSACh. 5.3 - Prob. 8PSACh. 5.3 - Prob. 9PSACh. 5.3 - Prob. 10PSACh. 5.3 - Prob. 11PSACh. 5.3 - Prob. 12PSACh. 5.3 - Prob. 13PSACh. 5.3 - Prob. 14PSBCh. 5.3 - Prob. 15PSBCh. 5.3 - Prob. 16PSBCh. 5.3 - Prob. 17PSBCh. 5.3 - Prob. 18PSBCh. 5.3 - Prob. 19PSBCh. 5.3 - Prob. 20PSBCh. 5.3 - Prob. 21PSBCh. 5.3 - Prob. 22PSBCh. 5.3 - Prob. 23PSBCh. 5.3 - Prob. 24PSBCh. 5.3 - Prob. 25PSBCh. 5.3 - Prob. 26PSCCh. 5.3 - Prob. 27PSCCh. 5.3 - Prob. 28PSCCh. 5.3 - Prob. 29PSDCh. 5.3 - Prob. 30PSDCh. 5.4 - Prob. 1PSACh. 5.4 - Prob. 2PSACh. 5.4 - Prob. 3PSACh. 5.4 - Prob. 4PSACh. 5.4 - Prob. 5PSACh. 5.4 - Prob. 6PSACh. 5.4 - Prob. 7PSACh. 5.4 - Prob. 8PSACh. 5.4 - Prob. 9PSACh. 5.4 - Prob. 10PSACh. 5.4 - Prob. 11PSACh. 5.4 - Prob. 12PSACh. 5.4 - Prob. 13PSBCh. 5.4 - Prob. 14PSBCh. 5.4 - Prob. 15PSBCh. 5.4 - Prob. 16PSBCh. 5.4 - Prob. 17PSBCh. 5.4 - Prob. 18PSBCh. 5.4 - Prob. 19PSBCh. 5.4 - Prob. 20PSBCh. 5.4 - Prob. 21PSCCh. 5.4 - Prob. 22PSCCh. 5.5 - Prob. 1PSACh. 5.5 - Prob. 2PSACh. 5.5 - Prob. 3PSACh. 5.5 - Prob. 4PSACh. 5.5 - Prob. 5PSACh. 5.5 - Prob. 6PSACh. 5.5 - Prob. 7PSACh. 5.5 - Prob. 8PSACh. 5.5 - Prob. 9PSACh. 5.5 - Prob. 10PSACh. 5.5 - Prob. 11PSACh. 5.5 - Prob. 12PSACh. 5.5 - Prob. 13PSACh. 5.5 - Prob. 14PSACh. 5.5 - Prob. 15PSBCh. 5.5 - Prob. 16PSBCh. 5.5 - Prob. 17PSBCh. 5.5 - Prob. 18PSBCh. 5.5 - Prob. 19PSBCh. 5.5 - Prob. 20PSBCh. 5.5 - Prob. 21PSBCh. 5.5 - Prob. 22PSBCh. 5.5 - Prob. 23PSBCh. 5.5 - Prob. 24PSBCh. 5.5 - Prob. 25PSBCh. 5.5 - Prob. 26PSBCh. 5.5 - Prob. 27PSBCh. 5.5 - Prob. 28PSCCh. 5.5 - Prob. 29PSCCh. 5.5 - Prob. 30PSCCh. 5.6 - Prob. 1PSACh. 5.6 - Prob. 2PSACh. 5.6 - Prob. 3PSACh. 5.6 - Prob. 4PSACh. 5.6 - Prob. 5PSACh. 5.6 - Prob. 6PSACh. 5.6 - Prob. 7PSACh. 5.6 - Prob. 8PSACh. 5.6 - Prob. 9PSACh. 5.6 - Prob. 10PSACh. 5.6 - Prob. 11PSBCh. 5.6 - Prob. 12PSBCh. 5.6 - Prob. 13PSBCh. 5.6 - Prob. 14PSBCh. 5.6 - Prob. 15PSBCh. 5.6 - Prob. 16PSBCh. 5.6 - Prob. 17PSBCh. 5.6 - Prob. 18PSBCh. 5.6 - Prob. 19PSCCh. 5.6 - Prob. 20PSCCh. 5.6 - Prob. 21PSDCh. 5.7 - Prob. 1PSACh. 5.7 - Prob. 2PSACh. 5.7 - Prob. 3PSACh. 5.7 - Prob. 4PSACh. 5.7 - Prob. 5PSACh. 5.7 - Prob. 6PSACh. 5.7 - Prob. 7PSACh. 5.7 - Prob. 8PSACh. 5.7 - Prob. 9PSACh. 5.7 - Prob. 10PSACh. 5.7 - Prob. 11PSBCh. 5.7 - Prob. 12PSBCh. 5.7 - Prob. 13PSBCh. 5.7 - Prob. 14PSBCh. 5.7 - Prob. 15PSBCh. 5.7 - Prob. 16PSBCh. 5.7 - Prob. 17PSBCh. 5.7 - Prob. 18PSBCh. 5.7 - Prob. 19PSBCh. 5.7 - Prob. 20PSBCh. 5.7 - Prob. 21PSBCh. 5.7 - Prob. 22PSBCh. 5.7 - Prob. 23PSBCh. 5.7 - Prob. 24PSBCh. 5.7 - Prob. 25PSBCh. 5.7 - Prob. 26PSCCh. 5.7 - Prob. 27PSCCh. 5.7 - Prob. 28PSCCh. 5.7 - Prob. 29PSCCh. 5 - Prob. 1RPCh. 5 - Prob. 2RPCh. 5 - Prob. 3RPCh. 5 - Prob. 4RPCh. 5 - Prob. 5RPCh. 5 - Prob. 6RPCh. 5 - Prob. 7RPCh. 5 - Prob. 8RPCh. 5 - Prob. 9RPCh. 5 - Prob. 10RPCh. 5 - Prob. 11RPCh. 5 - Prob. 12RPCh. 5 - Prob. 13RPCh. 5 - Prob. 14RPCh. 5 - Prob. 15RPCh. 5 - Prob. 16RPCh. 5 - Prob. 17RPCh. 5 - Prob. 18RPCh. 5 - Prob. 19RPCh. 5 - Prob. 20RPCh. 5 - Prob. 21RPCh. 5 - Prob. 22RPCh. 5 - Prob. 23RPCh. 5 - Prob. 24RPCh. 5 - Prob. 25RPCh. 5 - Prob. 26RPCh. 5 - Prob. 27RPCh. 5 - Prob. 28RPCh. 5 - Prob. 29RPCh. 5 - Prob. 30RP
Additional Math Textbook Solutions
Find more solutions based on key concepts
A pair of fair dice is rolled. What is the probability that the second die lands on a higher value than does th...
A First Course in Probability (10th Edition)
Voting A random sample of likely voters showed that 49 planned to support Measure X. The margin of error is 3 p...
Introductory Statistics
Time-lagged flights An airliner passes over an airport at noon traveling 500 mi/hr due west. At l:00 P.M., anot...
Calculus: Early Transcendentals (2nd Edition)
Fill in each blank so that the resulting statement is true. If n is a counting number, bn, read ______, indicat...
College Algebra (7th Edition)
CHECK POINT I Express as a percent.
Thinking Mathematically (6th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, geometry and related others by exploring similar questions and additional content below.Similar questions
- 5:00 PM Sat May 3 deltamath.com DeltaMath Given: ABBC and D is the midpoint of AC. Prove: ABD ≈ ACBD. ← Back to Home Deltamath Regents Review Week 3 Due: May 9 at 8:00 PM Grade: 97% Step Statement AB ≈ BC Reason 1 Given D is the midpoint of AC 2 BD BD 3 ADDC Calculating Volume (Mixed) Volume of Oblique Solids Volume, Density, and Unit 5 4 AABC is an isosceles triangle ZAZC Conversions (Level 1) Triangle Congruence Criteria try ZAD =/ DC Basic Triangle Proofs (Congruence Only - No CPCTC) Triangle Proofs (Reasons Only) Calculator Aseret Martinez Domi... Log Out Reflexive Property A midpoint divides a segment into two congruent segments The triangle has two congruent sides In a triangle, angles opposite of congruent sides are congruent An angle bisector divides an angle into two congruent angles B * A Ꭰ Note: the segment AC is a straight segment. 86%arrow_forwardLANDMARKS Stonehenge is a British landmark made of huge stones arranged in a circular pattern that reflects the movements of Earth and the moon. The diagram shows that the angle formed by the north/south axis and the line aligned from the station stone to the northmost moonrise position measures 23.5°. a. Find measure of arc BC. b. Is arc ABC semicircle? Explain. c. If the circle measures about 100 feet across, approximately how far would you walk around the circle from point B to point sarsen circle B station stone trilithons horseshoe 71° 23.5° farthest north moonrise Sarrow_forwardfind the value of each variablearrow_forward
- Name: Date: Bell: Unit 11: Volume & Surface Area Homework 2: Area of Sectors Directions: Find the area of each shaded sector. Round to the hundredths place. 1. GH 11 in 2. KL 20 ft H F 64 G L 119 M K 3. BA 6.5 cm 4. YZ 14.2 m B 23 X 87° Y Z 5. KL = 27.1 mm J 32 L X:360-32.1 K A-3 360 7. BD 18 cm E 136 B X=32.8 127.0 (271) A: 069.13 Amm² 19=2102.13 A-136 360.16912 A:300cm² A=96.13 6. PQ = 2.8 in P R 311° 8. WZ 5.3 km V = Z 108 W D 9. HK = 25 ft G H KO 26 X 10. SR 26 m = S 73 T R Gina Wilson (All Things Algebarrow_forward538 Chapter 13 12. Given: Points E(-4, 1), F(2, 3), G(4, 9), and H(-2, 7) a. Show that EFGH is a rhombus. b. Use slopes to verify that the diagonals are perpendicular. 13. Given: Points R(-4, 5), S(-1, 9), T(7, 3) and U(4, -1) a. Show that RSTU is a rectangle. b. Use the distance formula to verify that the diagonals are congruent. 14. Given: Points N(-1, -5), O(0, 0), P(3, 2), and 2(8, 1) a. Show that NOPQ is an isosceles trapezoid. b. Show that the diagonals are congruent. Decide what special type of quadrilateral HIJK is. Then prove that your answer is correct. 15. H(0, 0) 16. H(0, 1) 17. H(7, 5) 18. H(-3, -3) I(5, 0) I(2,-3) 1(8, 3) I(-5, -6) J(7, 9) K(1, 9) J(-2, -1) K(-4, 3) J(0, -1) K(-1, 1) J(4, -5) K(6,-2) 19. Point N(3, - 4) lies on the circle x² + y² = 25. What is the slope of the (Hint: Recall Theorem 9-1.) - line that is tangent to the circle at N? 20. Point P(6, 7) lies on the circle (x + 2)² + (y − 1)² = 100. What is the slope of the line that is tangent to the circle at…arrow_forwardCan you cut the 12 glass triangles from a sheet of glass that is 4 feet by 8 feet? If so, how can it be done?arrow_forward
- Can you cut 12 glass triangles from a sheet of glass that is 4 feet by 8 feet? If so, draw a diagram of how it can be done.arrow_forwardIn triangle with sides of lengths a, b and c the angle a lays opposite to a. Prove the following inequality sin a 2√bc C α b a Warrow_forwardFind the values of x, y, and z. Round to the nearest tenth, if necessary. 8, 23arrow_forward
- 11 In the Pharlemina's Favorite quilt pattern below, vega-pxe-frame describe a motion that will take part (a) green to part (b) blue. Part (a) Part (b)arrow_forward5. 156 m/WXY = 59° 63 E 7. B E 101 C mFE = 6. 68° 8. C 17arrow_forward1/6/25, 3:55 PM Question: 14 Similar right triangles EFG and HIJ are shown. re of 120 √65 adjacent E hypotenuse adjaca H hypotenuse Item Bank | DnA Er:nollesup .es/prist Sisupe ed 12um jerit out i al F 4 G I oppe J 18009 90 ODPO ysma brs & eaus ps sd jon yem What is the value of tan J? ed on yem O broppo 4 ○ A. √65 Qx oppoEF Adj art saused taupe ed for yem 4 ○ B. √65 29 asipnisht riod 916 zelprisht rad √65 4 O ○ C. 4 √65 O D. VIS 9 OD elimiz 916 aelonsider saused supsarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning
What are the Different Types of Triangles? | Don't Memorise; Author: Don't Memorise;https://www.youtube.com/watch?v=1k0G-Y41jRA;License: Standard YouTube License, CC-BY
Law of Sines AAS, ASA, SSA Ambiguous Case; Author: Mario's Math Tutoring;https://www.youtube.com/watch?v=FPVGb-yWj3s;License: Standard YouTube License, CC-BY
Introduction to Statistics..What are they? And, How Do I Know Which One to Choose?; Author: The Doctoral Journey;https://www.youtube.com/watch?v=HpyRybBEDQ0;License: Standard YouTube License, CC-BY
Triangles | Mathematics Grade 5 | Periwinkle; Author: Periwinkle;https://www.youtube.com/watch?v=zneP1Q7IjgQ;License: Standard YouTube License, CC-BY
What Are Descriptive Statistics And Inferential Statistics?; Author: Amour Learning;https://www.youtube.com/watch?v=MUyUaouisZE;License: Standard Youtube License