
Concept explainers
a.
To Find: How many pairs of
a.
Answer to Problem 26PSC
14 pairs of angles are formed, when two parallel lines are intersected by a transversal excluding linear pair of angles.
Explanation of Solution
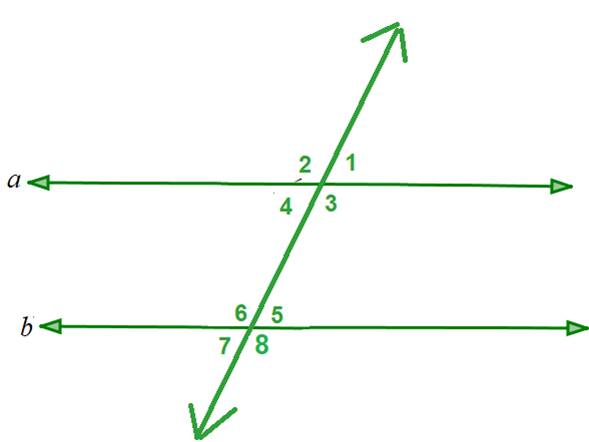
Given:
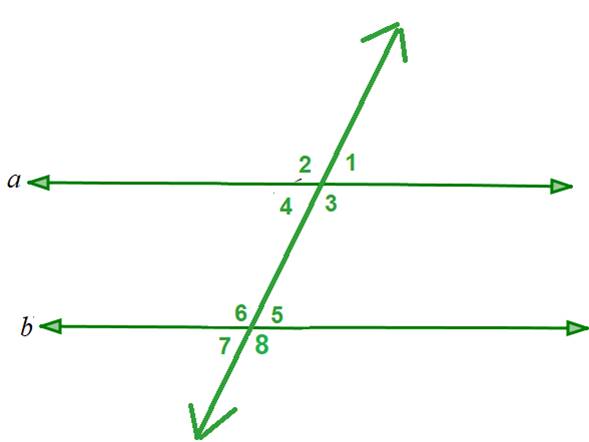
Concept Used:
When two parallel lines are intersected by a transversal following angles formed:
- Pair of corresponding angles
- Pair of alternate interior angles
- Pair of alternate exterior angles
- Pair of supplementary angles
- Pair of vertically opposite angles
Calculation:
Here, we have
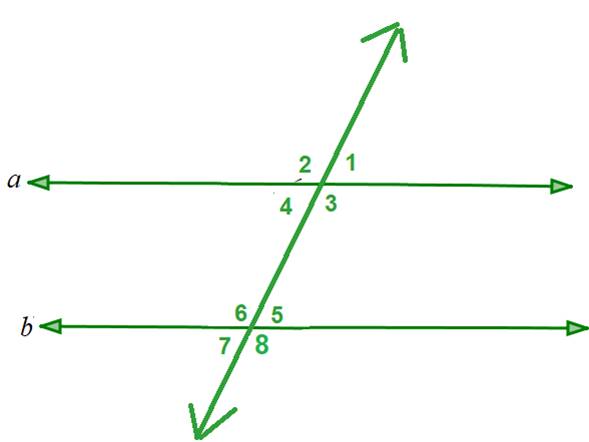
Since, we know that when two parallel lines are intersected by a transversal following angles formed:
- Pair of corresponding angles
- Pair of alternate interior angles
- Pair of alternate exterior angles
- Pair of supplementary angles
- Pair of vertically opposite angles
Thus, there are 14 pairs of angles formed, when two parallel lines intersected by a transversal.
b.
To Find: The probability of choosing pairs of alternate interior or pairs of exterior angles of pairs of corresponding angles.
b.
Answer to Problem 26PSC
Probability of choosing pairs of alternate interior or pairs of exterior angles of pairs of corresponding angles
Explanation of Solution
Given:
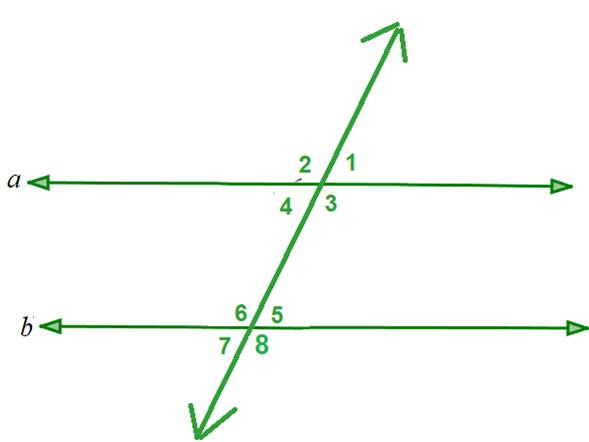
Concept Used:
When two parallel lines are intersected by a transversal following angles formed:
- Pair of corresponding angles
- Pair of alternate interior angles
- Pair of alternate exterior angles
- Pair of supplementary angles
- Pair of vertically opposite angles
Calculation:
Here, we have
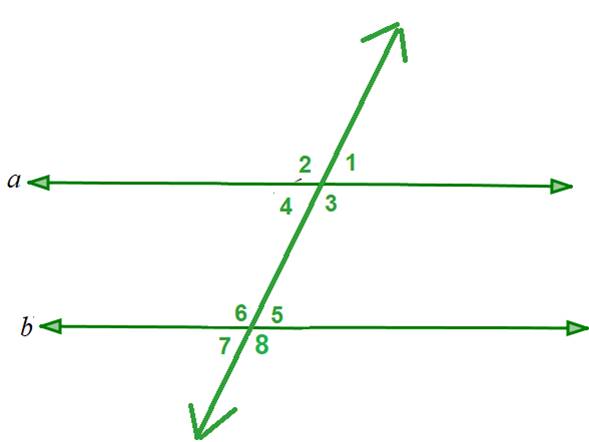
Since, we know that when two parallel lines are intersected by a transversal following angles formed:
- Pair of corresponding angles
- Pair of alternate interior angles
- Pair of alternate exterior angles
- Pair of supplementary angles
- Pair of vertically opposite angles
Hence, the probability of choosing pairs of alternate interior or pairs of exterior angles of pairs of corresponding angles
c.
To Find: The probability of choosing pairs of supplementary angles.
c.
Answer to Problem 26PSC
Explanation of Solution
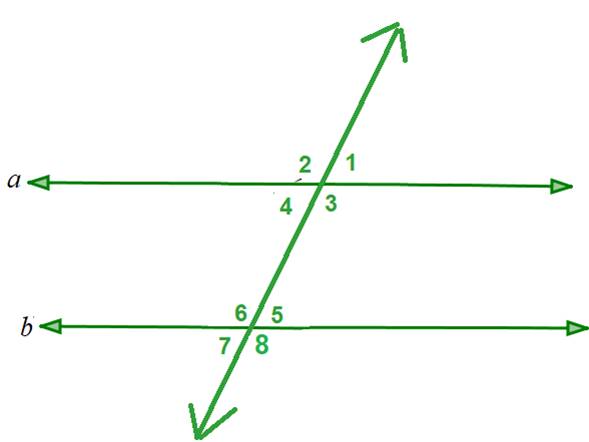
Given:
Concept Used:
When two parallel lines are intersected by a transversal following angles formed:
- Pair of corresponding angles
- Pair of alternate interior angles
- Pair of alternate exterior angles
- Pair of supplementary angles
- Pair of vertically opposite angles
Calculation:
Here, we have
Since, we know that when two parallel lines are intersected by a transversal following angles formed:
- Pair of corresponding angles
- Pair of alternate interior angles
- Pair of alternate exterior angles
- Pair of supplementary angles
- Pair of vertically opposite angles
Chapter 5 Solutions
Geometry For Enjoyment And Challenge
Additional Math Textbook Solutions
Elementary Statistics
Introductory Statistics
College Algebra (7th Edition)
Pre-Algebra Student Edition
Calculus: Early Transcendentals (2nd Edition)
Precalculus
- 14:09 2/16 jmap.org 5G 66 In the accompanying diagram of circle O, diameters BD and AE, secants PAB and PDC, and chords BC and AD are drawn; mAD = 40; and mDC = 80. B E Find: mAB, m/BCD, m/BOE, m/P, m/PAD ← G.C.A.2.ChordsSecantsand Tangent s19.pdf (538 KB) + 4 保存... Xarrow_forward16:39 < 文字 15:28 |美图秀秀 保存 59% 5G 46 照片 完成 Bonvicino - Period Name: 6. A right regular hexagonal pyramid with the top removed (as shown in Diagram 1) in such a manner that the top base is parallel to the base of the pyramid resulting in what is shown in Diagram 2. A wedge (from the center) is then removed from this solid as shown in Diagram 3. 30 Diogram 1 Diegrom 2. Diagram 3. If the height of the solid in Diagrams 2 and 3 is the height of the original pyramid, the radius of the base of the pyramid is 10 cm and each lateral edge of the solid in Diagram 3 is 12 cm, find the exact volume of the solid in Diagram 3, measured in cubic meters. Show all work. (T 文字 贴纸 消除笔 涂鸦笔 边框 马赛克 去美容arrow_forwardAnswer question 4 pleasearrow_forward
- 16:39 < 文字 15:28 |美图秀秀 保存 59% 5G 46 照片 完成 Bonvicino - Period Name: 6. A right regular hexagonal pyramid with the top removed (as shown in Diagram 1) in such a manner that the top base is parallel to the base of the pyramid resulting in what is shown in Diagram 2. A wedge (from the center) is then removed from this solid as shown in Diagram 3. 30 Diogram 1 Diegrom 2. Diagram 3. If the height of the solid in Diagrams 2 and 3 is the height of the original pyramid, the radius of the base of the pyramid is 10 cm and each lateral edge of the solid in Diagram 3 is 12 cm, find the exact volume of the solid in Diagram 3, measured in cubic meters. Show all work. (T 文字 贴纸 消除笔 涂鸦笔 边框 马赛克 去美容arrow_forwardAnswer question 3 pleasearrow_forwardAnswer question 3 pleasearrow_forward
- 38 Below triangle is isosceles. Find the value of x. 70° 60° x 20° Warrow_forwardTo approximate the length of a marsh, a surveyor walks x = 400 meters from point A to point B. Then, the surveyor turns 75° and walks 220 meters to point C (see figure). Approximate the length AC of the marsh. (Round your answer to one decimal place.) m C B 75° 220 m x marrow_forward. The students who attend Memorial High School have a wide variety of extra-curricular activities to choose from in the after-school program. Students are 38% likely to join the dance team; 18% likely to participate in the school play; 42% likely to join the yearbook club; and 64% likely to join the marching band. Many students choose to participate in multiple activities. Students have equal probabilities of being freshmen, sophomores, juniors, or seniors.What is the probability of the union of being either a freshman or senior? 0.07 0.44 0.50 0.25arrow_forward
- Which angles are complementary to each other? Select all that apply. 3 2 4 in 5 1 Z1 and 23 Z1 and 25 22 and 23 Z2 and 25 Submitarrow_forwardWhich angles are adjacent to each other? Select all that apply. 3 2 4 67 5 8 11 10 12 12 9 27 and 28 Z9 and 12 Z3 and 24 Z10 and Z11arrow_forwardIf the arc length of NMP is 11π, what is the length of MNP expressed in terms of πT? M N 5 44% ○ A. 54π OB. 108π P О с. 103 18 O D. 108arrow_forward
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning

