College Physics: A Strategic Approach (3rd Edition)

3rd Edition

ISBN: 9780321879721

Author: Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field

Publisher: PEARSON

See similar textbooks

Concept explainers

Newton’s Third Law of Motion

The horse pulls the cart and the cart pulls the horse in response as per Newton third law. So the big question is, “Don’t the two forces cancel each other? How does the cart accelerate?”

Videos

Textbook Question

Chapter 4, Problem 69MSPP

If your car is stuck in the mud and you don't have a winch to pull it out, you can use a piece of rope and a tree to do the trick. First, you tie one end of the rope to your car and the other to a tree, then pull as hard as you can on the middle of the rope, as shown in Figure P4.68 a. This technique applies a force to the car much larger than the force that you can apply directly. To see why the car experiences such a large force, look at the forces acting on the center point of the rope, as shown in Figure P4.68 b. The sum of the forces is zero, thus the tension is much greater than the force you apply. It is this tension force that acts on the car and, with luck, pulls it free.

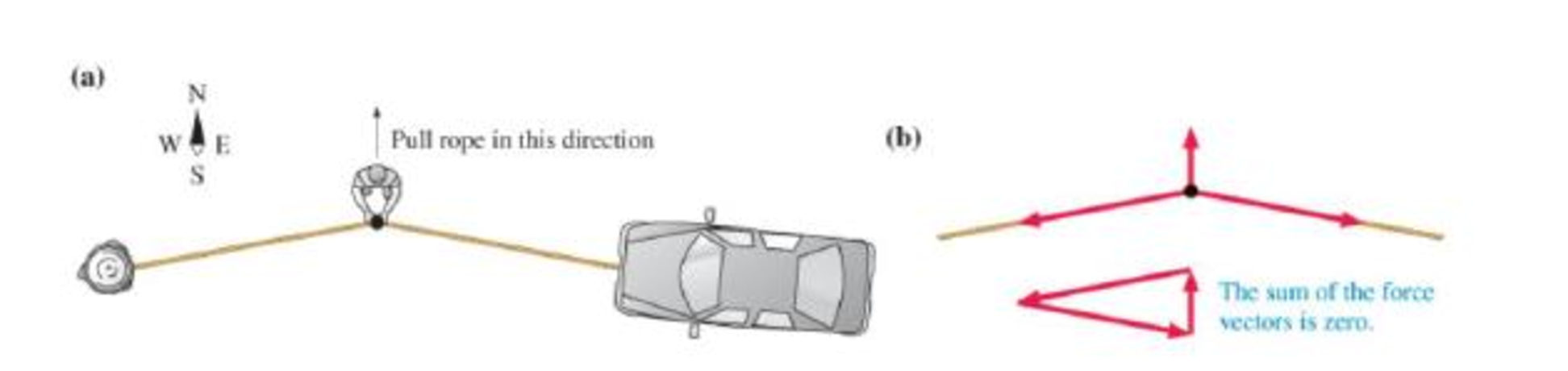
Chapter 4, Problem 69MSPP, If your car is stuck in the mud and you don't have a winch to pull it out, you can use a piece of

Figure P4.68

69. When you are pulling on the rope as shown, what is the approximate direction of the tension force on the tree?

A. North

B. South

C. East

D. West

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 4, Problem 68MSPP

Chapter 4, Problem 70MSPP

Students have asked these similar questions

1. A charge of -25 μC is distributed uniformly throughout a spherical volume of radius 11.5 cm. Determine the electric field due to this charge at a distance of (a) 2 cm, (b) 4.6 cm, and (c) 25 cm from the center of the sphere. (a) = = (b) E = (c)Ẻ = = NC NC NC

1. A long silver rod of radius 3.5 cm has a charge of -3.9 ис on its surface. Here ŕ is a unit vector ст directed perpendicularly away from the axis of the rod as shown in the figure. (a) Find the electric field at a point 5 cm from the center of the rod (an outside point). E = N C (b) Find the electric field at a point 1.8 cm from the center of the rod (an inside point) E=0 Think & Prepare N C 1. Is there a symmetry in the charge distribution? What kind of symmetry? 2. The problem gives the charge per unit length 1. How do you figure out the surface charge density σ from a?

1. Determine the electric flux through each surface whose cross-section is shown below. 55 S₂ -29 S5 SA S3 + 9 Enter your answer in terms of q and ε Φ (a) s₁ (b) s₂ = -29 (C) Φ զ Ερ (d) SA = (e) $5 (f) Sa $6 = II ✓ -29 S6 +39

Chapter 4 Solutions

College Physics: A Strategic Approach (3rd Edition)

Ch. 4 - If an object is not moving, does that mean that...Ch. 4 - An object moves in a straight line at a constant...Ch. 4 - If you know all of the forces acting on a moving...Ch. 4 - Three arrows are shot horizontally. They have left...Ch. 4 - A carpenter wishes to tighten the heavy head of...Ch. 4 - Internal injuries in vehicular acci-dents may be...Ch. 4 - Heres a great everyday use of the physics...Ch. 4 - Suppose you are an astronaut in deep space, far...Ch. 4 - Jonathan accelerates away from a stop sign. His...Ch. 4 - Normally, jet engines push air out the back of the...

Additional Science Textbook Solutions

Find more solutions based on key concepts

1.3 Obtain a bottle of multivitamins and read the list of ingredients. What are four chemicals from the list?

Chemistry: An Introduction to General, Organic, and Biological Chemistry (13th Edition)

What type of cut would separate the brain into anterior and posterior parts?

Anatomy & Physiology (6th Edition)

Name the components (including muscles) of the thoracic cage. List the contents of the thorax.

Human Physiology: An Integrated Approach (8th Edition)

23. Yellow light of wavelength 590 nm passes through a diffraction grating and makes an interference pattern on...

College Physics: A Strategic Approach (3rd Edition)

In Figure 12.14, why do the nuclei resulting from experiment 2 contain different amounts of DNA?

Campbell Biology (11th Edition)

25. FIGURE EX4.25 shows the angular-velocity-versus-time graph for a particle moving in a circle, starting from...

Physics for Scientists and Engineers: A Strategic Approach, Vol. 1 (Chs 1-21) (4th Edition)

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.