Chapter 2.10, Problem 43E

(a)

To determine

To find: The derivatives of the given function, the critical points, and the point of infection. Also, determine the situation when more and more cosines are piled up and explain it in terms of the updating function $x_{t+1}=\cos \left(x_t\right)$ .

Answer to Problem 43E

The derivate is $\sin \left(\cos \left(x\right)\right)\sin \left(x\right)$ , critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ and point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$ .As the pile increases the graph is flatten and the value is stable.

Explanation of Solution

Given:

The expression is $\cos \left(\cos \left(x\right)\right)$ .

Calculation:

The critical point is the point on the curve where the slope of the tangent is zero and the tangent is parallel to the x axis.

The point of inflexion is the point on the curve at which the sign of the curvature changes.

Consider the given expression is,

  $\cos \left(\cos \left(x\right)\right)$

Differentiate the above equation with respect to $x$ as,

  $\begin{array}{c}{f}^{\prime }\left(x\right)=\frac{d}{dx}\cos \left(\cos \left(x\right)\right)\\ =-\sin \left(\cos \left(x\right)\right)\frac{d}{dx}\left(\cos \left(x\right)\right)\\ =\sin \left(\cos \left(x\right)\right)\sin \left(x\right)\end{array}$

The graph for the above function is shown in Figure 1

  

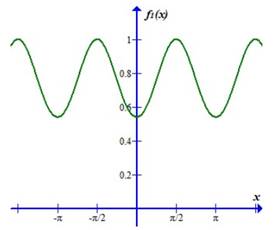
Figure 1

Then, the critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ .

The point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$

The critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ and point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$ .

From the above calculations it is observed that as more and more cosines are piled up the graph of the function is flat and stable at one value.

(b)

To determine

To find: The derivatives of the given function, the critical points, and the point of infection. Also, determine the situation when more and more cosines are piled up and explain it in terms of the updating function $x_{t+1}=\cos \left(x_t\right)$ .

Answer to Problem 43E

The derivate is $\sin \left(x\right)\sin \left(\cos \left(x\right)\right)\sin \left(\cos \cos \left(x\right)\right)$ , critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ and point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$ .As the pile increases the graph is flatten and the value is stable.

Explanation of Solution

Given:

The expression is $\cos \left(\cos \left(\cos \left(x\right)\right)\right)$ .

Calculation:

The critical point is the point on the curve where the slope of the tangent is zero and the tangent is parallel to the x axis.

The point of inflexion is the point on the curve at which the sign of the curvature changes.

Consider the given expression is,

  $\cos \left(\cos \left(\cos \left(x\right)\right)\right)$

Differentiate the above equation with respect to $x$ as,

  $\begin{array}{c}{f}^{\prime }\left(x\right)=\frac{d}{dx}\left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\\ =-\sin \left(\cos \left(\cos \left(x\right)\right)\right)\frac{d}{dx}\left(\left(\cos \left(\cos \left(x\right)\right)\right)\right)\\ =\sin \left(x\right)\sin \left(\cos \left(x\right)\right)\sin \left(\cos \cos \left(x\right)\right)\end{array}$

The graph for the above function is shown in Figure 1

  

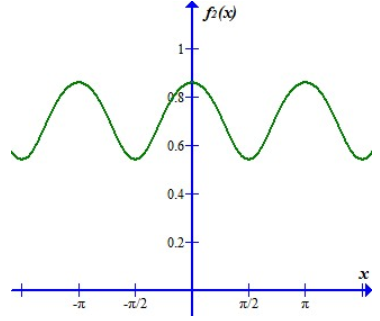
Figure 1

Then, the critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ .

The point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$

The critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ and point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$ .

From the above calculations it is observed that as more and more cosines are piled up the graph of the function is flat and stable at one value.

(c)

To determine

To find: The derivatives of the given function, the critical points, and the point of infection. Also, determine the situation when more and more cosines are piled up and explain it in terms of the updating function $x_{t+1}=\cos \left(x_t\right)$ .

Answer to Problem 43E

The derivate is $\sin \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\sin \left(\cos \left(\cos \left(x\right)\right)\right)\sin \left(\cos \left(\cos \left(x\right)\right)\right)\left(\sin \left(x\right)\right)$ , critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ and point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$ .As the pile increases the graph is flatten and the value is stable.

Explanation of Solution

Given:

The expression is $\cos \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)$ .

Calculation:

The critical point is the point on the curve where the slope of the tangent is zero and the tangent is parallel to the x axis.

The point of inflexion is the point on the curve at which the sign of the curvature changes.

Consider the given expression is,

  $\cos \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)$

Differentiate the above equation with respect to $x$ as,

  $\begin{array}{c}{f}^{\prime }\left(x\right)=\frac{d}{dx}\left(\cos \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\right)\\ =-\sin \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\frac{d}{dx}\left(\left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\right)\\ =-\sin \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\sin \left(\cos \left(\cos \left(x\right)\right)\right)\sin \left(\cos \left(\cos \left(x\right)\right)\right)\frac{d}{dx}\left(\cos \left(x\right)\right)\\ =\sin \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\sin \left(\cos \left(\cos \left(x\right)\right)\right)\sin \left(\cos \left(\cos \left(x\right)\right)\right)\left(\sin \left(x\right)\right)\end{array}$

The graph for the above function is shown in Figure 1

  

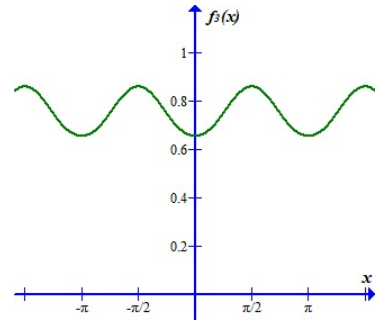
Figure 1

Then, the critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ .

The point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$

The critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ and point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$ .

From the above calculations it is observed that as more and more cosines are piled up the graph of the function is flat and stable at one value.

(d)

To determine

To find: The derivatives of the given function, the critical points, and the point of infection. Also, determine the situation when more and more cosines are piled up and explain it in terms of the updating function $x_{t+1}=\cos \left(x_t\right)$ .

Answer to Problem 43E

The derivate is $-\sin \left(\cos \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\right)\sin \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\sin \left(\cos \left(\cos \left(x\right)\right)\right)\left(\sin \left(\cos \left(x\right)\right)\sin \left(x\right)\right)$ , critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ and point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$ .As the pile increases the graph is flatten and the value is stable.

Explanation of Solution

Given:

The expression is $\cos \left(\cos \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\right)$ .

Calculation:

The critical point is the point on the curve where the slope of the tangent is zero and the tangent is parallel to the x axis.

The point of inflexion is the point on the curve at which the sign of the curvature changes.

Consider the given expression is,

  $\cos \left(\cos \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\right)$

Differentiate the above equation with respect to $x$ as,

  $\begin{array}{c}{f}^{\prime }\left(x\right)=\frac{d}{dx}\left(\cos \left(\cos \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\right)\right)\\ =-\sin \left(\cos \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\right)\frac{d}{dx}\left(\left(\cos \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\right)\right)\\ =-\sin \left(\cos \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\right)\sin \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\frac{d}{dx}\left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\frac{d}{dx}\left(\cos \left(\cos \left(x\right)\right)\right)\\ =-\sin \left(\cos \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\right)\sin \left(\cos \left(\cos \left(\cos \left(x\right)\right)\right)\right)\sin \left(\cos \left(\cos \left(x\right)\right)\right)\left(\sin \left(\cos \left(x\right)\right)\sin \left(x\right)\right)\end{array}$

The graph for the above function is shown in Figure 1

  

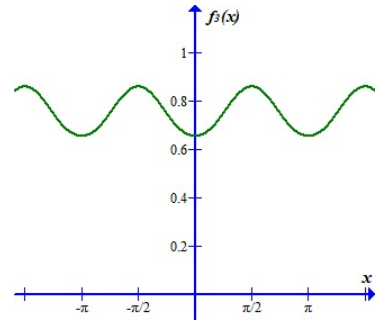
Figure 1

Then, the critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ .

The point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$

The critical points are $x=-\pi ,\frac{-\pi }{2},0,\frac{\pi }{2},\pi$ and point of inflexion is $x=-\frac{3\pi }{4},-\frac{\pi }{4},\frac{\pi }{4}.\frac{3\pi }{4}\mathrm{..}$ .

From the above calculations it is observed that as more and more cosines are piled up the graph of the function is flat and stable at one value.