Chapter 2, Problem 2.36P

To determine

Check the allowed energies of the centered infinite square well are consistent with equation 2.30, and confirm that the wave function can be obtained from the equation 2.31, and sketch the first three solution.

Answer to Problem 2.36P

The allowed energies of the centered infinite square well are consistent with equation 2.30, and the wave function can be obtained from the equation 2.31. The sketch the first three solution is shown below.

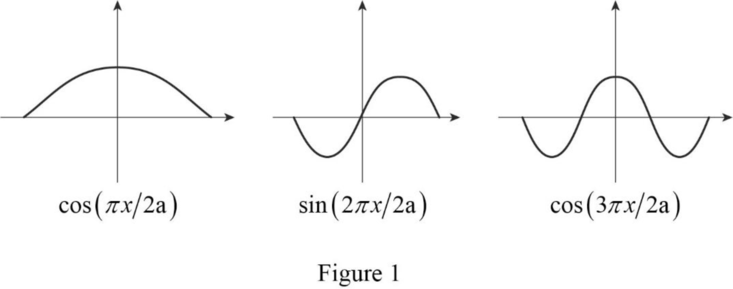
Explanation of Solution

Write the solution of classical simple harmonic oscillator.

    $\psi \left(x\right)=A\sin kx+B\cos kx$        (I)

The time boundary conditions are $\psi \left(a\right)=\psi \left(-a\right)=0$.

Apply this condition in equation1.

    $A\sin ka+B\cos ka=0$        (II)

    $-A\sin ka+B\cos ka=0$        (III)

Add equation (II) and (III), and solve.

    $\begin{array}{c}B\cos ka=0\\ ka=\left(j-\frac{1}{2}\right)\pi \\ \text{or }\\ B=0\end{array}$        (IV)

Where j = 1,2,3,…

Subtract equation (II) and (III), and solve.

    $\begin{array}{c}A\sin ka=0\\ ka=j\pi \\ \text{or }\\ A=0\end{array}$        (V)

If $B=0$ , then $k=\frac{j\pi }{a}$.

Let $n=2j$ in this case, then $k=\frac{n\pi }{2a}$

Then the equation (I) becomes,

    $\psi \left(x\right)=A\sin \frac{n\pi }{2a}x$        (VI)

Normalise the function in the equation (VI).

    $\begin{array}{c}{A}^2{\displaystyle \underset{-a}{\overset{a}{\int }}{\sin }^2\frac{n\pi }{2a}x}dx=1\\ A^{2}a=1\\ A=\frac{1}{\sqrt{a}}\end{array}$        (VII)

If $A=0$ , then $k=\left(j-\frac{1}{2}\right)\frac{\pi }{a}$.

Let $n=2j-1$ in this case, then $k=\frac{n\pi }{2a}$

Then the equation (I) becomes,

    $\psi \left(x\right)=B\cos \frac{n\pi }{2a}x$        (VIII)

Normalise the function in the equation (VIII).

    $\begin{array}{c}{A}^2{\displaystyle \underset{-a}{\overset{a}{\int }}{\cos }^2\frac{n\pi }{2a}x}dx=1\\ B^{2}a=1\\ B=\frac{1}{\sqrt{a}}\end{array}$        (IX)

In these both cases, the energy will get as follows.

    $\begin{array}{c}E=\frac{{\hslash }^2{k}^2}{2m}\\ =\frac{n^2{\pi }^2{\hslash }^2}{2m\left(2a\right)}\end{array}$        (X)

This is in agreement with equation 2.30 for a well of width 2a.

Use $\frac{x+a}{2}$ for $x$ in the wave function, then it takes equation 2.31 as follows.

    $\begin{array}{c}\sqrt{\frac{2}{a}}\sin \left(\frac{n\pi }{a}\frac{x+a}{2}\right)=\sqrt{\frac{2}{a}}\sin \left(\frac{n\pi x}{2a}+\frac{n\pi }{2}\right)\\ =\{\begin{array}{l}{\left(-1\right)}^{\frac{n}{2}}\sqrt{\frac{2}{a}}\sin \frac{n\pi x}{2a}\left(n\text{even}\right)\\ {\left(-1\right)}^{\frac{n-1}{2}}\sqrt{\frac{2}{a}}\cos \frac{n\pi x}{2a}\left(n\text{odd}\right)\end{array}\end{array}$        (XI)

So graph for the above function for first three solution is shown below.

It is same as Figure 2.2, except that some are upside down.

Conclusion:

Therefore, the allowed energies of the centered infinite square well are consistent with equation 2.30, and the wave function can be obtained from the equation 2.31. The sketch the first three solution is shown in Figure 1.