Chapter 2, Problem 2.33P

To determine

The form of the heat diffusion equation for each case, and to write the equation for the initial condition and the boundary conditions that are applied at $x=0$ and $x=L$ .

The equation for case (a) are, the heat equation is $\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=0$ , the initial condition for temperature distribution is $T\left(x,0\right)=T_i$ , the boundary condition equation for $x=0$ is ${\frac{\partial T}{\partial x}|}_{x=0}=0$ and at $x=L$ is $T_s=T\left(L,t\right)$ . The equation for case (b) is, the heat equation is $\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=0$ , the initial condition for temperature distribution is $T\left(x,0\right)=T_i$ , the boundary condition equation for $x=0$ is ${\frac{\partial T}{\partial x}|}_{x=0}=T_i$ and at $x=L$ is $-k{\frac{\partial T}{\partial x}|}_{x=L}=-h\left[T\left(L,t\right)-T_{\infty }\right]$ . The equations for case (c) are, the heat equation is $\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=\frac{1}{\alpha }\frac{\partial T}{\partial t}$ , the initial condition for temperature distribution is $T\left(x,0\right)=T_i$ , the boundary condition equation for $x=0$ is ${\frac{\partial T}{\partial x}|}_{x=0}=0$ and at $x=L$ is $T_s=T\left(L,t\right)$ .The equations for case (d) are, the heat equation is $\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=\frac{1}{\alpha }\frac{\partial T}{\partial t}$ , the initial condition for temperature distribution is $T\left(x,0\right)=T_i$ , the boundary condition equation for $x=0$ is ${\frac{\partial T}{\partial x}|}_{x=0}=0$ and at $x=L$ is $-k{\frac{\partial T}{\partial x}|}_{x=L}=-h\left[T\left(L,t\right)-T_{\infty }\right]$ .

Given:

The given diagram is shown in Figure 1.

  

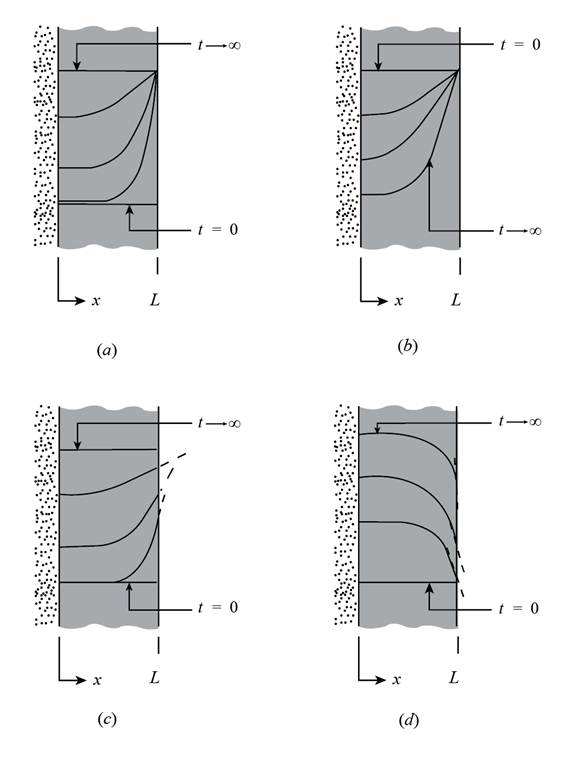
Figure 1

Formula Used:

The expression for the governing differential equation for the one-dimensional heat transfer without any heat generation is given by,

  $\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=\frac{1}{\alpha }\frac{\partial T}{\partial t}$

Here, $T$ is the temperature, $\dot{q}$ is the heat flux, $k$ is the thermal conductivity and $\alpha$ is the thermal diffusivity.

The expression for the slope of the temperature distribution is given by,

  ${\frac{\partial T}{\partial x}|}_{x=0}=0$

Calculation:

Case (a):

The temperature distribution at steady-state conditions is constant due to which the thermal energy generation does not take place. Therefore the dimensional heat conduction equation for case (a) is calculated as,

  $\begin{array}{l}\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=\frac{1}{\alpha }\frac{\partial T}{\partial t}\\ \frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=0\end{array}$

The initial condition of temperature for the plain wall is evaluated as,

  $T\left(x,0\right)=T_i$

Here, $T_i$ is the initial temperature.

The boundary condition equation for the temperature distribution at condition of $x=0$ is given by,

  ${\frac{\partial T}{\partial x}|}_{x=0}=0$

Here, $\frac{\partial T}{\partial x}$ is the temperature gradient.

The temperature remains the same at a time when step is greater than zero, thus the temperature at the surface remains constant. Therefore the boundary condition equation of temperature distribution at condition of $x=L$ is given by,

  $T_s=T\left(L,t\right)$

Here, $T_s$ is the surface temperature.

Case (b):

The temperature distribution at the steady-state condition is constant due to which the thermal energy generation does not take place, therefore the one-dimensional heat conduction equation is given by,

  $\begin{array}{l}\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=\frac{1}{\alpha }\frac{\partial T}{\partial t}\\ \frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=0\end{array}$

The initial condition of temperature distribution in a plane wall at $x=0$ and $x=L$ is given by,

  $T\left(x,0\right)=T_i$

The initial temperature is the same throughout the solid plane, therefore, the boundary condition equation of temperature at condition of $x=0$ is given by,

  ${\frac{\partial T}{\partial x}|}_{x=0}=T_i$

The temperature gradient at the surface decreases with the time step and the thermal energy generation leads to further fall in temperature. Thus the boundary condition equation of temperature distribution at condition of $x=L$ is given by,

  $-k{\frac{\partial T}{\partial x}|}_{x=L}=-h\left[T\left(L,t\right)-T_{\infty }\right]$

Case (c)

The temperature distribution at steady-state conditions is parabolic due to which the thermal energy generation takes place since the temperature increase with time due to large temperature the thermal energy becomes positive. Therefore, the one-dimensional heat conduction equation for the Cartesian coordinate in $x$ direction is given by,

  $\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=\frac{1}{\alpha }\frac{\partial T}{\partial t}$

The initial temperature is uniformly distributed across the solid and the initial condition temperature distribution for $x=0$ and $x=L$ is given by,

  $T\left(x,0\right)=T_i$

The boundary condition equation of temperature distribution at condition of $x=0$ is given by,

  ${\frac{\partial T}{\partial x}|}_{x=0}=0$

The boundary condition equation of temperature distribution at condition of $x=L$ is that the surface temperature remains constant and is given by,

  $T\left(L,t\right)=T_s$

Case (d)

The temperature distribution at steady-state conditions is parabolic due to which the thermal energy generation takes place. Therefore the one-dimensional heat conduction equation for the Cartesian coordinate in $x$ direction is given by,

  $\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=\frac{1}{\alpha }\frac{\partial T}{\partial t}$

The initial temperature is uniformly distributed across the solid and the initial condition temperature distribution for $x=0$ and $x=L$ is given by,

  $T\left(x,0\right)=T_i$

The boundary condition equation of temperature distribution at condition of $x=0$ is given by,

  ${\frac{\partial T}{\partial x}|}_{x=0}=0$

The temperature gradient at the surface decreases with the time step and the thermal energy generation leads to further fall in temperature. Thus the boundary condition equation of temperature distribution at condition of $x=L$ is given by,

  $-k{\frac{\partial T}{\partial x}|}_{x=L}=-h\left[T\left(L,t\right)-T_{\infty }\right]$

Conclusion:

Therefore, the equation for case (a) is, the heat equation is $\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=0$ , the initial condition for temperature distribution is $T\left(x,0\right)=T_i$ , the boundary condition equation for $x=0$ is ${\frac{\partial T}{\partial x}|}_{x=0}=0$ and at $x=L$ is $T_s=T\left(L,t\right)$ . The equation for case (b) are, the heat equation is $\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=0$ , the initial condition for temperature distribution is $T\left(x,0\right)=T_i$ , the boundary condition equation for $x=0$ is ${\frac{\partial T}{\partial x}|}_{x=0}=T_i$ and at $x=L$ is $-k{\frac{\partial T}{\partial x}|}_{x=L}=-h\left[T\left(L,t\right)-T_{\infty }\right]$ . The equations for case (c) are, the heat equation is $\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=\frac{1}{\alpha }\frac{\partial T}{\partial t}$ , the initial condition for temperature distribution is $T\left(x,0\right)=T_i$ , the boundary condition equation for $x=0$ is ${\frac{\partial T}{\partial x}|}_{x=0}=0$ and at $x=L$ is $T_s=T\left(L,t\right)$ .The equations for case (d) are, the heat equation is $\frac{{\partial }^{2}T}{\partial x^2}+\frac{\dot{q}}{k}=\frac{1}{\alpha }\frac{\partial T}{\partial t}$ , the initial condition for temperature distribution is $T\left(x,0\right)=T_i$ , the boundary condition equation for $x=0$ is ${\frac{\partial T}{\partial x}|}_{x=0}=0$ and at $x=L$ is $-k{\frac{\partial T}{\partial x}|}_{x=L}=-h\left[T\left(L,t\right)-T_{\infty }\right]$ .