
Two rigid bars are connected to each other by two linearly elastic springs. Before loads are applied, the lengths or the springs are such, that the bars are parallel and the springs are without stress.
(a) Derive a formula for the displacement E4at point 4 when the load P is applied at joint 3 and moment PL is applied at joint 1. as shown in the figure part a. (Assume that the bars rotate through very small angles under the action of load P.)
(b) Repeat part (a) if a rotational spring, kr= kL2, is now added at joint 6. What is the ratio of the deflection d4 in the figure part a to that in the figure part b ?
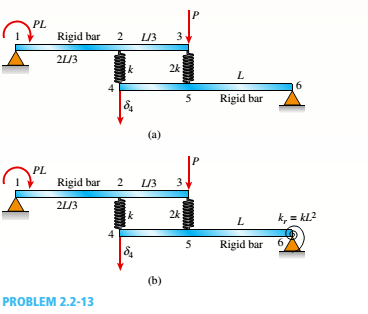
(a)
The formula for the displacement at point
Answer to Problem 2.2.13P
The formula for the displacement at point
Explanation of Solution
Given information:
The load applied at point
The moment art point
The following figure shows the free body diagram of the bar:
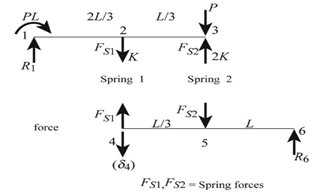
Figure-(1)
Write the expression for the moment equilibrium about support
Here, the reaction at forces in spring
Write the expression for the moment equilibrium about support
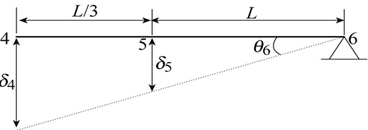
Figure-(2)
Write the expression for deflection
Here, the deflection at point
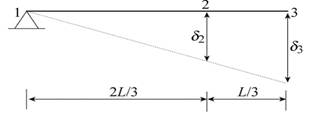
Figure-(3)
Write the expression for deflection
Here, the deflection at point
Write the expression for the spring stiffness.
Here, the spring constant is
Write the expression for the elongation of spring
Write the expression for the elongation of spring
Calculation:
Substitute
Substitute
Substitute
Substitute
Conclusion:
The formula for the displacement at point
(b)
The ratio of deflection at point
Answer to Problem 2.2.13P
The ratio of deflection at point 4 is
Explanation of Solution
Given Information:
The spring constant for rotational spring is
The following figure shows the free body diagram of the bar having rotational spring:
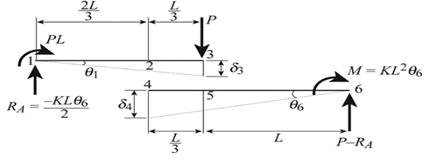
Figure-(4)
Write the expression for the moment equilibrium about support
Here, angle of deflection of beam is
Write the expression for the net elongation of spring
Write the expression for the displacement at point
Write the expression for the displacement at point
Write the expression for the net elongation of spring
Write the expression for displacement at point 3.
Write the expression for displacement at point 5.
The figure below shows the free body diagram of the bar.
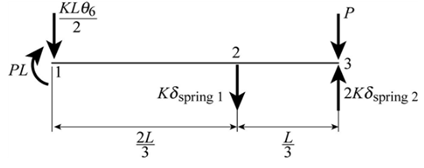
Figure-(5)
Write the expression for the vertical force equilibrium.
Write the expression for the moment equilibrium about point 2.
Write the expression for the angle of deflection of the beam
The following figure shows the forces acting on point
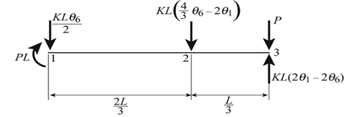
Figure 6
Write the expression for the ratio of deflection of part
Calculation:
Substitute
Substitute
Substitute
Substitute
Conclusion:
The ratio of deflection at point
Want to see more full solutions like this?
Chapter 2 Solutions
Mechanics of Materials (MindTap Course List)
- This is an exam review question. The answer is Pmin = 622.9 lb but whyarrow_forwardPlease do not use any AI tools to solve this question. I need a fully manual, step-by-step solution with clear explanations, as if it were done by a human tutor. No AI-generated responses, please.arrow_forwardPlease do not use any AI tools to solve this question. I need a fully manual, step-by-step solution with clear explanations, as if it were done by a human tutor. No AI-generated responses, please.arrow_forward
- Please do not use any AI tools to solve this question. I need a fully manual, step-by-step solution with clear explanations, as if it were done by a human tutor. No AI-generated responses, please.arrow_forwardThis is an old practice exam. Fce = 110lb and FBCD = 62 lb but whyarrow_forwardQuiz/An eccentrically loaded bracket is welded to the support as shown in Figure below. The load is static. The weld size for weld w1 is h1 = 4mm, for w2 h2 = 6mm, and for w3 is h3 =6.5 mm. Determine the safety factor (S.f) for the welds. F=29 kN. Use an AWS Electrode type (E100xx). 163 mm S 133 mm 140 mm Please solve the question above I solved the question but I'm sure the answer is wrong the link : https://drive.google.com/file/d/1w5UD2EPDiaKSx3W33aj Rv0olChuXtrQx/view?usp=sharingarrow_forward
- Q2: (15 Marks) A water-LiBr vapor absorption system incorporates a heat exchanger as shown in the figure. The temperatures of the evaporator, the absorber, the condenser, and the generator are 10°C, 25°C, 40°C, and 100°C respectively. The strong liquid leaving the pump is heated to 50°C in the heat exchanger. The refrigerant flow rate through the condenser is 0.12 kg/s. Calculate (i) the heat rejected in the absorber, and (ii) the COP of the cycle. Yo 8 XE-V lo 9 Pc 7 condenser 5 Qgen PG 100 Qabs Pe evaporator PRV 6 PA 10 3 generator heat exchanger 2 pump 185 absorberarrow_forwardQ5:(? Design the duct system of the figure below by using the balanced pressure method. The velocity in the duct attached to the AHU must not exceed 5m/s. The pressure loss for each diffuser is equal to 10Pa. 100CFM 100CFM 100CFM ☑ ☑ 40m AHU -16m- 8m- -12m- 57m 250CFM 40m -14m- 26m 36m ☑ 250CFMarrow_forwardA mass of ideal gas in a closed piston-cylinder system expands from 427 °C and 16 bar following the process law, pv1.36 = Constant (p times v to the power of 1.36 equals to a constant). For the gas, initial : final pressure ratio is 4:1 and the initial gas volume is 0.14 m³. The specific heat of the gas at constant pressure, Cp = 0.987 kJ/kg-K and the specific gas constant, R = 0.267 kJ/kg.K. Determine the change in total internal energy in the gas during the expansion. Enter your numerical answer in the answer box below in KILO JOULES (not in Joules) but do not enter the units. (There is no expected number of decimal points or significant figures).arrow_forward
- my ID# 016948724. Please solve this problem step by steparrow_forwardMy ID# 016948724 please find the forces for Fx=0: fy=0: fz=0: please help me to solve this problem step by steparrow_forwardMy ID# 016948724 please solve the proble step by step find the forces fx=o: fy=0; fz=0; and find shear moment and the bending moment diagran please draw the diagram for the shear and bending momentarrow_forward
 Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
