Chapter 11.7, Problem 11IP

To determine

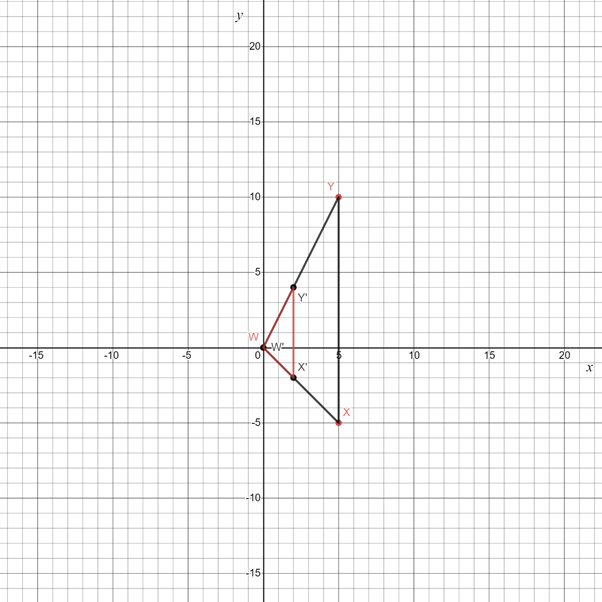
To find: Vertices of each figure after a dilation.

Answer to Problem 11IP

  $\begin{array}{l}W\text{'}\left(0,0\right)\\ X\text{'}\left(2,-2\right)\\ Y\text{'}\left(2,4\right)\end{array}$

Explanation of Solution

Given information:

  $\begin{array}{l}k=\frac{2}{5}\\ W=\left(0,0\right)\\ X=\left(5,-5\right)\\ Y=\left(5,10\right)\end{array}$

The dilation is $\left(x,y\right)\to \left(\frac{2}{5}x,\frac{2}{5}y\right)$ .

Multiply the coordinates of each vertex by $\frac{2}{5}$ .

  $\begin{array}{l}W\left(0,0\right)\to W\text{'}\left(\frac{2}{5}\left(0\right),\frac{2}{5}\left(0\right)\right)\\ \Rightarrow W\left(0,0\right)\to W\text{'}\left(0,0\right)\end{array}$

  $\begin{array}{l}X\left(5,-5\right)\to X\text{'}\left(\frac{2}{5}\left(5\right),\frac{2}{5}\left(-5\right)\right)\\ \Rightarrow X\left(5,-5\right)\to X\text{'}\left(2,-2\right)\end{array}$

  $\begin{array}{l}Y\left(5,10\right)\to Y\text{'}\left(\frac{2}{5}\left(5\right),\frac{2}{5}\left(10\right)\right)\\ \Rightarrow Y\left(5,10\right)\to Y\text{'}\left(2,4\right)\end{array}$

Therefore,

Coordinates after dilation are

  $\begin{array}{l}W\text{'}\left(0,0\right)\\ X\text{'}\left(2,-2\right)\\ Y\text{'}\left(2,4\right)\end{array}$

Graph of image is