Chapter 9.12, Problem 180RP

(a)

To determine

Draw the $P-v$ and $T-s$ diagrams for the given cycle.

Answer to Problem 180RP

The $P-v$ and $T-s$ diagrams for the given cycle are shown as in Figure (1).

Explanation of Solution

Draw the $P-v$ and $T-s$ diagram for the given cycle.

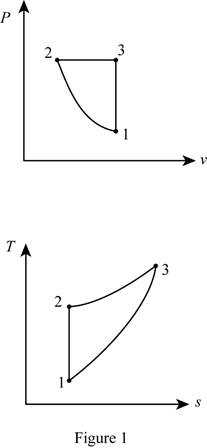
Thus, the $P-v$ and $T-s$ diagrams for the given cycle are shown as in Figure (1)

(b)

To determine

The expression for the back work ratio as a function of k and r.

Answer to Problem 180RP

The expression for the back work ratio as a function of k and r is $\underset{\_}{\frac{1}{k-1}\frac{1}{r^{k-1}}\frac{r^{k-1}-1}{r-1}}$.

Explanation of Solution

Find the work of compression using the first law for process 1-2.

$\begin{array}{l}{q}_{1-2}-w_{1-2}=\Delta u_{1-2}\\ w_{1-2}=-\Delta u_{1-2}\\ w_{1-2}=-c_v\left(T_2-T_1\right)\\ w_{\text{comp}}=-w_{1-2}\end{array}$

$w_{\text{comp}}=-c_v\left(T_2-T_1\right)$ (I)

Here, heat interaction during the process 1-2 is $q_{1-2}$, work interaction for process 1-2 is $w_{1-2}$, change in specific internal energy for process 1-2 is $\Delta u_{1-2}$, constant volume specific heat is $c_v$, temperature at state 1 and 2 is $T_1,\text{and}{T}_2$ respectively.

Write the expression of expansion work.

$\begin{array}{c}{w}_{\exp }=w_{2-3}\\ ={\displaystyle \underset{2}{\overset{3}{\int }}Pdv}\\ =P\left(v_3-v_2\right)\\ =R\left(T_3-T_2\right)\end{array}$ (II)

Here, gas constant is R, specific volume at state 2 and 3 is $v_2\text{and}{v}_3$, and work interaction for process 2-3 is $w_{2-3}$.

Write the expression of back work ratio using the equations (I) and (II).

$\begin{array}{c}\frac{w_{\text{comp}}}{w_{\exp }}=\frac{c_v\left(T_3-T_1\right)}{R\left(T_3-T_2\right)}\\ =\frac{c_v}{R}\frac{T_1}{T_2}\frac{\left(T_3/T_1\right)-1}{\left(T_3/T_2\right)-1}\end{array}$ (III)

Here, temperature at state 1, 2, and 3 are $T_1,T_2,\text{and}{T}_3$ respectively.

Conclusion:

Process 1-2: Isentropic

Calculate the ratio of $T_1/T_2$ and $P_2/P_1$.

$\begin{array}{c}\frac{T_1}{T_2}={\left(\frac{v_2}{v_1}\right)}^{k-1}\\ =\frac{1}{r^{k-1}}\end{array}$

$\begin{array}{c}\frac{P_2}{P_1}={\left(\frac{v_1}{v_2}\right)}^k\\ =r^k\end{array}$

Here, pressure at state 1 and 2 is $P_1,P_2$, volume at states 1 and 2 is $v_1,v_2$, compression ratio is r, and specific heat ratio is k.

Process 2-3: Constant pressure

Calculate the expression for $T_3/T_2$.

$\begin{array}{l}\frac{P_3{v}_3}{T_3}=\frac{P_2{v}_2}{T_2}\\ \frac{T_3}{T_2}=\frac{v_3}{v_2}=\frac{v_1}{v_2}=r\\ \frac{T_3}{T_2}=\frac{v_1}{v_2}\\ \frac{T_3}{T_2}=r\end{array}$

Here, volume at state 1 and 2 is $v_1\text{}\text{and}{v}_2$.

Process 3-1: Constant volume

Calculate the expression for $T_3/T_1$.

$\begin{array}{l}\frac{P_3{v}_3}{T_3}=\frac{P_1{v}_1}{T_1}\\ \frac{T_3}{T_1}=\frac{P_3}{P_1}\\ \frac{T_3}{T_1}=\frac{P_2}{P_1}\\ \frac{T_3}{T_1}=r^k\end{array}$

Substitute $r^k$ for $\frac{T_3}{T_1}$ and $r$ for $\frac{T_3}{T_2}$, $\frac{1}{r^{k-1}}$ for $\frac{T_1}{T_2}$, and $\frac{R}{k-1}$ for $c_v$ in Equation (III).

$\begin{array}{c}\frac{w_{\text{comp}}}{w_{\exp }}=\frac{\frac{R}{k-1}}{R}\left(\frac{1}{r^{k-1}}\right)\frac{\left(r^k\right)-1}{\left(r\right)-1}\\ =\frac{1}{k-1}\left(\frac{1}{r^{k-1}}\right)\frac{\left(r^k\right)-1}{\left(r\right)-1}\end{array}$

Thus, the expression for the back work ratio as a function of k and r is $\underset{\_}{\frac{1}{k-1}\frac{1}{r^{k-1}}\frac{r^k-1}{r-1}}$.

(c)

To determine

The expression for the cycle thermal efficiency as a function of k and r.

Answer to Problem 180RP

The expression for the cycle thermal efficiency as a function of k and r is $1-\frac{1}{k}\frac{1}{r^{k-1}}\frac{r^k-1}{r-1}$.

Explanation of Solution

Express out the heat addition and heat rejection in the process using first law to the closed system for processes 2-3 and 3-1.

$q_{\text{in}}=c_p\left(T_3-T_2\right)$

$q_{\text{out}}=c_v\left(T_3-T_1\right)$

Here, constant pressure specific heat is $c_p$.

Express the cycle thermal efficiency.

${\eta }_{\text{th}}=1-\frac{q_{\text{out}}}{q_{\text{in}}}$ (IV)

Conclusion:

Substitute $c_p\left(T_3-T_2\right)$ for $q_{\text{in}}$ and $c_v\left(T_3-T_1\right)$ for $q_{\text{out}}$ in Equation (IV).

$\begin{array}{c}{\eta }_{\text{th}}=1-\frac{c_v\left(T_3-T_1\right)}{c_p\left(T_3-T_2\right)}\\ =1-\frac{1}{k}\frac{T_1\left(T_3/T_1-1\right)}{T_2\left(T_3/T_2-1\right)}\end{array}$

Substitute $r^{k-1}$ for $\frac{T_3}{T_1}$, $r$ for $\frac{T_3}{T_2}$, and $\frac{1}{r^{k-1}}$ for $\frac{T_1}{T_2}$.

$\begin{array}{c}{\eta }_{\text{th}}=1-\frac{c_v\left(T_3-T_1\right)}{c_p\left(T_3-T_2\right)}\\ =1-\frac{1}{k}\frac{1}{r^{k-1}}\frac{\left(r^k-1\right)}{\left(r-1\right)}\end{array}$

Thus, the expression for the cycle thermal efficiency as a function of k and r is $1-\frac{1}{k}\frac{1}{r^{k-1}}\frac{r^k-1}{r-1}$.

(d)

To determine

The value of the back work ratio and thermal efficiency as r goes to unity.

Answer to Problem 180RP

The value of the back work ratio and thermal efficiency as r goes to unity is $\underset{\_}{1}$ and $\underset{\_}{0}$.

Explanation of Solution

Recall the expression of back work ratio and apply the limits as r goes to infinity.

$\begin{array}{l}\frac{w_{\text{comp}}}{w_{\exp }}=\frac{1}{k-1}\left(\frac{1}{r^{k-1}}\right)\frac{\left(r^{k-1}\right)-1}{\left(r\right)-1}\\ \underset{r\to 1}{\lim }\frac{w_{\text{comp}}}{w_{\exp }}=\frac{1}{k-1}\left\{\underset{r\to 1}{\lim }\frac{1}{r^{k-1}}\frac{\left(r^{k-1}\right)-1}{\left(r\right)-1}\right\}\\ \underset{r\to 1}{\lim }\frac{w_{\text{comp}}}{w_{\exp }}=\frac{1}{k-1}\left\{\underset{r\to 1}{\lim }\frac{\left(r^{k-1}\right)-1}{\left(r^k\right)-r^{k-1}}\right\}\\ \underset{r\to 1}{\lim }\frac{w_{\text{comp}}}{w_{\exp }}=\frac{1}{k-1}\left\{\underset{r\to 1}{\lim }\frac{\left(k-1\right)r^{k-2}}{k{r}^{k-1}-\left(k-1\right)r^{k-2}}\right\}\end{array}$

$\begin{array}{c}\underset{r\to 1}{\lim }\frac{w_{\text{comp}}}{w_{\exp }}=\frac{1}{k-1}\left\{\frac{k-1}{k-k+1}\right\}\\ =\frac{1}{k-1}\left\{\frac{k-1}{1}\right\}\\ =1\end{array}$

Thus, the value of the back work ratio as r goes to unity is $\underset{\_}{1}$.

Recall the expression of cycle thermal efficiency and apply the limits as r goes to infinity.

$\begin{array}{l}{\eta }_{\text{th}}=1-\frac{1}{k}\frac{1}{r^{k-1}}\frac{r^k-1}{r-1}\\ \underset{r\to 1}{\lim }{\eta }_{\text{th}}=1-\frac{1}{k}\left\{\underset{r\to 1}{\lim }\frac{1}{r^{k-1}}\frac{r^{k-1}}{r-1}\right\}\\ \underset{r\to 1}{\lim }{\eta }_{\text{th}}=1-\frac{1}{k}\left\{\underset{r\to 1}{\lim }\frac{r^{k-1}}{r^k-r^{k-1}}\right\}\\ \underset{r\to 1}{\lim }{\eta }_{\text{th}}=1-\frac{1}{k}\left\{\underset{r\to 1}{\lim }\frac{k{r}^{k-1}}{k{r}^{k-1}-\left(k-1\right)r^{k-2}}\right\}\end{array}$

$\begin{array}{c}\underset{r\to 1}{\lim }{\eta }_{\text{th}}=1-\frac{1}{k}\left\{\frac{k}{k-k+1}\right\}\\ =1-\frac{1}{k}\left\{\frac{k}{1}\right\}\\ =0\end{array}$

Thus, the value of the thermal efficiency as r goes to unity is $\underset{\_}{0}$.

From the results of cycle thermal efficiency and back work ratio values of 0 and 1, it shows that no expansion and net work can be done whether you add heat to the system when there is no compression $\left(r=1\right)$.